This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
2 solutions
The problem can be solved using analytical geometry as well.
Set your coordinate system with a center at point B, x-axis going to the right, past point C. This gives you the points A = (-3, 0), C = (4, 0), and D = (0, -2). Center of the circle is equidistant from all of these points. To be equidistant from both A and C it has to have an x-coordinate equal to 1/2. To be equidistant form D and C, it has to be on a perpendicular bisector of CD. Line through CD has a slope 1/2. The midpoint between C and D is (2, -1). Line through (2, -1) with a slope -2 is given by
y − ( − 1 ) = − 2 ( x − 2 )
Substituting into it x = 1/2 we get the y-coordinate of the center of the circle as y = 2. Distance between the center, (1/2, 2) and (0, -2) is
( 2 1 ) 2 + 4 2 = 2 6 5
Recognizing that AB * BC = DB * BE or 3 * 4 =2 * BE gives BE =6
Perpendicular bisectors of the two chords define center O.
Perpendicular from O to AC connects to AC 3.5 from A.
O is 4 - 2 = 2 away from AC.
So we have a right triangle with sides 3.5 and 2 and hypotenuse R.
R^2 = 2^2 + (7 / 2)^2 = 4 + 49 / 4 = 16 / 4 + 49 / 4 = 65 / 4
R = 2 6 5 so 65 + 2 = 67
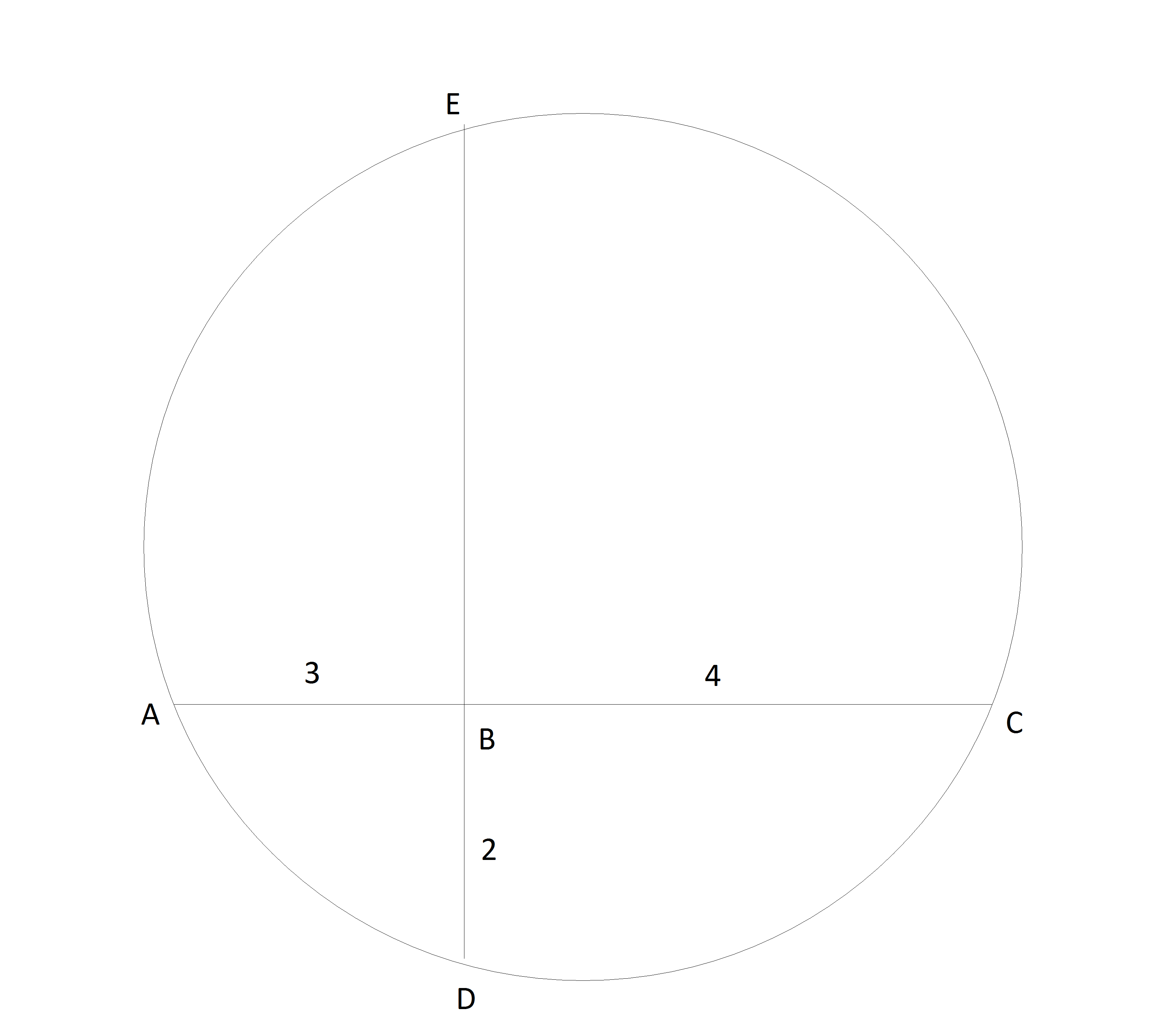
Triangles ABE and DBC are similar, so the distance BE is 6. Diameter KL parallel to the line AC bisects cord DE giving FE = FD = 4. Similarly vertical diameter bisects AC and this results in the width of the rectangle BFGH being 1/2. The triangles KFE and DFL are also similar, therefore
4 K F = F L 4
4 R − 2 1 = R + 2 1 4
Solving for R we get
R = 2 6 5