What's the Area?
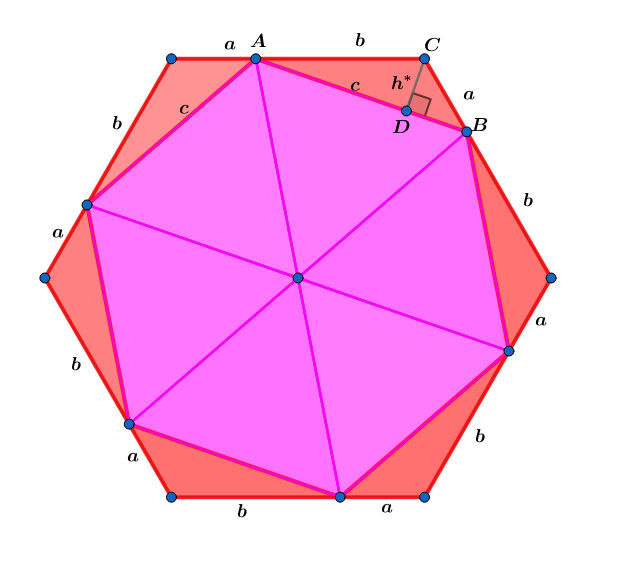
Let the diagram above be extended to a regular -gon and , where , be the area of one of the red triangles.
If , find .
The area of what simple closed curve does converge to?
The answer is 3.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
Let m ( ∠ A C B ) = n ( n − 2 ) 1 8 0 be one of the interior angles of the larger n − g o n .
sin ( n ( n − 2 ) 1 8 0 ) = sin ( 1 8 0 − n 3 6 0 ) = sin ( n 3 6 0 ) = sin ( n 2 π ) .
Using the law of sines ⟹ sin ( n 2 π ) c = sin ( α ) a ⟹ sin ( α ) = c a sin ( n 2 π ) ⟹ h ∗ = c a b sin ( n 2 π ) ⟹ ∑ j = 1 n A j = n 2 1 ( c ) ( c a b sin ( n 2 π ) ) = 2 n a b sin ( n 2 π ) .
Using the inequality cos ( x ) < x sin ( x ) < 1 ⟹ π cos ( n π ) < 2 n sin ( n 2 π ) < π ⟹ lim n → ∞ ∑ j = 1 n A j = π a b ⟹ ∑ j = 1 n A j converges to the area of an ellipse.
lim n → ∞ ∑ j = 1 n A j = π a b = π ( a 2 + 2 a b − 1 2 b 2 ) ⟹ a 2 + a b − 1 2 b 2 = 0 ⟹ ( a − 3 b ) ( a + 4 b ) = 0 since a , b > 0 ⟹ b a = 3 .