What Fraction Is It?
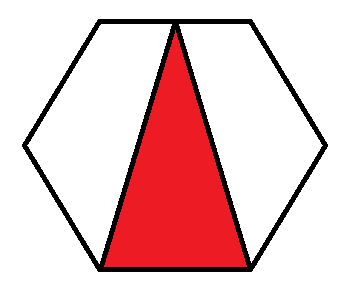 The area of the shaded triangle expressed as a fraction of the area of the regular hexagon is:
The area of the shaded triangle expressed as a fraction of the area of the regular hexagon is:
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
22 solutions
Please elaborate on your first statement.
Solving without touching pen or calc:
1)Look at the red triangle ,the height and base especially. 2)See a rectangle at the middle of the hexagon by the vertical sides of hexagon as two sides.Now if you remove the red triangle you will get two triangles with half the base,so if you add the area of two triangles you will get the same area as the red triangle.So the rectangle is two times the red triangle. 3)Now if you remove the rectangle ,remaining is two trangles at the left and right where each triangle can be further split into two with base/2 and height/2.Now we understood that after removing the rectangle,the remaining area can be filled with another red triangle cut into four pieces. 4)So totally 3 Red triangles are necessary to fill the hexagon,So the answer is 1/3.
:D,i was not having a calc or pen near by.
area of regular hexagon= 3/2 root(3) x^2
area of shaded region= root(3)/2* x^2 , as the height of shaded triangle is root(3)*x
so ratio= 1/3
area of triangle = 1/2 x base x altitude whereas area of regular hexagon = 1/2 x perimeter x apothem. Let us suppose base = b and apothem = a then area of triangle = 1/2 x b x 2a (altitude of triangle is twice that of apothem) = ab. And area of regular hexagon = 1/2 x 6b x a = 3ab. Comparing the two areas, we see area of hexagon is thrice the area of triangle OR area of triangle is 1/3 the area of regular hexagon.
i did it that way
You can redraw the hexagon into three equal rectangles. the shaded area divides two of those rectangles into halves. That is, the shaded area equal one of the three rectangles. Then, the shaded area is 1/3 the hexagon area.
Half the height (=half the area) is one of 6 "petals" making up hexagon. Hence 1/3
The hexagon is made up of 6 equilateral △ s. Let the height of each of these triangles be h and sides be s .
Area of the Hexagon = 2 6 h s = 3 h s
Area of the Red △ = 2 2 h s = h s
⟹ ar(Hexagon) ar ( △ ) = 3 h s h s = 3 1
Area of regular hexagon = (3√3)/2 x^2 . Distance b/w the parallel sides of regular hexagon = √3 x . Since, area of triangle = (base * height)/2 = (t*√3 x)/2 =(√3 x^2)/2 . So, (area of triangle)/(area of hexagon)= ((√3 x^2)/2)/((3√3)/2 x^2 ) = 1/3
The hexagon is a regular hexagon, so we can divide it into 6 pieces of a regular triangle.
Then, if I let the height of the triangle to h , and a length of a side to 2, then we get h = 3 .
Because the length is two, and the half-length of the side is one, then, in the regular triangle, we get 2 2 − 1 2 = 3 by Pythagorean theorem .
So, the height of the regular hexagon is 2 × 3 = 2 3 .
Then, the area of red shaded triangle is 2 2 3 × 2 = 2 3 .
And, the area of regular triangle is 2 3 × 2 × 6 = 6 3 .
So, 3 1 .
Slvd it in just seconds....in mind ...without any pen or calc.!!
We cn imagine the figure divided in 6 triangles of wich 2 triangles form the shaded area ......clearly area of each triangle=1/6of figure
nd tht of shaded region =1/3 of the figure
Let a rectangle be drawn joining each end of any 2 opposite sides of the hexagon and let its area be A. The red triangle's area is then A/2.... Let the area of the hexagon be H.. After drawing the rectangle we see that the area of the hexagon has been divided in 3 parts.....a rectangle, and 2 triangles, each of whose area is A/4. thus the whole area becomes H=A+A/4+A/4=3A/2 We need A/2. So we can now solve for it A/2=H/3 That means the triangle is 1 3rd of the hexagon. .....
You can break the hexagon down into 6 equilateral triangles with length "L" and height "H". The area of the hexagon becomes 6(1/2(L H)). The area of the red triangle becomes 1/2(L (2H)). These reduce to give a hexagon area of 3LH and a red triangle area of LH. Taking the ratio of the two yields LH/3LH=1/3.
Eyeball it or think of it can't be more parts than 3 so le duh
If you remove the red triangle and close the gap you get a pentagon. So subtract from the area of an hexagon with side 1 the area of a pentagon with side 1 (I used Google for that) to get the red area, then do the ratio and you get ~0.33 which is close to 1/3.
Putting 6 diagonals & counting all triangles formed.There are exactly a third of theme are shaded
If the length of one of the sides is 1, the area of the triangle is one because 1 * 2 * 1/2 = 1. The total area would be 6 (1 * 1 * 1/2) and then 1/3 is your answer :)
Call the area of the hexagon "H" and the area of the triangle "T". Then our answer should be T/H. Call the side length of the hexagon "b" and the length from the center of the hexagon to the point on one of the sides such that the length is perpendicular to the side "a". Then T is equal to 2ab/2 = ab. H is equal to 2 times the area of the trapezoid that is one half of the hexagon. The trapezoid's area, call it "T*", is (2a + a)b/2. Then H is 3ab. Then T/H is ab/3ab = 1/3.
I have to choose between 1l4 1l3 1l5 1l6, choosing 1l4 would mean that the area of hexagone =4times the area of the red triangle which is visually (by intuition) not possible and so is choosing 1l5 and 1l6.the only remaining possible answer is 1l3
A hexagon is a sum of 6 equilateral triangles. Rest is cake walk.
I sensed it. Forming a rectangle from the red triangle and two additional rectangles from the 2 white remaining spaces. Hence 1/3
you just estimate it #winning
If triangle had a height that was half as great then the area would clearly be 1/6 of the hexagon. The formula for calculating this area for this triangle is just 1/2 * base * height. Therefore if the the height were not to the centre (half as great) but to the other side as the question asks then if 0.5 * base * (height/2)=1/6, 0.5 * base * height=1/3 as it is a linear relationship. :)