What fraction of the diagram is black?
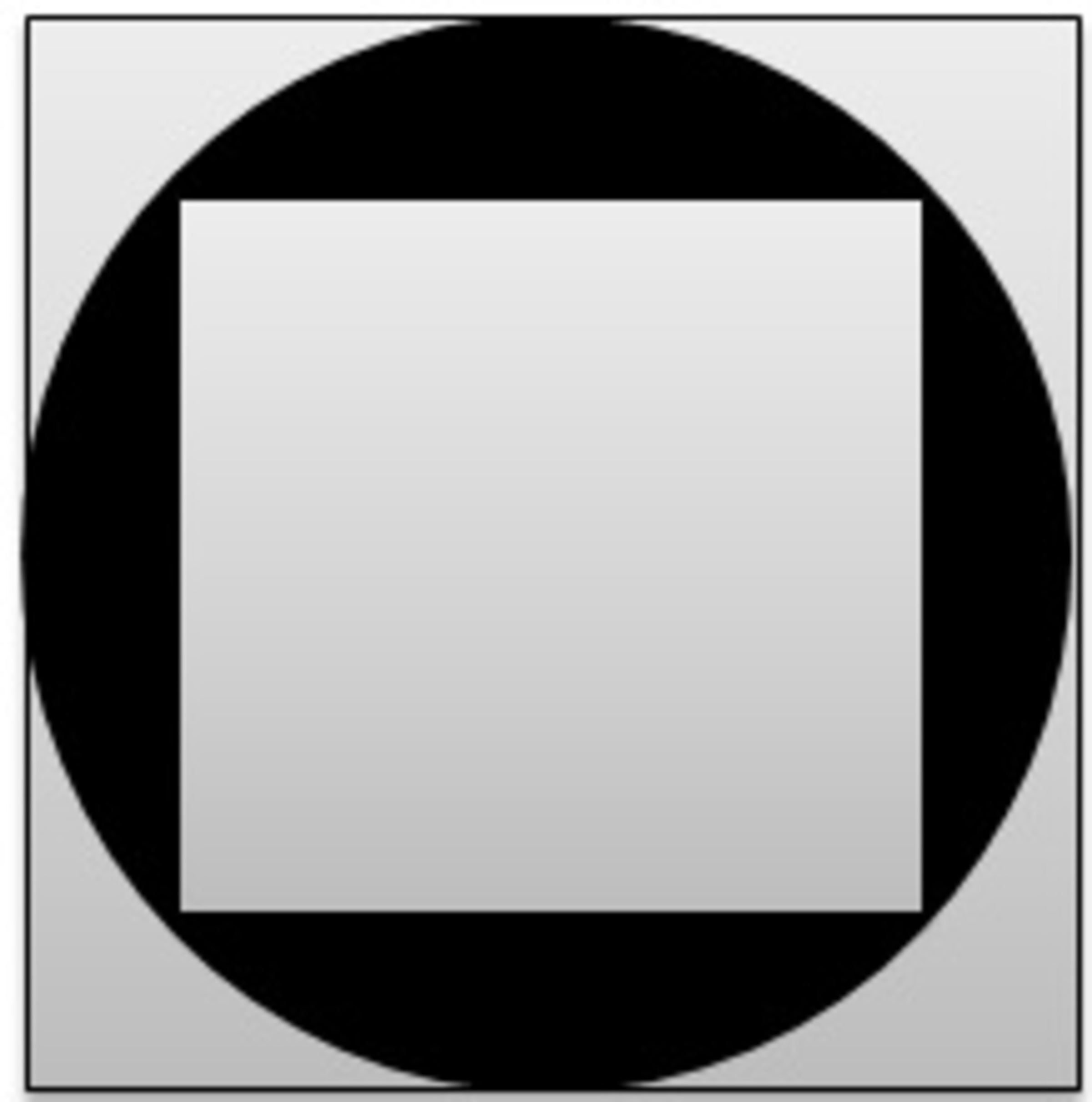
A grey square has a black circle inscribed and a grey square inscribed in the circle. What fraction of the diagram is black? (Round your answer off to 3 decimal places)
The answer is 0.285.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
Let the side of the big square be x units. Hence it's area = x 2 .
The radius of the circle = 2 x , because x is the diameter. Hence the area of the circle = π 4 x 2 .
We can observe that the diagonal of the smaller square is the diameter of the circle, therefore each side of the smaller square = 2 x 2 = 2 x 2 . Hence it's area = ( 2 x 2 ) 2 = 4 2 x 2 .
Therefore the area of the black region = ar(circle)-ar(small square) = π 4 x 2 − 4 2 x 2 = 2 x 2 [ 2 π − 1 ]
Hence the ratio of the black region to the whole square = x 2 2 x 2 [ 2 π − 1 ] = 4 π − 2 1 ≈ 0 . 2 9