What happens to water level?
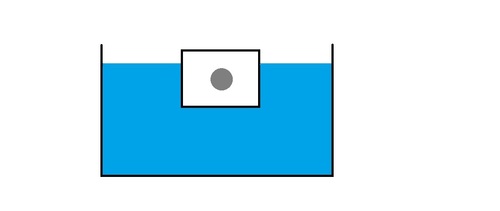 An ice cube is floating with a ball of lead inside it. When all the ice melts, what happens to the water level?
An ice cube is floating with a ball of lead inside it. When all the ice melts, what happens to the water level?
NOTE-
1) Take density of ice to be less than density of water.
2) Density of lead > Density of water.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
When the ice is floating,
d w a t e r v d i s p l a c e d g = ( M i c e + m l e a d ) g
Therefore, v d i s p l a c e d = d w a t e r ( M i c e + m l e a d )
When all the ice melts, it gets converted into water so volume displaced by it will be same as before. But, since the lead ball sinks in water, using Archimedes priciple, V d i s p l a c e d = d l e a d m l e a d
Now, the total volume displaced is
V d i s p l a c e d = d w a t e r M i c e + d l e a d m l e a d
Since, d l e a d > d w a t e r
The final volume displaced is less than initial volume displaced.
S o , w a t e r l e v e l f a l l s !