What Is the Area of the Sky Seen by a Fish?

Trajectory of the light beams and the shape of the area in which the fish sees the sky

We assume the fish’s eye as the point in which the rays converge.

The area will be circular with the radius r

The beams which are incident on the surface at the angle of 90°

Now we focus only on the beams that are refracted at the angle α (see „Picture of the beams“).

We derive the relation for the angle of refraction α from Snell’s law:

$\frac{\sin {90°}}{\sin \mathrm{α}}=\frac{n_{2}}{n_{1}}$

where $n_{1}$ is the refractive index of air and $n_{2}$ is the refractive index of water.

since $\sin {90°}=1$ , we can write:

$\frac{1}{\sin \mathrm{α}}=\frac{n_{2}}{n_{1}}$ Therefrom we express $\sin \mathrm{α}$ :

$\sin \mathrm{α}=\frac{n_{1}}{n_{2}},\tag{2}$

Due to the similarity of triangles we can adjust the picture as follows: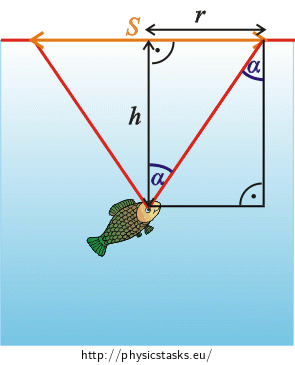

We can see from the picture that $\sin \mathrm{α}=\frac{r}{\sqrt{r^2+h^2}},\tag{3}$ where $\sqrt{r^2+h^2}$ is a hypotenuse of the triangle and we have determined its length from the Pythagorean theorem. Size of the desired area

1. $S=\pi · r^2,$ 2. $\sin \mathrm{α}=\frac{n_{1}}{n_{2}}$ 3. $\sin \mathrm{α}=\frac{r}{\sqrt{r^2+h^2}}$

If we multiply both sides of the equation (3) by $\sqrt{r^2+h^2}$ $r=\sqrt{r^2+h^2}·\sin \mathrm{α}$

According to (2)) we substitute sinα: $r= \sqrt{r^2+h^2} · \frac{n_{1}}{n_{2}}$

We raise both sides of the equation to the power of two and multiply the brackets: $r^2= \left(r^2+h^2 \right)· \left(\frac{n_{1}}{n_{2}}\right)^2=r^2\left(\frac{n_{1}}{n_{2}}\right)^2+h^2\left(\frac{n_{1}}{n_{2}}\right)^2$

Then we subtract $r^2\left (\frac{n_{1}}{n_{2}}\right )^2$ from both sides of the equation and factor out $r^2$ $r^2\left [1 - \left(\frac{n_{1}}{n_{2}}\right)^2\right]= h^2\left (\frac{n_{1}}{n_{2}}\right)^2$ We divide both sides of the equation by $\left[1 - (\frac{n_{1}}{n_{2}})^2\right]$ and extract the root:

$r= \frac {h\left(\frac{n_{1}}{n_{2}}\right)} {\sqrt{1 - \left(\frac{n_{1}}{n_{2}}\right)^2}}= \frac {h · n_{1}} {n_{2} \sqrt{1 - \left(\frac{n_{1}}{n_{2}}\right)^2}}$

We convert the expression inside the square root to the common denominator and adjust the fraction:

$r= \frac {h · n_{1}} {n_{2} \sqrt{\frac{n_{2}^2-n_{1}^2}{n_{2}^2}}} =\frac {h · n_{1}} { \sqrt{n_{2}^2-n_{1}^2}}$

We substitute r in relation (1) and raise the whole equation to the power of two:

$S=\pi r^2=\pi · \left( \frac {h · n_{1}} {\sqrt{n_{2}^2-n_{1}^2}}\right)^2= \pi · \frac {h^2 · n_{1}^2} {n_{2}^2-n_{1}^2}$

Numerical solution: We know from the task assignment: h=3 m.

We look up the values of π , n2 and n1 in the Tables:

Ludolph’s number $π =˙ 3.14$ Refractive index of air $n_{1} =˙ 1.00$ Refractive index of water $n_{2} =˙ 1.33$

We substitute for the values and obtain the size of the desired area:

$S =˙ \frac{3{.}14 · 3^2 .{1{.}00}^2}{{1{.}33}^2 - {1{.}00}^2}\mathrm{m^2}=\frac{3{.}14 · 9 ·1}{{1{.}33}^2 - {1}}\mathrm{m^2}=36{.}8\mathrm{m^2}$