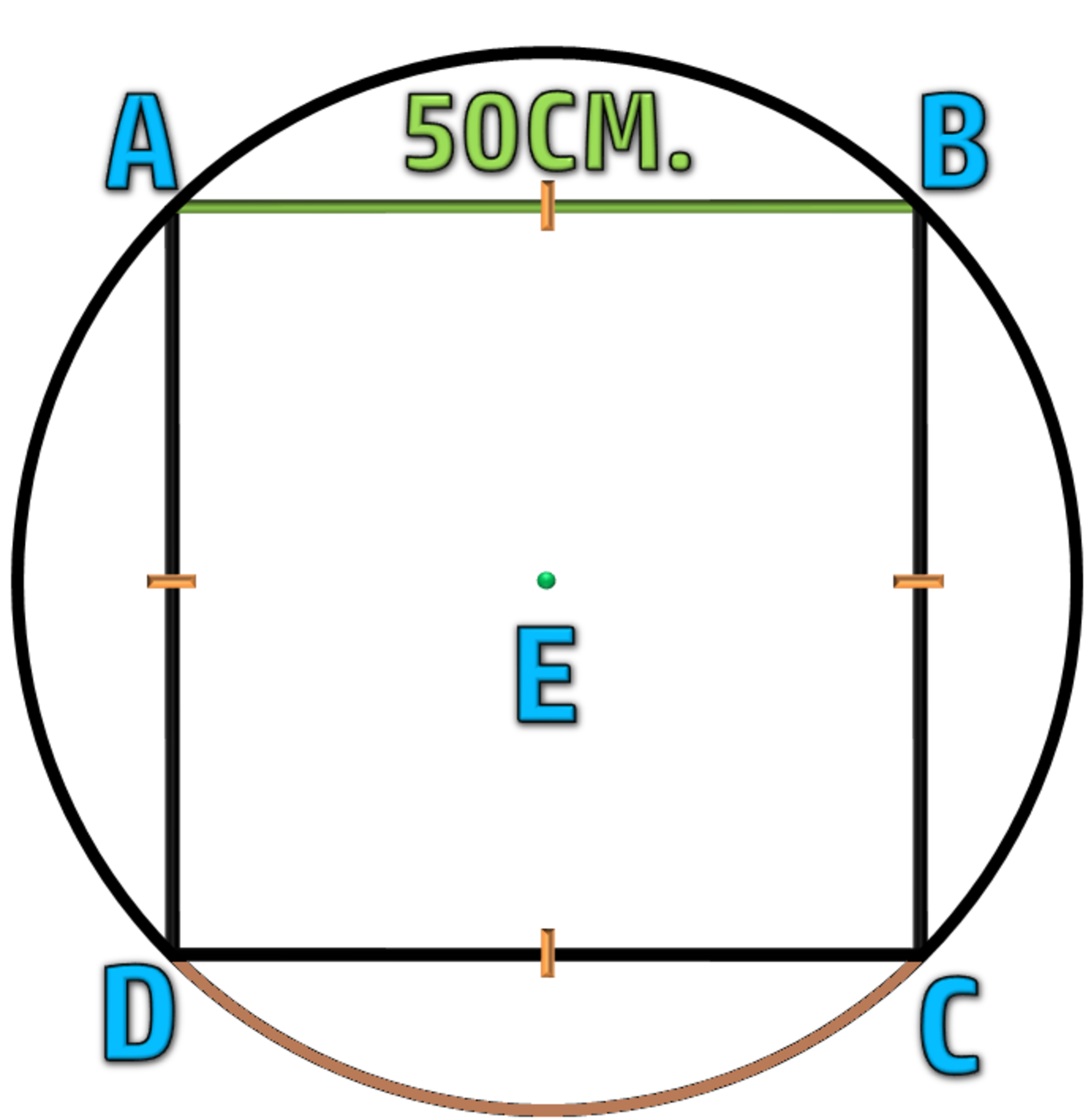
What is the brown arc length?

Geometry Level 3

  • A B C D ABCD is a square, Its side is 50 cm 50\text{ cm} .

  • E E is the circle center.

Find the brown arc length (in cm \text{cm} ).

Give your answer to 3 decimal places.


The answer is 55.536.

Yahia El Haw by Yahia El Haw , 18, Egypt

This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try refreshing the page, (b) enabling javascript if it is disabled on your browser and, finally, (c) loading the non-javascript version of this page . We're sorry about the hassle.

3 solutions

Radius of the circle is half the diameter of the square. But the brown arc is one fourth the circumference. ∴ b r o w n a r c = 1 2 ∗ ( 50 ∗ 2 ) ∗ ( 2 ∗ π ) ∗ 1 4 . = 55.5360 \therefore\ brown\ \ arc\ =\frac 1 2*\ (50*\sqrt2)\ *\ (2*\pi)\ *\frac 1 4.=55.5360

2 Helpful 0 Interesting 0 Brilliant 0 Confused
Yahia El Haw
May 29, 2016

2 Helpful 0 Interesting 0 Brilliant 0 Confused

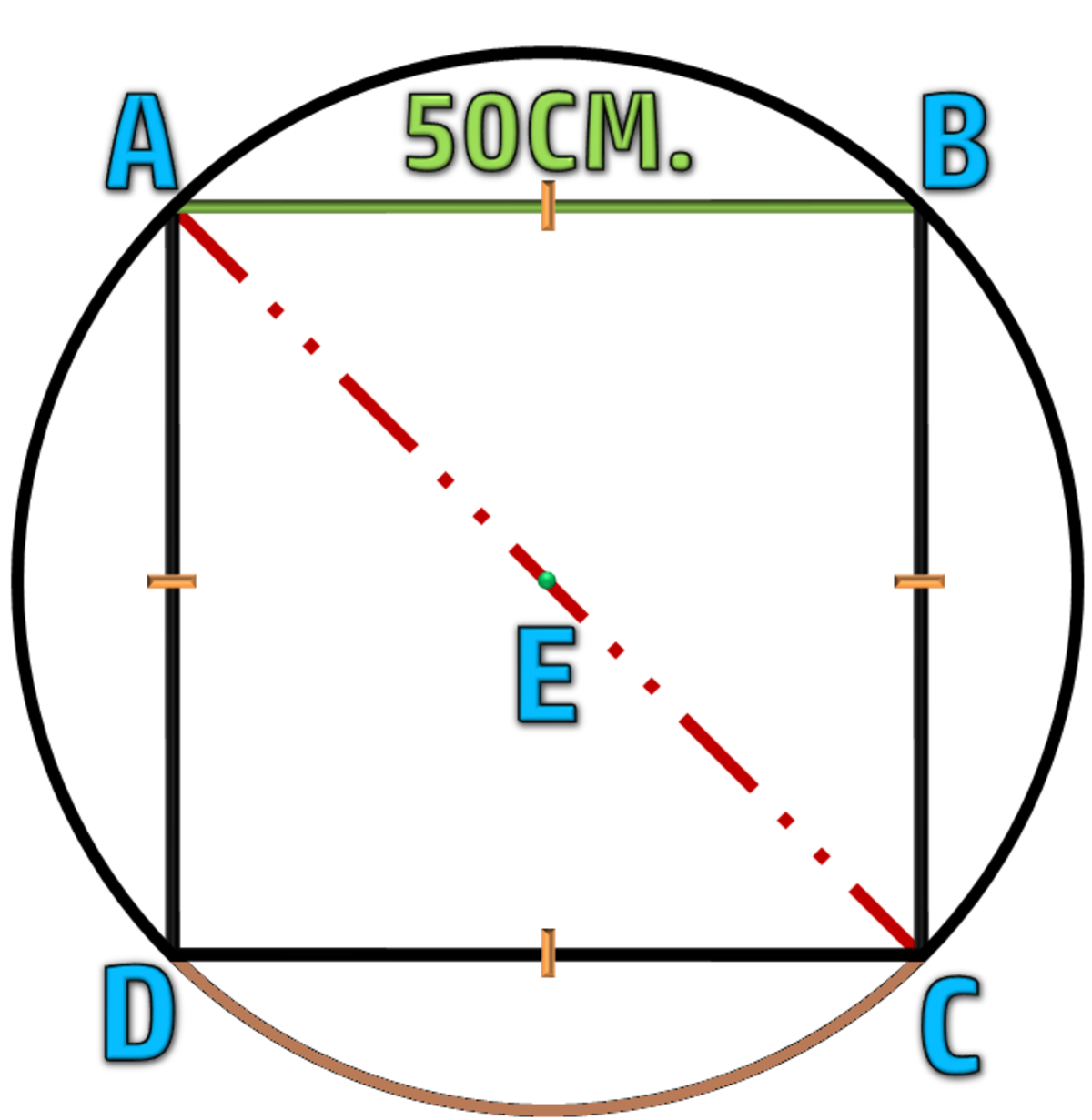
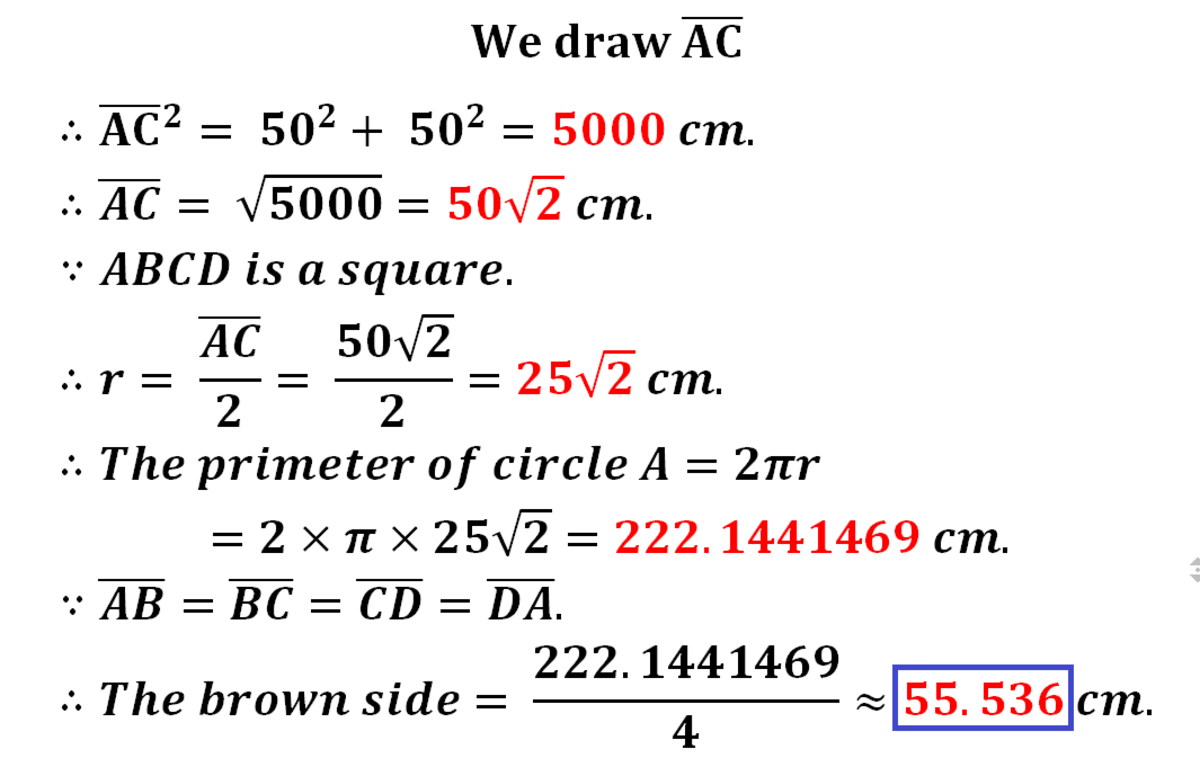
The diagonal of the square is the diameter of the circle. Because of symmetry, the length of the brown arc is equal to the circumference of the circle, divided by four. By pythagorean theorem, the diagonal is

d = 5 0 2 + 5 0 2 = 5000 d=\sqrt{50^2+50^2}=\sqrt{5000}

So the length of the brown arc is

c = 1 4 π d = 1 4 π ( 5000 ) = c=\dfrac{1}{4}\pi d=\dfrac{1}{4} \pi (\sqrt{5000})= 55.536 \boxed{55.536}

0 Helpful 0 Interesting 0 Brilliant 0 Confused

0 pending reports

×

Problem Loading...

Note Loading...

Set Loading...