What is the volume of this thing?
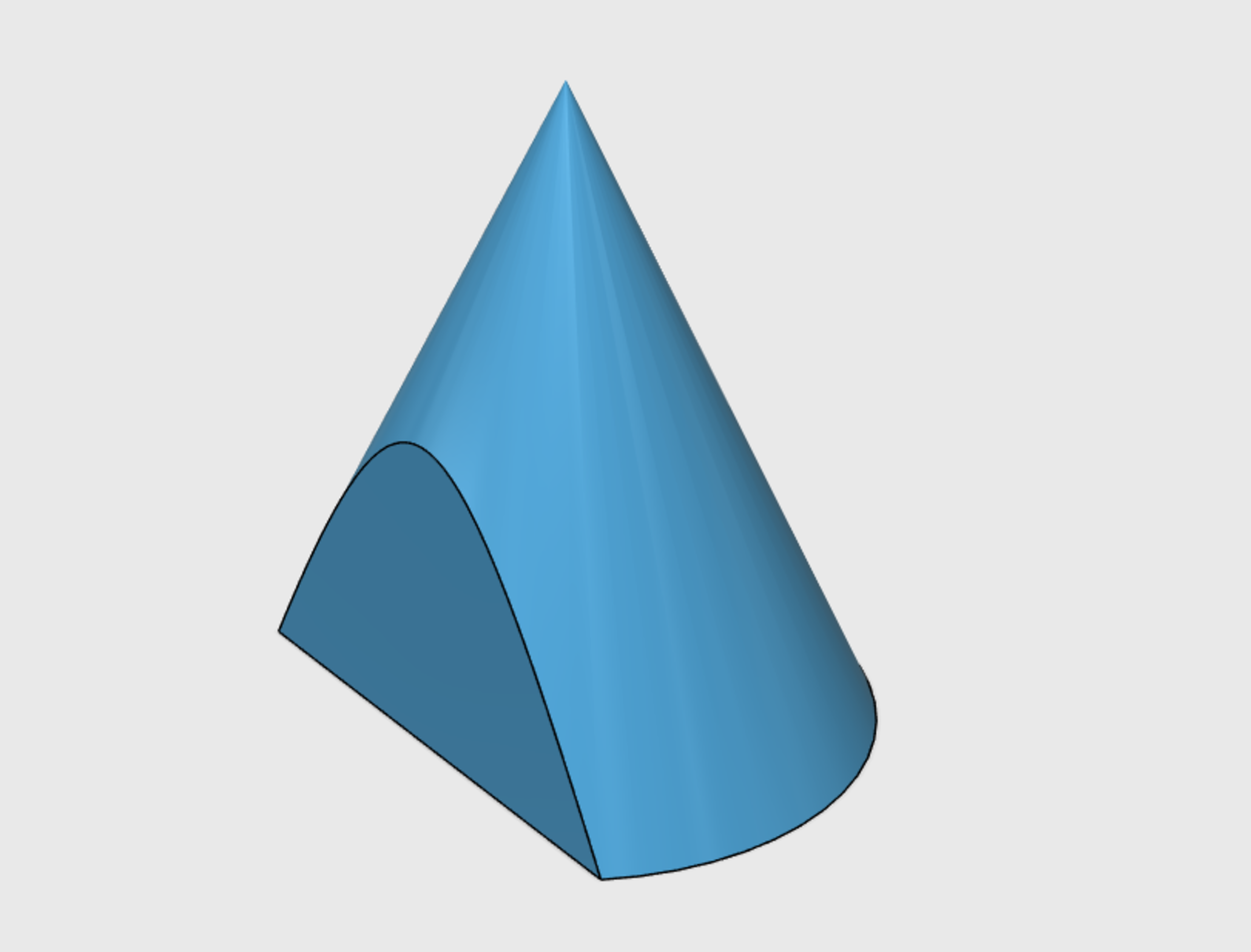
A right circular cone of base radius 20 cm and height 40 cm is cut vertically by a plane that is 1 0 cm from the axis of the cone. Find the volume of the cut cone (in cubic cm) ?
The answer is 14910.94.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
2 solutions
The volume removed from the original right cone of vertical height H & base radius R cut by a plane parallel to the symmetrical axis at a distance x is given by the most Generalized Formula
V r e m o v e d = 3 R H ( R 3 cos − 1 ( R x ) − 2 R x R 2 − x 2 + x 3 ln ( x R + R 2 − x 2 ) )
substituting the corresponding values, H = 4 0 , R = 2 0 , x = 1 0 , one should get
V r e m o v e d = 3 ⋅ 2 0 4 0 ( 2 0 3 cos − 1 ( 2 0 1 0 ) − 2 ⋅ 2 0 ⋅ 1 0 x 2 0 2 − 1 0 2 + 1 0 3 ln ( 1 0 2 0 + 2 0 2 − 1 0 2 ) ) ≈ 1 8 4 4 . 2 2 3 3 8 4
hence, the volume of cut cone
V l e f t = 3 1 π R 2 H − V r e m o v e d = 3 1 π ( 2 0 ) 2 1 0 − 1 8 4 4 . 2 2 3 3 8 4 ≈ 1 4 9 1 0 . 9 3 7 4 4 c m 3
If z is 2 1 of the vertical axis, then we integrate via slices parallel to the base
1 6 9 ( 3 1 4 0 ⋅ π ⋅ 2 0 2 ) + 2 ∫ 0 1 0 ( 1 0 ( 2 0 − z ) 2 − 1 0 2 + ( 2 0 − z ) 2 arcsin ( 2 0 − z 1 0 ) ) d z = 1 4 9 1 0 . 9 3 7 4 . . .
The volume of the top half of the cone is 8 1 of the whole cone, while the volume of half of the conical frustum is 1 6 7 of the whole cone. Add both to get 1 6 9 , and then add that integrated volume.