What's the area of this shape, -10? (I)
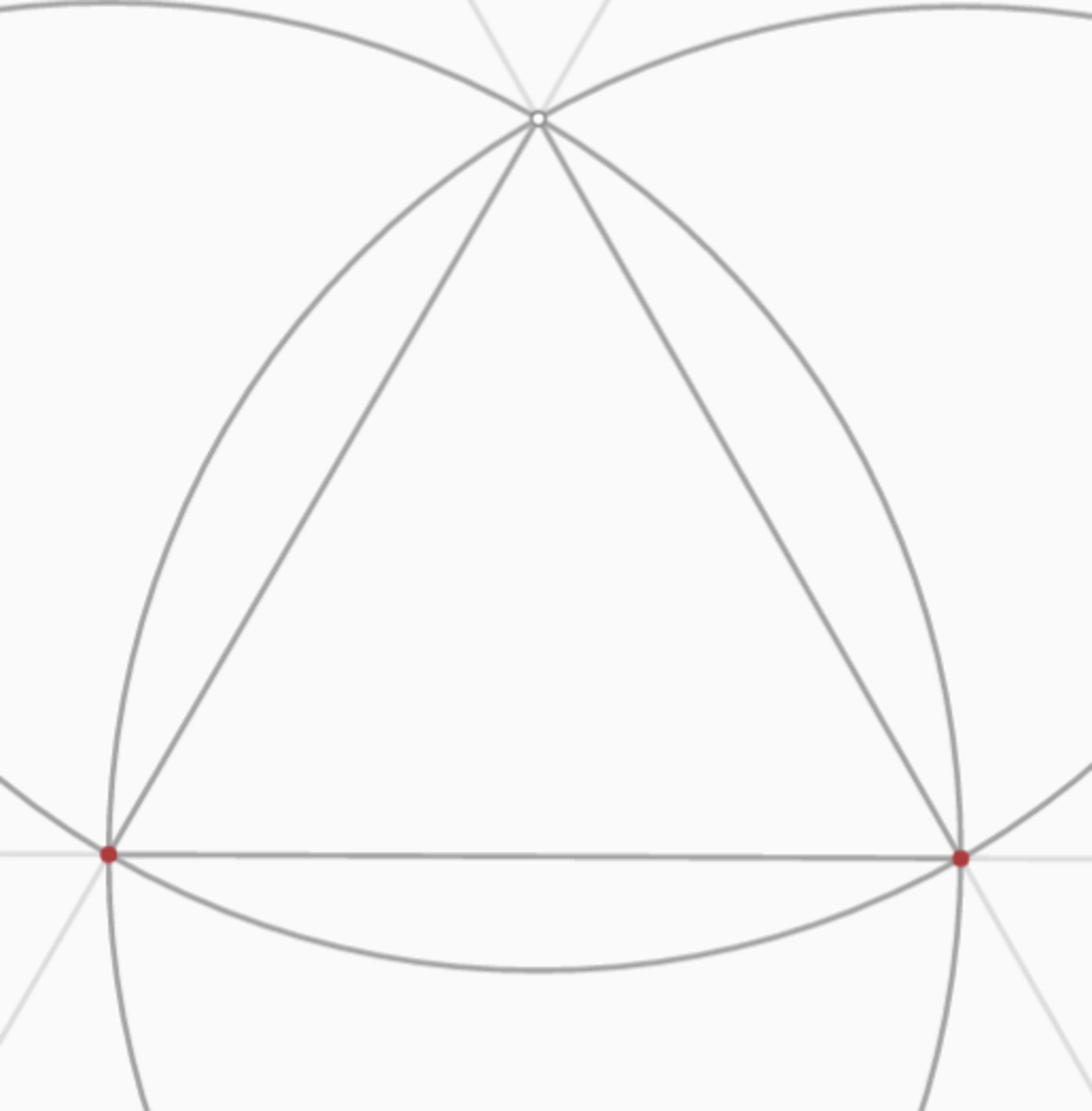
Find the area of the Reuleaux triangle given below, and subtract the value by 1 0 . Assume that all arcs that look like part of a circle to be part of a circle.
While calculating the area of the equilateral triangle, round off the area to a whole number. The side lengths of the equilateral triangle is 7. The value of π is 7 2 2
Report area : The report room
The answer is 25.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
3 solutions
Thanks a lot @Mahdi Raza for trying my question!
Area of the given shape is 3 × ( 2 1 × 7 2 × 3 π − △ ) + △ , where △ is the area of the equilateral triangle = 4 3 × 7 2 .
That is, the required area is 2 4 9 ( π − 3 ) ≈ 3 4 . 5 3 3 7 7 5 or 3 5 when rounded off.
The required answer is 3 5 − 1 0 = 2 5 .
Thank you a lot sir. Your solution is simply elegant! Thanks for trying out my question!
First we solve for the equilateral triangle's area.
A = 4 3 a 2 (where a is the side length of the triangle)
A = 4 3 × 4 9
A ≈ 2 1 . 2 1 7 6 2
By rounding off, we get:
A ≈ 2 1
Then, we find the area of the outer three circle chords by using the area of a sector of this circle
3 6 0 6 0 × 7 2 2 × 7 × 7
6 2 2 × 7
2 5 3 2
Subtracting from the triangle's area, we get:
2 5 3 2 − 2 1 = 4 3 2
When multiplying by three to get the area of all the three chords, we get
4 3 2 × 3 = 1 4
By addition to get the total area of the Reuleaux triangle, we get:
2 1 + 1 4 = 3 5
According to the question, we should subtract the final value by 1 0
3 5 − 1 0 = 2 5
"While calculating the area of the equilateral triangle, round off the area to a whole number"
This is mentioned in the quote
Log in to reply
ok sorry, but I will still advise you to change it cuz it may affect the calculation in different ways.
Log in to reply
Check @Mahdi Raza 's solution. They didn't round it off and still got a correct answer.
You can see @Alak Bhattacharya 's solution too if you like. They both explained the question properly.
A Reuleaux triangle = 3 × A segment + A triangle . Each of the 3 minor segments is equal due to symmetry, hence their areas are equal. The area is equal to:
A segment A triangle = A sector − A triangle = ( 3 6 0 6 0 ⋅ π ( 7 ) 2 ) − ( 4 3 ⋅ ( 7 ) 2 ) = ( 7 ) 2 × ( 6 1 ⋅ π − 4 3 ) = ( 4 3 ⋅ ( 7 ) 2 )
A Reuleaux triangle A Reuleaux triangle = 3 × A segment + A triangle = 3 × ( 7 ) 2 × ( 6 1 ⋅ π − 4 3 ) + ( 4 3 ⋅ ( 7 ) 2 ) = ( 7 ) 2 × ( 2 1 π − 4 3 3 + 4 3 ) = ( 7 ) 2 × ( 2 1 π − 2 1 3 ) = 2 1 ( 7 ) 2 ( π − 3 ) ≈ 3 5 ⟹ Answer is 35 - 10 = 2 5