Where Should The Earth Go?
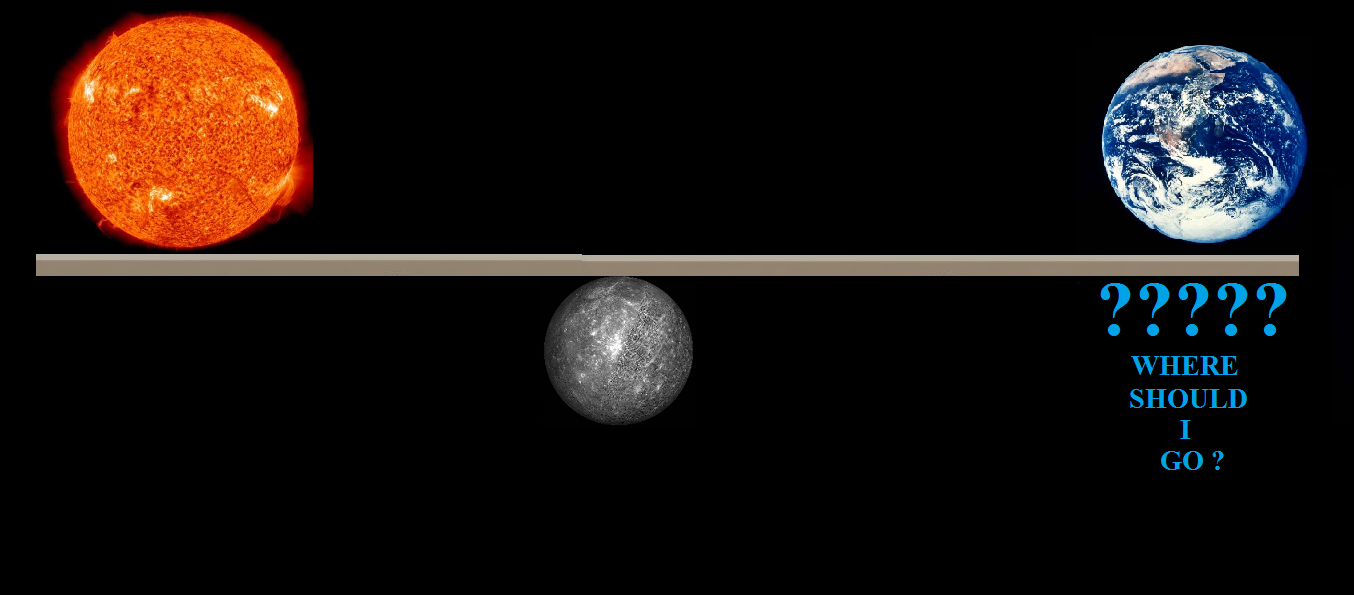
The Earth and the Sun want to play seesaw. They decided to make mercury as the pivot point (fulcrum). The earth wanted to come on the same level as the sun so it went somewhere in the universe to sit at such a position such that he could balance the sun and come in the same level.
How far did the Earth go from its original position ?
If your answer is
km, submit
as your answer.[after rounding the answer to tenth ( 1/10 ) digit ].
Take :--------
Mass of Sun = 2 * 10^30 Kg
Mass of the Earth = 6* 10^24 Kg
Dist. between Sun and Mercury =58,000,000 Km
Dist. between Mercury and earth = 92,000,000 Kms
Assume that gravity works in the space just like it does on earth.
The answer is 19.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
This question is based on the first class of lever. Here mercury is the fulcrum and sun and earth are on either sides of the seesaw Therefore, Mass of Sun * Distance between sun and mercury = Mass of Earth * Distance between mercury and Earth . ( after Earths displacement )
=> 2 * 10 ^30 * 58,000,000 = 6 * 10^24 * x
=> y = 6 ∗ 1 0 2 4 2 ∗ 1 0 3 0 ∗ 5 8 , 0 0 0 , 0 0 0 km
= 1.9333333333......... * 10 ^13 km
Now , Earth's displacement from its original position = Distance between earth and mercury (after earth's displacement ) - Original . distance between earth and mercury
= 1.933333333.....*10^13 km - 92000000 km
= 19333333333333 km - 92000000 km
= 19333261333333 km
~ 1.933333..... * 10^ 13 km
= 19.33333..... * 10^ 12 km So the answer is19