Where'd the Extra Square Come From?

Logic Level 2

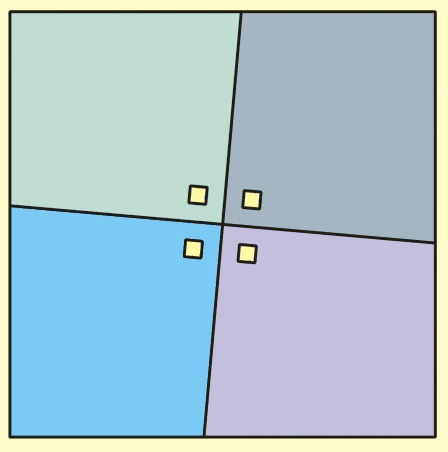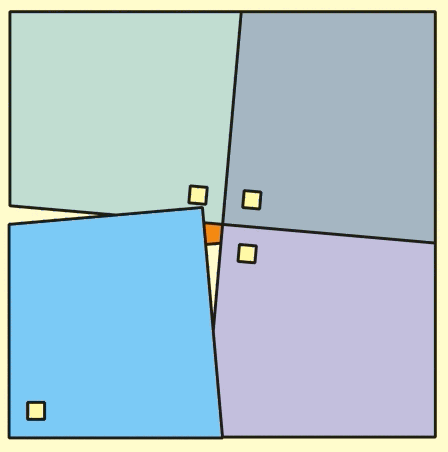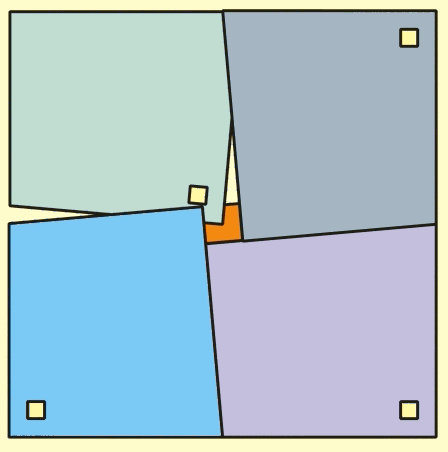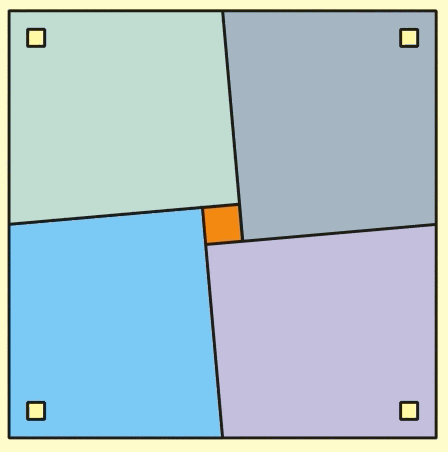Four quadrilaterals are arranged to form a square. Then each quadrilateral is rotated and an orange square appears at the center, but the total area seems unchanged. If each quadrilateral doesn't change size when it's rotated, then how do you explain the creation of this orange square?

The yellow squares in the corners of each quadrilateral cause the extra area The original big square is smaller than the big square in the final frame Rotating shapes causes them to shrink, allowing the red square to exist At least one of the quadrilaterals is not congruent to the others

View wiki
Andrew Ellinor by Andrew Ellinor , 33, USA

This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try refreshing the page, (b) enabling javascript if it is disabled on your browser and, finally, (c) loading the non-javascript version of this page . We're sorry about the hassle.

2 solutions

Andrew Ellinor
Oct 8, 2015

The side of the new large square is slightly larger than the side of the square in the original arrangement. It's just barely detectable, but the final frame's large square is about 1% larger in area. In the image above you can see that the side length is just a bit longer in the figure on the left, allowing for the extra area in the middle.

19 Helpful 0 Interesting 1 Brilliant 0 Confused

I don't like this problem.

Patrick Engelmann - 5 years, 8 months ago

Log in to reply

Me neither, who could've spotted that??

Digital Gaming 227 - 12 months ago

Tricky right?

Andrew Ellinor - 5 years, 8 months ago

That is how I came up with my answer as well, however I read the comments on Facebook to verify that I wasn't just perceiving it (our brains have a way of showing us what we want to see).

With that said:

I read a comment that suggests we look at the lengths of each side in the quadrilaterals.

We can see that the perimeter of the large square is using one of the smallest sides of each of the four quadrilaterals (Two adjacent angles that total more than 180).

When the quadrilaterals are rotated that smaller side is then placed on the interior of the big square.

This was brought to my attention from Mohamad Abd Elrahman and a reply by Nimrod De la Peña (That clarified what Mohamad had already said)

(This explanation, though, isn't completely adequate because the perimeter consists of two sides of each quadrilateral. It may be possible that sides (A + B) < sides (C + D). (After work I have some further thoughts I want to throw out there about the efficacy of this problem and perhaps a proof which shows the GIF is also dishonest.)

Ian McKay - 5 years, 8 months ago

To make second square, the quadrilateral re arranged. Then space occured in the middle.

1 Helpful 0 Interesting 0 Brilliant 0 Confused

The size of the final square changed, refer to solution above

Digital Gaming 227 - 12 months ago

0 pending reports

×

Problem Loading...

Note Loading...

Set Loading...