Inelastic but the disc comes back
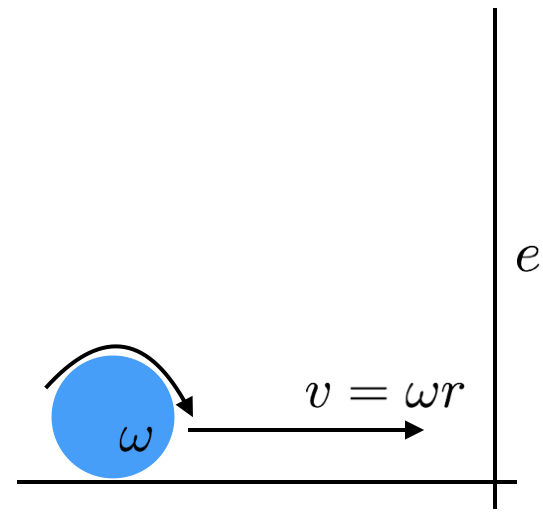
A uniform disc is rolling on rough ground as shown in the figure.
The disc is about to collide with a frictionless wall. Find the maximum value of the coefficient of restitution, e , between the wall and the disc so that disc will collide with the wall a second time.
Details
- Radius of the disk is R = 1 m .
- The mass of the disc is M = 1 5 k g .
- The velocity of the disc is 2 5 m / s .
The answer is 0.5.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
2 solutions
Great solution! And nice problem @Harsh Shrivastava keep posting more like these! 😃😃
Log in to reply
Sure!
But you also keep posting any interesting CM problems you come across!
After the collision (and until rolling resumes), let the disc have speed V towards the wall, and angular velocity ω . The disc experiences a friction force F = μ m g towards the wall. Then Newton's Laws state that m V ˙ = F 2 1 m r 2 ω ˙ = − F r so that V ˙ = μ g and ω ˙ = − r 2 μ g . Thus we deduce that (with time t = 0 representing the moment of collision) V = μ g t − e v ω = r v − r 2 μ g t until rolling resumes, and this occurs when μ g t − e v = V = r ω = v − 2 μ g t namely when t = 3 μ g ( 1 + e ) v . At this time we have V = 3 1 ( 1 − 2 e ) v and so rolling resumes with the disc still heading towards the wall (and so a second collision occurs) provided that e < 2 1 . The supremum of possible values of e is thus 2 1 .
Note that, if e = 2 1 , the cylinder stops skidding precisely when V = 0 and ω = 0 . Thus the cylinder stops skidding, coming to rest at the same time. This means that the cylinder will not return to collide with the wall a second time in this case. The set of values of e for which a second collision is possible does not have a maximum.
It is interesting to note that, during the skidding process, the distance of the cylinder from the wall is e v t − 2 1 μ g t 2 Thus, if μ g 2 e v < 3 μ g ( 1 + e ) v , which occurs if e < 5 1 , the cylinder strikes the wall a second time before the skidding process stops.
Log in to reply
Better to let one the Brilliant people, like @Calvin Lin , mark the report as resolved, which it now has been..
It has now been done.
After collision , let the disc have speed V towards from the wall . Shouldn't there be "away" in place of "towards".
Log in to reply
No. You will note that V < 0 initially, so V is the velocity towards the wall. The condition for a second collision with the wall is that V > 0 when rolling resumes...
The best way to solve this problem is by using angular momentum conservation in the following way
Just After colloision the disc rebounds with a velocity e . V towards the left with no change in angular velocity.
Now after some time the disc will again start pure rolling now what we want is that the disc to move towards the right when it again starts pure rolling .At that moment the angular momentum about any point on the ground would be tending to 0 and into the plane (let's denote it by -ve sign).
Now since the angular momentum remains conserve about that point so
Angular momentum just After colloision= final angular momentum
⇒ M . e . V . R − ω I c o m ≤ 0
⇒ M . e . V . R ≤ 2 M R 2 ω
⇒ e ≤ 2 1