Whole numbers

Algebra Level 1

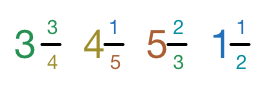Which of the following fractions, when added to the sum of the above numbers, makes the result a whole number?

1 60 \dfrac{1}{60} 7 60 \dfrac{7}{60} 51 60 \dfrac{51}{60} 53 60 \dfrac{53}{60}

View wiki
Anuj Shikarkhane by Anuj Shikarkhane , 19, India

This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try refreshing the page, (b) enabling javascript if it is disabled on your browser and, finally, (c) loading the non-javascript version of this page . We're sorry about the hassle.

5 solutions

Sum up all the numbers in the sequence. Then, the sum in mixed fraction is subtracted from the nearest whole number i.e. 16-(907/60)=53/60

4 Helpful 0 Interesting 0 Brilliant 0 Confused

Do we have a smarter way? Or its just like solving exercise question from Math books!

Sahil Sayed - 6 years, 7 months ago

Log in to reply

one may add only the fractions, ignoring the whole numbers to get the sum as 127/60 , the fraction part of which is 7/60. Therefore, the answer is 1- 7/60= 53/60

J Chaturvedi - 6 years, 7 months ago

3.75+4.2+5.67+1.5=15.12; Round up to 16 and the difference is .88 so .88*60=53/60

Ivan Seward - 6 years, 7 months ago

Log in to reply

The statement of the problem is slightly misleading... I thought that "3 3/4" was meaning 3*3/4, and not 3+3/4... Notation puzzle!

Magic FX - 6 years, 7 months ago
Ayush Goyal
Jan 31, 2015

Add the fractions 225+252+340+90/60 which is equal to 907/60. Only 53/60 would make it a whole number

1 Helpful 1 Interesting 2 Brilliant 1 Confused
Remzy Yimam
Dec 17, 2014

3.75+4.2+5.67+1.5=15.12; Round up to 16 and the difference is .88 so .88*60=53/60

0 Helpful 0 Interesting 0 Brilliant 0 Confused
Rohan Shah
Nov 11, 2014

the fraction above can be written as:

(3+4+5+1)+(3/4 + 1/5 + 2/3 + 1/2)

You only need a fraction that will make 3/4 + 1/5 + 2/3 + 1/2 be a whole #.

So,

3/4 + 1/5 + 2/3 + 1/2 + x/60 should be a whole #.

So,

(45+12+40+30+x)/60 should be a whole #.

(127+x)/60 should be a whole #.

Smallest # that can equip x: 180≤127+x≤60n

53≤x≤60n-127

Therefore, the answer is: 53/60 .

0 Helpful 0 Interesting 0 Brilliant 0 Confused
Viveak Mp
Oct 30, 2014

The LCm of the fraction is 60. So adding the fractions ll be (225+252+340+90 = 927). So adding 53 to it makes it a number divisible by 60.

0 Helpful 0 Interesting 0 Brilliant 0 Confused

It would be 907, not 927

Anirudha Brahma - 5 years, 2 months ago

0 pending reports

×

Problem Loading...

Note Loading...

Set Loading...