Will They Ever Meet?

Relevant wiki: Geometric Probability - Problem Solving

From this question, the probability runs in a continuous time period, so it is prudent to understand the circumstances in each point in time first.

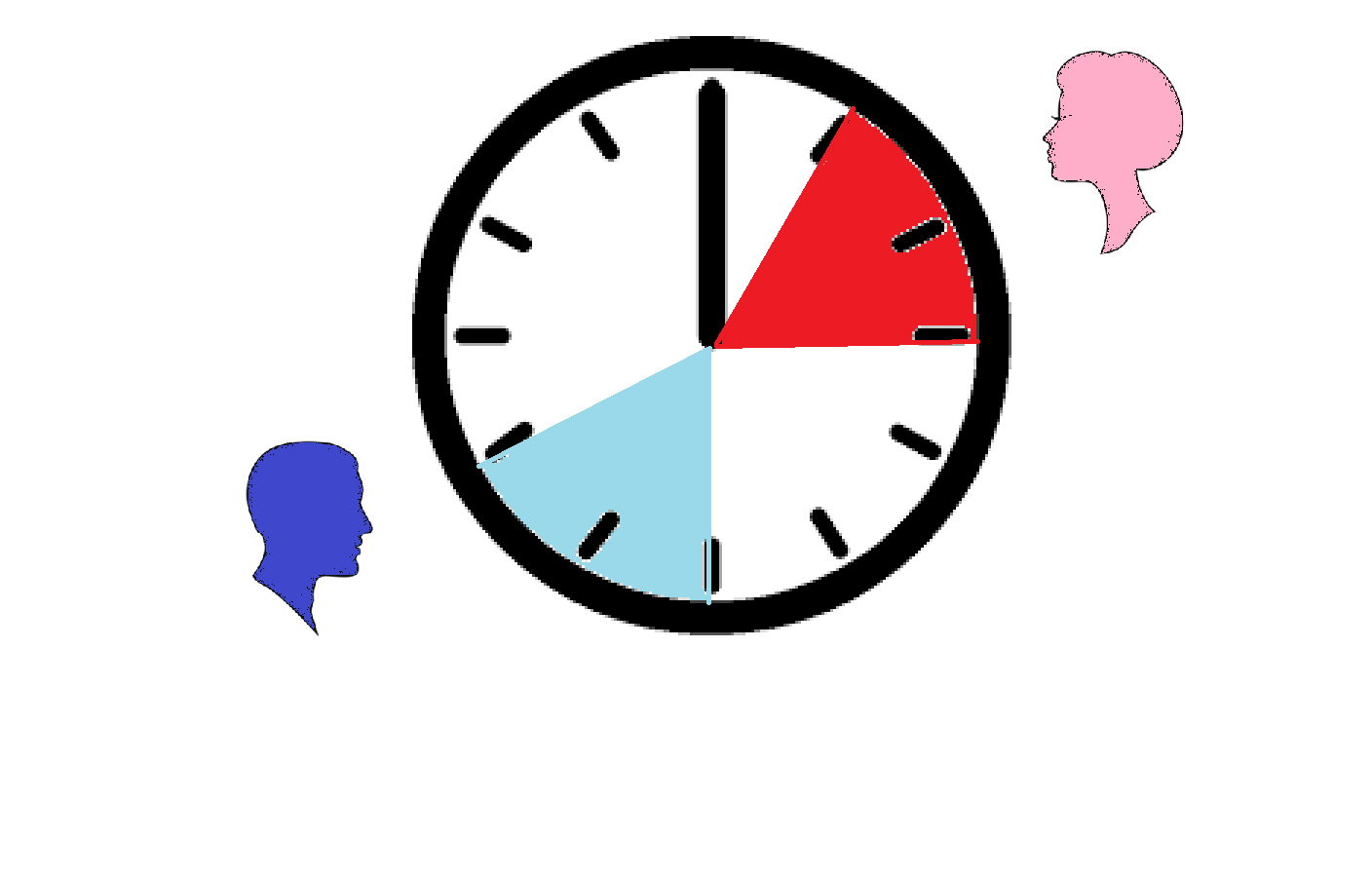
Starting with Sasha coming right on noon time, she will wait for Joel for $10$ minutes, so the only way Joel can meet her is that he must come between 12:00 - 12:10 P.M. (10 minutes) as shown below:

Now if we shift Sasha's time to come at 12:05 P.M., Joel can similarly meet her around 12:05 - 12:15 P.M. but also can come to wait for her since 12:00 because he can wait until 12:10 P.M.. Therefore, the time frame for this case is 12:00 - 12:15 P.M. (15 minutes) as shown below:

Then when shifting her time to come at 12:10 P.M., Joel can still barely make it in time to meet her if he comes in since 12:00 P.M.. Hence, the time frame is from 12:00 - 12:20 P.M. (20 minutes) as shown below:

The time frame of 20 minutes will be the same until Sasha comes in later than 12:50 P.M.. For example, if she comes at 12:55 P.M., the time frame for Joel will be reduced to 15 minutes (because he will not come later than 13:00 P.M.) as shown below:

Finally, if Sasha decides to come in the last minute of lunch time, Joel will only have a time frame of 10 minutes prior to wait for her as shown below:

Now by using geometric probability, we can visualize the time portion as a pie chart within the whole circular clock. For instance, at the beginning, Joel has 10 minutes, so P(t = 0) = $\dfrac{10}{60} = \dfrac{1}{6}$ .

Then by gathering all the probability $P$ plots over time $T$ , we can get a function graph as shown below:

Finally, by calculating the average area under graph over 1 hour time, we can evaluate the probability for this couple's meeting:

$P = \dfrac{1}{3} - (\dfrac{1}{6})(\dfrac{1}{6}) = \dfrac{11}{36}$ .

Thus, $a + b = 11 + 36 = 47$ .

Let Sasha arrive at 12:x pm and Joel arrive at 12:y pm

Our constraints on x and y are:

$x \leq 60$

$y \leq 60$

The above 2 equations is our total sample space.

$|x-y| \leq 10$ which gives,

$x \leq y+10$ and

$y \leq x+10$

The above 2 equations give the favorable conditions of meeting.

Plotting these 4 equations on the graph, and plotting the areas given by them, the probability of meeting is given by,

$P = \frac{3600-2500}{3600} = \frac{11}{36}$

So a+b = 47

when I did this problem,47 percent of people got it right -_-.