Wire Frame Cube Moment

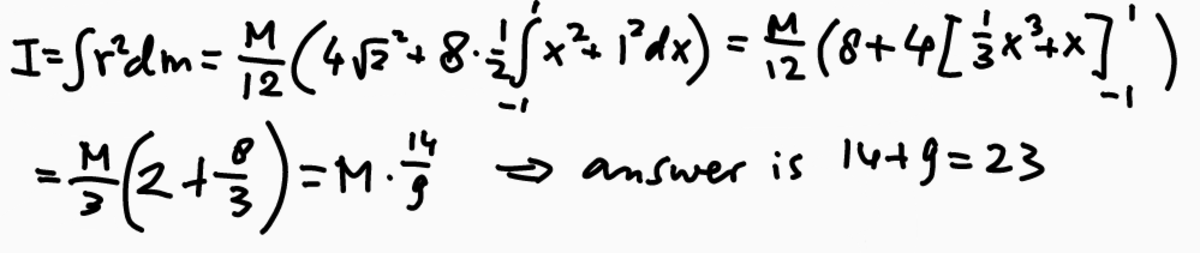
Each of the 12 constituent line segments has a mass $\frac{M}{12}$ and a length of 2. The 8 segments located at either $(z = 1)$ or $(z = -1)$ can all be treated the same way. Using the Parallel Axis Theorem (since the center of each segment is 1 unit away from the origin) and the expression for the moment of inertia for a rod about its middle $(\frac{M l^{2}}{12})$ , the moment for each of these 8 segments is:

$\frac{M}{12} * \frac{2^{2}}{12} + \frac{M}{12} * 1^{2} = \frac{M}{36} * \frac{3M}{36} = \frac{M}{9}$

The moment for the 8 segments is therefore $\frac{8M}{9}$

The remaining 4 segments all have the entirety of their mass located a distance of $\sqrt{2}$ from the origin, so we can simply use the $mr^{2}$ formula for those. The combined moment of inertia for those 4 segments is:

$4 \frac{M}{12} (\sqrt{2})^{2} = \frac{8M}{12} = \frac{2M}{3} = \frac{6M}{9}$

The total moment is therefore $\frac{8M}{9} + \frac{6M}{9} = \frac{14M}{9} = \frac{aM}{b}.$

$(a+b) = 23$