You'll be stuck
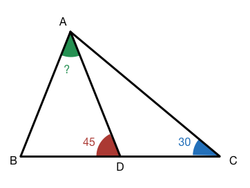 In
△
A
B
C
, let
D
be the midpoint of
B
C
. If
∠
A
D
B
=
4
5
∘
and
∠
A
C
D
=
3
0
∘
, what is
∠
B
A
D
in degrees?
In
△
A
B
C
, let
D
be the midpoint of
B
C
. If
∠
A
D
B
=
4
5
∘
and
∠
A
C
D
=
3
0
∘
, what is
∠
B
A
D
in degrees?
The answer is 30.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
2 solutions
You are Un-Comparable!!!!!!!!!!! The last step is a beauty
Good use of sine rule
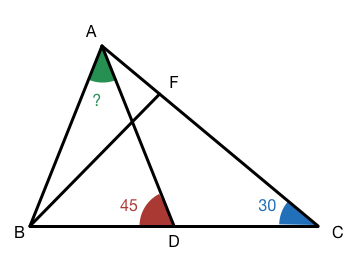
Let BF be orthogonal to the side AC with point F on AC. As angle DCB equals 30, then from the right triangle we get B F = B C sin 3 0 ∘ = B C / 2 = B D = D F .
Then, ∠ F B D = 6 0 ∘ and B F = F D , so B F D is an equilateral triangle. This gives us ∠ F D B = 6 0 ∘ and ∠ F D A = 6 0 ∘ − 4 5 ∘ = 1 5 ∘ .
Since ∠ F A D and ∠ D F A are both 1 5 ∘ , so F A D is an isosceles triangle with A F = F D . Since A D = F D = B F , thus A F B is an isosceles triangle and ∠ F A B = 4 5 ∘ .
Thus, ∠ B A D = ∠ B A F − ∠ D A F = 4 5 ∘ − 1 5 ∘ = 3 0 ∘ .
Let B C = a , A C = b , A B = c , A D = d and ∠ B A D = θ . We note that ∠ B A C = θ + 1 5 ∘ . Using Sine Rule, we have:
⎩ ⎪ ⎨ ⎪ ⎧ 2 a sin θ = c sin 4 5 ∘ a sin ( θ + 1 5 ∘ ) = c sin 3 0 ∘ ⇒ sin θ = 2 2 c a ⇒ sin ( θ + 1 5 ∘ ) = 2 c a
⇒ sin θ sin ( θ + 1 5 ∘ ) = 2 = 2 1 2 1 × 2 = 2 1 2 1 = sin 3 0 ∘ sin 4 5 ∘ ⇒ θ = 3 0 ∘