Acknowledgement

I owe this to @Amrita Roychowdhury whom I thank for being one of the few people who could pay attention to my Kaboobly Doo.

To The Reader

For the Calculus Pro -

I understand that you do not like the way I explained this. But I have already mentioned in the comment that this is way too informal though it is actually a very good way to teach or to introduce the idea to a beginner.

For the Calculus Newbie -

Do not just read this like a story. View yourself as Amrita and when Agnishom asks a question to Amrita, try to work it out yourself. Hopefully, it will answer many questions and strengthen your foundations. If you really think that this seems to be more friendly than the usual Calculus text-book approaches, then I will recommend you read Calculus Made Easy by Silvanus Thompson.

Day 1

Agnishom:

Lets get started with some differential calculus. Are you ready Miss Roychowdhury?

Amrita:

oh yes,Agnishom, let's start.

Agnishom:

Okay;

First of all: d is an operator in calculus. it actually means a very small part of something. E.g, dx means a small part of x.

In differentiation, we are concerned with the ratio of change of one quantity at a particular instant with respect to another.

Consider the simple equation: y=2x

Now, we will consider how much y changes when x is increased by a little amount.

What can you say about the change of y with respect to x?

Amrita:

i think if x increases,then y will also increase proportionally.

Agnishom:

You are correct. Differentiating is the art of finding out this proportionality constant at a particular point on the graph.

y=2x

Suppose x=1, then y=2

Suppose x=1.0001, then y = 2.0002

Thus, dx=0.0001 and dy=0.0002 [dx is a very small quantity of x, very very small]

Can we say that dy/dx = 2 ?

Amrita:

yes,we can say that.

Agnishom:

Suppose, we are revolving a stone tied in a string. When the string breaks, the stone escapes tangentially? Why? Because that was the way the stone was looking at, at the instant of the breakage.

Similarly, at a particular point on the graph, the slope or the dy/dx we are considering is that of the tangent at that point. Will you see a figure?

Amrita:

yes,show the fig.

Agnishom:

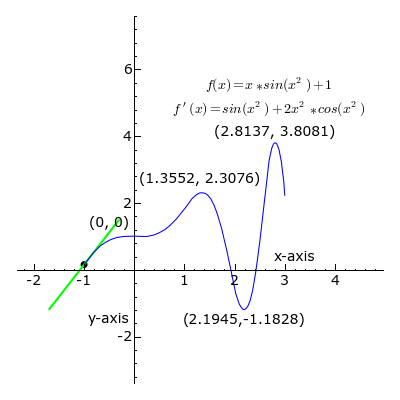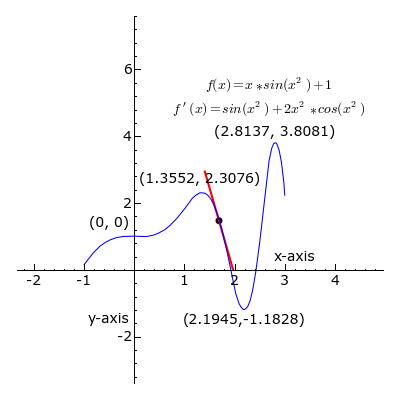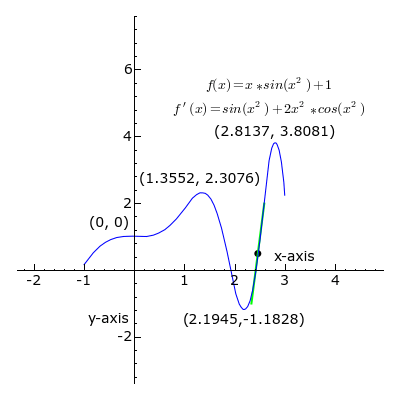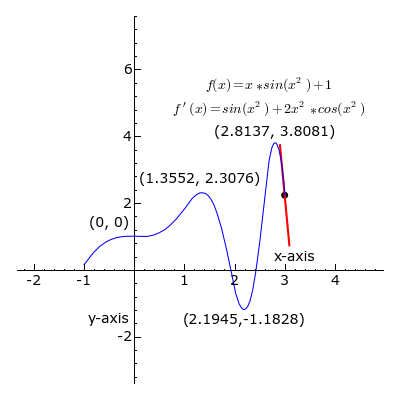derivatives

Do not look at the confusing notations yet.

The tangent on the curve is not the same everywhere. Somewhere, it has a steep slope, somewhere it hasn't. Do you agree?

Day 2

Amrita:

Hello Agnishom. Let's start Calculus.

Agnishom:

Okay, a little recaptulation...

Do you remember what dx means?

If yes, did I tell you what dy/dx means?

Amrita:

dx means a small part of x.

dy/dx refers to the change of y if x is changed by small amount.

am I right, Sir?

Agnishom:

Ma'am, I'd like to change the second defintion a little. dy/dx refers to the rate of change of y if x is chnged by a small amount.

You can do this on a plane paper: Draw the parabola $y=x^2$

Now take two points on it and join them with a straight line. Again, take two points which are even closer nd join them by a line.

You will notice that the closer you get, the more the line looks like tangent at that region.

Yes?

Amrita:

Yes Sir. I drew the parabola.

Agnishom:

Call me Agnishom, or Sushil, if you like.

Do you agree that the closer the points, the more the line joining them looks like a tangent at that point?

Amrita:

Yes. Btw is ur another name Sushil?

Agnishom:

No, the guy in this picture is called Sushil:

sushil sushil

Suppose the two points we chose were (x,y) and (x + dx, y + dy). Since, dx and dy are very very small this is the tangent exactly.

Suppose we, join (x,y) and (x + dx, y + dy). So, the slope of the line is $\frac{(y + dy) - y}{(x+dx) -x}=\frac{dy}{dx}$

In other words, when we are differentiating then we are essentially finding the slope of the tangent at that point on the function.

Do you agree?

If yes, lets get on to doing differentiation

Amrita:

Yes Agnishom, I agree with it.

Agnishom:

Lets do some differentiation then.

We do differentiation to find a derivative.

Let us consider a curve of the equation $y = f(x) = x^2$

We will differentiate f(x)

Suppose we have chosen the two points on the curve (x,x^2). If the x coordinate of the second point is $x+dx$ , then what is the y coordinate of the second point?

Hint: Use the equation of the curve

Amrita:

I think it's y+dy.

Am I right?

Agnishom:

Can you express it in terms of x and dx?

Amrita:

$y+dy=(x+dx)^2$

Is it? I am not sure. Please tell me.

Agnishom:

Yes. It is.

Now, $y+dy=(x+dx)^2= x^2+2 \; x\; dx + (dx)^2 = x^2+2 \; x\; dx + d^2x$

We write (dx)^2 as d^2x just like (sin x)^2 is sin^2 x

Now, note that dx is a very very small part as compared to x. d^2x is even smaller.

Just for an example, if dx = 0.0001x, then d^2x = 0.0000001x which is even less small compared to x.

Since, we are only interested in dx and dy, we will neglect small quantities of the second order like d^2x.

So, $y+dy=(x+dx)^2= x^2+2 \; x\; dx + (dx)^2 = x^2+2 \; x\; dx + d^2x = x^2+2 \; x\; dx$

Do you understand why it is fair to do this? Is everything clear uptil now?

Amrita:

And (dx)^2 should be d^2 x^2 not just d^2 x. Is it so?

Agnishom:

Nope.

We write (dx)^2 as d^2x just like (sin x)^2 is sin^2 x

dx does not mean d multiplied with x. It is a special kind of a operator meaning a small part of x

Amrita:

o. then we can proceed now or r u tired?

Agnishom:

I am fine. I just got lost in a deep ocean, so I am late to reply...

We've got two equations: $y+dy= x^2+2 \; x\; dx$ $y = x^2$

Please do this:

Subtract the second equation from the first
Divide both sides by dx

Then, post the results.

Amrita:

Then, Agnishom dy/dx=2x.

Agnishom:

:D Great!

We say that the derivative of x^2 is 2x

Can you try and find out the derivative of x^3 in the same way?

Amrita:

I think derivative of x^3 is 3x^2.

Agnishom:

Very good.

There is a theorem that tells the derivative of $x^a$ is $a x^{a-1}$

You'll be taught the proof in school but just remember the rule for now.

Amrita:

O.K. then?

Agnishom:

Let us study two more kind of cases.

Find the derivative of f(x) if f(x) = 7
Find the derivtive of 9x.

Try doing these

Amrita:

I am feeling very tired now. I will try to do these and inform u later. Will that be o.k.?

And what will be y if f(x)=7? Similarly what about 9x?

Agnishom:

y=f(x)=7 which means y is always 7.

These two cases are supposed to be solved by common sense or a graph rather than those type of calculations we did before.

Shall I explain it today or are you tired?

Amrita:

First let me try, Agnishom.

Agnishom:

Okay.

I've a request for you.

Amrita:

Yes Agnishom, speak out.

Agnishom:

Although trying to do these like the x^2 and x^3 will give correct results, I want you to do it by looking at their graphs or some simple reasoning.

There are two reasons why I want you to do it with the graphs:

You get a feeling of what you're doing.
Rakesh Babu would agree with it.

If you think plotting them by hand is tedious, I can plot the graphs with a computer and post here. Should I?

Amrita:

Sure Agnishom, I must listen to you.

Agnishom:

I mean do you want me to post the graphs or do you prefer drawing them yourself?

Amrita:

Post it for me,plz.

Agnishom:

Find the derivative of f(x) if f(x)= 7

Here is the plot of y = 7

y=7 y=7

Find the derivative of f(x) if f(x) = 9x

Here is the plot of y = 9x

f2

Amrita:

Please give some hints to find out the Derivatives.

Agnishom:

Hint 1: Derivative reffers to the slope of the tangent at a particular given point.

Hint 2: These two graphs have the same tangents and slope everywhere.

Amrita:

Please explain me the problems

Agnishom:

Look at the first problem

We say that dy/dx is the rate of change of y with respect to small changes of x.

Suppose, x=1 => y=7

If, x = 1.0001, y = 7

If x = 2, y = 7

So, y does not change with x

Fill up the blank: Thus, the change of y is _

Amrita:

Not possible as it is constant for any value of x.

Agnishom:

Can we say that the change in y is 0?

Amrita:

Yes,we can surely say that, Agnishom.

Agnishom:

So, for a small change of x, there is no change in y at all

Thus derivative of f(x) = 7 is 0

Look at the first graph again. Doesn't it have slope 0 everywhere?

Amrita:

Yes as dy=0.

Agnishom:

Also, the derivative of any constant is 0.

Please remember this rule too.

Any queries? May I proceed to the 9x problem?

Amrita:

I am liking ur teaching very much, Agnishom. Thnx 4 taking the trouble of teaching me.

Agnishom:

Thanks

Let us proceed to the next question now.

What is the slope of the straight line y=9x?

Amrita:

Agnishom,I am really feeling very tired now. I will understand it later from u. Bye,gdn8,ttyl.

Day 3

Agnishom:

Let's deal with the other question

Amrita:

Then we know that y=9x.

Agnishom:

What can you say about the slope of the straight line y= 9x?

Amrita:

Slope is same everywhere.

Btw,can u plz tell me the def of slope once again?

Agnishom:

The slope of a curve at any point reffers to the rate of change of y with a very small change in the value of x.

In case, of straight lines which have the equation y = mx + c. m is the slope.

Can you figure out its value?

Amrita:

Is m=9-c/x?

Agnishom:

y = 9x

Or, y = 9x + 0 [Compare this with y = mx + c]

m is just 9

In other words, if we increase the value of x by some amount, the value of y will change by 9 times that amount.

Numerical Example:

If x changes from 1 to 1.000001

y will become 9.000009 from 9

Since the slope is the same everywhere, we can say that the derivative of 9x is 9.

Okay?

Amrita:

And another question,in this graph y is changing with a small change of x. Then the slope should'nt be 0.

What about it?

Agnishom:

The slope isn't 0, the slope is 9.

Amrita:

So the derivative of f(x)=9x is 9, right?

Plz tell me the def of derivative.

Agnishom:

Derivative of a function f(x) reffers to the rate of change of f(x) with respect to a infinitesimal change in x.

It is the same thing as dy/dx. Since, usually we call y = f(x), $\frac{d\;f(x)}{dx}=\frac{dy}{dx}$

Do you get it?

Amrita:

Yes,Then?

Agnishom:

We have seen atleast three things till now:

The derivative of $x^a$ is $ax^{a-1}$ E.g, The derivative of x^3 is 3x^2
The derivative of any constant is 0. E.g, The derivative of 9 is 0.
The derivative of $ax$ is $a$ . E.g, The derivative of 7x is 7

Have we seen them?

Amrita:

Yes,we have seen them.Then?

Agnishom:

Notation: The derivative of f(x) is written as f'(x)

Another Rule: The derivative of af(x) is af'(x)

Proof: Let y = af(x)

With a small change in y, y + dy = af(x+dx)

Subtracting, the first equation from the second: dy = a f(x+dx)-f(x)

Dividing both sides by dx, dy/dx = a (f(x+dx) - f(x))/dx

But, (f(x+dx) - f(x))/dx is the derivative of f(x)

So, dy/dx = a f'(x)

Do you understand the proof? Please remember this rule too. Let me show an example usage of this rule, okay?

Amrita:

Agnishom, I will understand the rest of the Calculus from u later.Now going 2 watch Mahabharat.Bye,gdn8,ttyl.

Day 3

Amrita:

Now plz start teaching me Calculus,if u have time.

Agnishom:

(Please look at the last thing I posted to you)

Amrita:

I was just recapitulating it.Now,u can show this with an example.

Agnishom:

Example: Find the derivative of 9x^2

We can split this into two parts 9 and x^2

Since 9 is a constant we can apply the rule we just discussed.

Thus derivative of 9x^2 = 9(x^2)' = 92x = 18x

Can you find the derivative of 3x^3?

Amrita:

Derivative of 3x^3 is 9x^2.Right?

Agnishom:

Yes, very good.

Let us look at a problem: What is the slope of the tangent to the curve y=x^3 at x=4? Can you find out the equation for the tangent?

Amrita:

I can understand that y=64.Then plz give me a hint.

Agnishom:

Had we discussed that the derivative of a function is the function that desrcibes the slopes of the tangents on that function?

Amrita:

I think we haven't. Or maybe I have forgotten it. Anyway,what's it?

Agnishom:

(Oh, come on! That is the very first idea I started my lectures with)

Anyway, I will explain it once again.

Please find the derivative to x^3 for now.

Amrita:

It's 3x^2.

Agnishom:

The value of 3x^2 at x=4 is ....?

Amrita:

I am confused. What will be the answer?

Agnishom:

Value of 3x^2 with x=4 is 3(4^2)=48

Since 3x^2 is the derivative of x^3, and the value of 3x^2 at x=4 is 48, we can say that the slope of the tangent of x^3 at x=4 is 48.

Do you get what I am saying? If yes, then let's verify it.

Amrita:

Yes,now I got it.

Agnishom:

Now, that was theory. We must check out if that is the real tangent. Lets do an experiment

Please go to http://www.mathsisfun.com/data/function-grapher.php and do the following settings:

Set y = x^3 in one function
Set y = 48*x-128 [Note that this 48 is the slope we got]
Click the Out 10x button to zoom out

You should get something like this:

experiment

Getting that?

Amrita:

I am getting that.

Agnishom:

If you say so....

Some Other Day

Amrita:

Agnishom,u told me that u will show me whether it's a real tangent. Well I have plotted the graph. Then?

Agnishom:

Can you see that we have actually found the tangent?

Amrita:

Yes.

Agnishom:

Do you believe that differential calculus works, now? :)

By the way, here is another rule:

(f+g)' = f' + g'

Example Usage:

Find the derivative of x^2 + 9x

Answer = (x^2)' + (9x)' = 2x + 9

Amrita:

Yes,I understood what u did. Then?

Agnishom:

I was talking about the graph that you plotted. It is a visual proof that calculus works! Do you believe it?

Amrita:

Yes, I believe it completely.

Agnishom:

Thank you.

Situation: the relation between the distance travelled, denoted by x, and the time taken by a body, denoted by t, is given below:

$x=7t^2+5t+8$

Please find out the equation for the velocity of the body

Amrita:

Please give me some hint.

Agnishom:

Instantenous velocity is the derivative of displacement with respect to time.

$v=\frac{dx}{dt}$

Can you differentiate the function in the previous post?

Day after Some Other Day

Agnishom:

By the way, velocity is the rate of increase in displacement with respect to time.

That means it is the derivative of displacement with respect to time. Yes?

Amrita:

Yes, Agnishom.

Agnishom:

This was my equation: x=7t^2+5t+8

Can you differentiate the right side with respect to t?

Amrita:

It's 14t+5,as u told me just now.

Agnishom:

Velocity = dx/dt = 14t+5

If acceleration is defined as the change of velocity with respect to time, please find the acceleration of the body.

Acceleration = dv/dt [where v is velocity]

Amrita:

What's the answer ?

Agnishom:

I suppose it is 14.

Amrita:

dv=14?

Agnishom:

No, dv/dt = 14

Amrita:

How? Please show me

Agnishom:

Acceleration is change of velocity/change of time = dv/dt.

We must compute the derivative of v with respect to t. We have got v = 14t+5

Differentiating 14t+5 with respect to t, we get (14t)'+(5)'=14+0

Do you get this?

Amrita:

Then, dt = ?

Agnishom:

dt is a small part of t. When we are differentiating v with respect to t, it means that we want the ratio of change of v with respect to a very small change of t.

I think you are confused.

Amrita:

No no,it's O.K. Please proceed on.

Agnishom:

Ok, notice something.

We were given that x = 7t^2+5t+8

Differentiating: We get $\frac{dx}{dt}=14t+5$

But again, we call dx/dt as v

We redifferentiate v with respect to t

$\frac{dv}{dt}=14$

But wasn't v = dx/dt?

yeah, So We have actually done this

$\frac{dv}{dt}=\frac{\frac{dx}{dt}}{dt}$

We have differentiated 7t^2+5t+8 two times consecutively.

The result after differentiating twice is called a second derivative. For example, the second derivative of 7t^2+5t+8 is 14

We can also write $\frac{\frac{dx}{dt}}{dt}$ as $\frac{d^2x}{dt^2}$ .

Amrita:

I couldn't understand the last step. Can u plz xplain it 2 me?

Agnishom:

It is just a notation. (Though there is a good reason for it)

One more notation: f'(x) is the first derivative of f(x) f''(x) is the second derivative of f(x) f'''(x) is the third derivative of f(x) and so on

Amrita:

O.k. Then?

Agnishom:

Amrita, Read these rules: http://www.mathsisfun.com/calculus/derivatives-rules.html

---

The conversation continued to the chain rule, partial derivatives, total derivatives, the exponential function, extrema, taylor series, integration and more but as for the introduction to derivatives this is it.

Markdown	Appears as
`italics` or `_italics_`	italics
`bold` or `__bold__`	bold
- bulleted - list	bulleted list
1. numbered 2. list	numbered list
Note: you must add a full line of space before and after lists for them to show up correctly
paragraph 1 paragraph 2	paragraph 1 paragraph 2
`[example link](https://brilliant.org)`	example link
`> This is a quote`	This is a quote
# I indented these lines # 4 spaces, and now they show # up as a code block. print "hello world"	# I indented these lines # 4 spaces, and now they show # up as a code block. print "hello world"

Math	Appears as
Remember to wrap math in `\(` ... `\)` or `\[` ... `\]` to ensure proper formatting.
`2 \times 3`	$2 \times 3$
`2^{34}`	$2^{34}$
`a_{i-1}`	$a_{i-1}$
`\frac{2}{3}`	$\frac{2}{3}$
`\sqrt{2}`	$\sqrt{2}$
`\sum_{i=1}^3`	$\sum_{i=1}^3$
`\sin \theta`	$\sin \theta$
`\boxed{123}`	$\boxed{123}$

A (very) Informal (yet conceptual) Introduction to Derivatives

Acknowledgement

To The Reader

For the Calculus Pro -

For the Calculus Newbie -

Day 1

Agnishom:

Amrita:

Agnishom:

Amrita:

Agnishom:

Amrita:

Agnishom:

Amrita:

Agnishom:

Day 2

Amrita:

Agnishom:

Amrita:

Agnishom:

Amrita:

Agnishom:

Amrita:

Agnishom:

Amrita:

Agnishom:

Amrita:

Agnishom:

Amrita:

Agnishom:

Amrita:

Agnishom:

Amrita:

Agnishom:

Amrita:

Agnishom:

Amrita:

Agnishom:

Amrita:

Agnishom:

Amrita:

Agnishom:

Amrita:

Agnishom:

Amrita:

Agnishom:

Amrita:

Agnishom:

Amrita:

Agnishom:

Amrita:

Agnishom:

Amrita:

Agnishom:

Amrita:

Agnishom:

Amrita:

Agnishom:

Amrita:

Agnishom:

Amrita:

Agnishom:

Amrita:

Day 3

Agnishom:

Amrita:

Agnishom:

Amrita:

Agnishom:

Amrita:

Agnishom:

Amrita:

Agnishom:

Amrita:

Agnishom:

Amrita:

Agnishom:

Amrita:

Agnishom:

Amrita: