CAPACITORS! Need help!

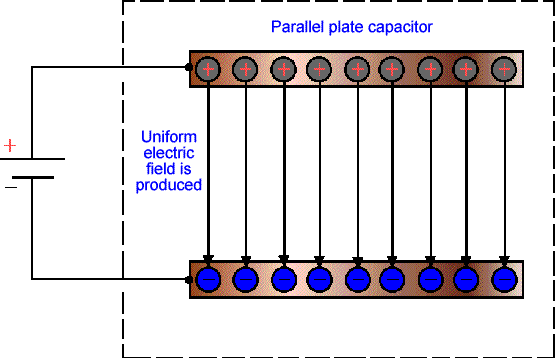
This has been bugging me for days and I really need to move on:

Why do electrons stop flowing when the potential difference between the plates of a capacitor equals the battery's terminal potential?

Explanations like THIS ring many bells, but not the ones I'm looking for:

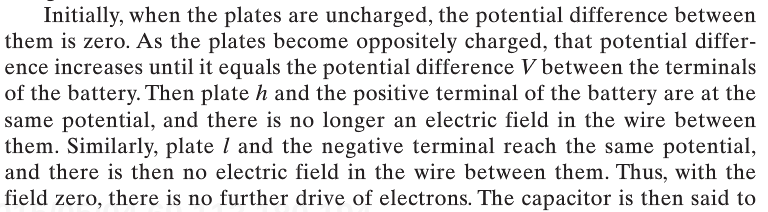
Also, what does it mean for there to be a potential AT a certain object? Like, potential AT THE PLATE and AT THE BATTERY TERMINAL. This makes absolutely no sense to me since voltage is relative. Is it bad wording, and it's implying the potential difference BETWEEN the plate and the terminal? In this case, does it simply mean there's no electric field between the plate and the terminal, and thus no voltage? Still doesn't answer the above question though.

I've tried this animation, but nay.

This, from another book, also won't ring bells:

Why do the charges on the plates have to be exactly equal in magnitude?

I've found this electric potential diagram to be somewhat useful, maybe you can refer to this in your answer:

And, lastly, does the battery supply the electrons to the negative plate or does it simply transfer the electron received from the positive plate to the negative plate? If it's the latter, it explains my previous question.

I really appreciate any help. Thank you!

#ElectricityAndMagnetism #Voltage #Capacitance

Easy Math Editor

This discussion board is a place to discuss our Daily Challenges and the math and science related to those challenges. Explanations are more than just a solution — they should explain the steps and thinking strategies that you used to obtain the solution. Comments should further the discussion of math and science.

When posting on Brilliant:

Use the emojis to react to an explanation, whether you're congratulating a job well done , or just really confused .
Ask specific questions about the challenge or the steps in somebody's explanation. Well-posed questions can add a lot to the discussion, but posting "I don't understand!" doesn't help anyone.
Try to contribute something new to the discussion, whether it is an extension, generalization or other idea related to the challenge.
Stay on topic — we're all here to learn more about math and science, not to hear about your favorite get-rich-quick scheme or current world events.

Markdown	Appears as
`italics` or `_italics_`	italics
`bold` or `__bold__`	bold
- bulleted - list	bulleted list
1. numbered 2. list	numbered list
Note: you must add a full line of space before and after lists for them to show up correctly
paragraph 1 paragraph 2	paragraph 1 paragraph 2
`[example link](https://brilliant.org)`	example link
`> This is a quote`	This is a quote
# I indented these lines # 4 spaces, and now they show # up as a code block. print "hello world"	# I indented these lines # 4 spaces, and now they show # up as a code block. print "hello world"

Math	Appears as
Remember to wrap math in `$` ... `$` or `\[` ... `\]` to ensure proper formatting.
`2 \times 3`	$2 \times 3$
`2^{34}`	$2^{34}$
`a_{i-1}`	$a_{i-1}$
`\frac{2}{3}`	$\frac{2}{3}$
`\sqrt{2}`	$\sqrt{2}$
`\sum_{i=1}^3`	$\sum_{i=1}^3$
`\sin \theta`	$\sin \theta$
`\boxed{123}`	$\boxed{123}$

Comments

In almost every context within the purview of electrostatics, when we speak of potential at a point, the reference point of zero potential is at infinity. So, yes potential at a point without a reference is meaningless and this is just a matter of convenient definition. However, once defined in this manner, there is no ambiguity forthwith and the cited paragraph implicitly/contextually assumes said convention. (Of course you could just ground one point on the circuit and measure potentials with respect to this point but this really is a convention best suited to the study of things like transistors and logic gates.)

And the electrons stop flowing precisely because of a lack of potential difference between the terminal and the plate. Of course that doesn't explain $why$ the potential difference becomes zero, and I'm not sure if that is your actual query.

Also. the battery does not create any charges and is only 'transferring' charges from one side to the other. This is a consequence of the fact that the circuit is a closed one and thus the total charge must be conserved(and was zero to start with).

Indeed, the best possible analogy is to think of the capacitor as a spring and the battery as an agent that's compressing the spring with a $constant$ force. After a while the 'spring' is 'compressed' to the point wherein the restorative force exactly balances the external force.

The battery is kind of like a pump and is pumping electrons instead of water and doesn't create any more 'water'.

Of course, a lot of these analogies might seem like hand-waving because, well...that's precisely what they are. We are 'justified' in invoking them only because the equations governing both scenarios are analogous.

@John Muradeli

Shashwat Shukla - 6 years, 1 month ago

Well explained Shashwat. Capacitance is an interesting topic.

Ronak Agarwal - 6 years, 1 month ago

Thanks :)...And capacitance is especially interesting when things like spheres in an infinite medium are considered. I find such questions very interesting.

Shashwat Shukla - 6 years, 1 month ago

@Shashwat Shukla – Infact we start learning about capacitors by first learning about sphere in infinite mediums,and then learning about parellel plate capacitors.

Ronak Agarwal - 6 years, 1 month ago

Thank you for your explanation, Shashwat. However, I still need help with my main point.

My question is, rephrased, why when the potential difference between the plates equals the potential difference between terminals then there is no potential difference between the plates and their respective terminals?

No potential difference indicates that the net electric field is zero between the wires - hence when we integrate along the wire, the sum is zero and the voltage is zero. Right? I don't think so; we need to be reminded that voltage is not a vector quantity. When working between two point charges, exactly at a distance between the charges, the electric field is zero but the voltage is anything but zero:

We can see this by the diverging electric field lines, indicating that a positive test charge would shoot outwards if placed at that point. We can also see this by conventional means, like bringing one charge in from infinity and then the other charge - clearly measuring a potential difference. But at the midpoint, there still would be a potential difference. However... I think I just realized that the electric field is zero merely AT THAT VERY POINT, and thus that very infinitesimal yields no contribution to the electric potential. So.... I guess the initial hypothesis holds; if the electric field along a path is zero, so is the potential difference due to that path/between start & finish.

So, the net electric field must be zero along the wire. In other words, the charge on the plates has to produce an electric field equal in magnitude but opposite in direction to that produced by the terminal. Now the question is, why are those fields equal in magnitudes when the potential differences between plates and terminals are equal?

Here's a hint: I try to use the capacitance formula for parallel flat plates $(C\sim A/d)$ to confuse myself even more; when we double the distance, the potential difference doubles too; now, we can store half the charge (before reaching the terminal voltage)! Now if we double the area it makes sense to store twice the charge. But basically capacitance measures how much charge we can store until the plates reach the terminal voltage - which is determined by the voltage between the terminals (how punny). I simply do not see the electric field from the wire from, say, the positive plate, becoming any more intense when we double the distance between the plates than when they were at the original distance of separation. Maybe I'm wrong in this respect?

And please refrain from using the water tank or whatever analogy; they are all so wrong in so many ways.

Thanks a lot!

John M. - 6 years, 1 month ago

Why do electrons stop flowing when the potential difference between the plates of a capacitor equals the battery's terminal potential?

why when the potential difference between the plates equals the potential difference between terminals then there is no potential difference between the plates and their respective terminals?

Let $A =$ "the potential difference between the plates equals the potential difference between terminals", $B =$ "there is no potential difference between the plates and their respective terminals", and $C =$ "electrons stop flowing."

Then note that $B$ implies $A$ ; $B$ implies $C$ but $A$ does not imply $B$ . The proof of the first and second statement is obvious. For the third, you can take the potentials w.r.t. infinity to be $5V$ ; $-5V$ at the plates and $20V$ and $10V$ at the terminals. Here, $A$ holds, but $B$ does not.

Now, we know that $B$ will occur. This is because if there is a potential difference between the plates and the terminals, then current will flow to neutralize the potential difference. Since $B \implies A$ , and $B \implies C$ that means that $A$ and $C$ will also occur.

TL;DR $A$ does not cause $C$ , but both of them are caused by the occurrence of $B$ .

Now the question is, why are those fields equal in magnitudes ... I try to use the capacitance formula for parallel flat plates to confuse myself even more; when we double the distance, the potential difference doubles too; now, we can store half the charge

This is my guess and probably incorrect, but here's why. First off, remove the invariants. The battery doesn't change so remove it. So now the question is why does the electric field stay constant when we

halve the charge and double the distance.

Since the plates remain the same, we can approximate them as points without adversely affecting calculations and treat the capacitor as a dipole. Since $E$ is proportional to $Q.d$ in a dipole, we can see that the electric field stays constant.

double the charge and double the area.

We can't do the same as before, since the plates change. But, since the positions of the plate don't change here, we can use the fact that the electric field due to a sheet is proportional to $\sigma$ when everything else is invariant. This would explain why the electric field is constant.

I know my answer is very sketchy and hand-wavy, but I hope you can understand the gist of it.

Siddhartha Srivastava - 6 years, 1 month ago

@Siddhartha Srivastava – Srivastava to the rescue!

Alright so you made lots of good points that made me discover some of the things on my own, but few questions remain. Let me use this diagram for reference:

"Since is proportional to in a dipole, we can see that the electric field stays constant."

No. In fact, the electric field between the plates remains approximately constant as long as the width of plates is much greater than distance of separation between them - as seen from image above. The electric field then is only proportional to charge density - and you could argue then just charge, since area remains constant and charge increases and distributes itself uniformly about the plate (hence charge/area). So the expression for E is

$E=\frac{\sigma}{2 \varepsilon_{0}}$

Now, let me interpret voltage as I see it, real quick. So, if you take a test charge and put it in a field and you measure the voltage of that test charge with respect to two different charge sources, then, which ever source yields a greater voltage, the (negative) test charge will be attracted to it. Why? Well, the way I see it, voltage simply tells me which source has a greater electric field distribution in space with respect to the test charge of interest. So, for simplest example, if we place a positive test charge between to infinite positive plates, the one on the right which has, say, $10V$ , will repel the positive test charge toward the left plate which has $5V$ - because the NET ELECTRIC FIELD - or, the NET FORCE PER CHARGE is directed LEFT. Correct? Now with infinite sheets you could simply measure each plate's voltage from infinity, but, it won't work with variable electric fields (say, point charges) - which is why I measure the voltage with respect to the TEST CHARGE.

So, now, how the heck do you determine an electric field along the wires? "It's a complicated task, and since the voltages are the same at terminals and their respective plates, we can conclude the net electric field is zero." Really? Well, first let me point out the major problem I have with that logic. Yes, it seems natural that if voltages at respective plates and terminals are equal, no charge should flow. But... we forgot how we determined the voltages in the first place.

So you demonstrated that if potential difference between plates and terminals is the same ( $A$ ), that does not necessarily imply that $B$ will also hold. But, how did you measure the $10V$ , $20V$ , $-5V$ , and $5V$ in the first place?? Now you cannot simply bring in a point charge from infinity from any direction because electric field distribution is not uniform! So, the only hope you have of measuring a "potential difference" is to do it between the plates. THAT'S IT! Between the plates! You can't know anything absolutely! What I mean is, you can't just pick a random distance from a plate and say AH-HA! YOUR VOLTAGE IS 8!

So now, the halving distance problem becomes way more... problematic. Yeah - let's double the distance. Now the potential difference between the plates is twice what it used to be. Does it mean the electric field between the plates and their respective terminals just DOUBLED?? Tough stuff, eh?

Well, typing all this (probably already almost an hour since I saw you post it 6 minutes after your original post), I've thought about the last diagram I posted in the original note carefully. Now I'll go painting a little bit so bear with me here - but I hope ... loll... I think I should move on haha. Gotta do 20 weeks of physics by Monday morning (AP exam). I'll be back with the painting and IM OUTA HERE!

Thanks a lot though - don't think I don't appreciate your help. It's VITAL to my survival!

John M. - 6 years, 1 month ago

@John M. –

No. In fact, the electric field between the plates ... So the expression for E is

I was talkng about the electric field outside the plates, not between the plates. I thought you meant the same thing since you were talking about wires. The only wires are outside the capacitor.

Now, let me interpret voltage as I see it, real quick ......TEST CHARGE.

Not nessecarily true.Take a very long pipe with one end near you and another far away from you. Now place a positive charge near your end. The potential increases at this point. Now, if you place a positive test charge here, we would expect the test charge to fly away to the lower potential (other end). So far so good.

Now, place a huge positive charge at the middle of the pipe. Now, by symmetry, the potential difference at both ends is unchanged, i.e, it is greater at your end. But, if we place a test charge at your end now, it'll still stay at your end, since it can't cross due to the large charge, even though it is at a spot with a higher potential. Which contradicts what you said. A test charge does not nessecarily always go from a higher potential to a lower potential.

So you demonstrated that if potential difference between plates and terminals is the same (), that does not necessarily imply that will also hold. But, how did you measure ... What I mean is, you can't just pick a random distance from a plate and say AH-HA! YOUR VOLTAGE IS 8!

Basically, what potential difference signifies is the net amount of work down to bring a positive unit charge from one point to another. When we don't specify the starting point, we are talking about the net work done to bring a positive unit charge from infinity. This is pretty easy to measure. It doesn't matter if the electric field is non-uniform. We just want to measure how much work is done to bring the charge to that point from infinity.

So if I say that point A has a pot. of $5V$ and B has a pot. of $10V$ it means it takes $5J$ to bring a unit charge to A from infinity and $10J$ to bring a unit charge to B from infinity . Now the great thing is that Electrostatic force is a conservative force. That means that work done by it is not path-dependant. So we can take any path. If we take a path of infinity -> A -> B, we get that $W_{\infty \rightarrow A} + W_{A \rightarrow B} = W_{\infty \rightarrow B}$ or $V_{\infty \rightarrow A} + V_{A \rightarrow B} = V_{\infty \rightarrow B}$ or $V_{\infty \rightarrow B} - V_{\infty \rightarrow A} = V_{A \rightarrow B}$ .

So the potential difference between A and B is just the difference of potentials of A and B when measured from the same point. So talking in terms of potential from infinity is much easier then specifying the pot. diff. between every pair of points.

Alright so here's my idea: ... I mean, I can only think about the voltage between the battery's terminals - but I don't know how that applies to the entire circuit!

Your reasoning is correct. A lower electric field would imply a lower current. But that is not possible, as that would imply there is a buildup of charge somewhere in the wire, which is not possible.

Also, voltage is the potential difference between two points. That's it. So when you connect them with a wire, the "voltage" of the circuit is just the potential difference between the terminals of the battery.

Siddhartha Srivastava - 6 years, 1 month ago

@Siddhartha Srivastava – Hm... so, I fail to understand your pipe example. Where are we putting this test charge, exactly? Is the pipe hollow? Do we place it inside? Then isn't net electric field inside zero (OH YOU BET)? So that's not it. Could you elaborate please?

So, you're using the fact that electricity is a conservative force to state that "when you connect them with a wire, the "voltage" of the circuit is just the potential difference between the terminals of the battery." But... what...? Uhh. Okay, that's fine.

So... let me get this straight. Assume we have $14V$ at the plate. Assume we have $15V$ at the battery. I have two questions:

(1) - How would you measure the respective voltages, exactly? From infinity? If that's so, are they still relevant to each other through the wire?

(2) - There's a potential difference of $1V$ . Now what causes a negative test charge (or just a charge) to flow from the $14V$ plate to the $15V$ terminal? Well, the answer largely depends on how you answer (1).

So, if you answer those two (and the voltage pipe example), it'll all fall in place. ALL OF IT.

(P.S. I hope you saw my reply to myself with the battery diagram).

John M. - 6 years, 1 month ago

@John M. – Image

So pipe in green. The big circle is the large charge. Small circle is the small charge. And the X is where the test charge is placed. Kind of obvious that even though there is a potential difference between two ends of the pipes, the charge won't flow, due to the large charge.

(1) - How would you measure the respective voltages, exactly? From infinity? If that's so, are they still relevant to each other through the wire?

Theoretically or practically? When we make theoretical calculations, we usually measure the potential difference w.r.t. to infinity. You just integrate the electric field w.r.t. displacement from infinity to that point.

Practically, we can't do that, so we measure its potential w.r.t to the ground with the help of a voltmeter.

Also, just to clear this up, calculating w.r.t to a fixed point ( infinity, ground), is just a matter of convention.We assume that the potential of a point at infinity is zero. Thus, whenever we calculate the potential difference between two points, we can find the potential of that point. Kind of like how we don't a absolute point of reference in space but assume a fixed point of reference for ease of calculations.

And by " are they still relevant" do you mean that if we measure the potential of point A w.r.t. infinity to be 14V and potential of B w.r.t. infinity to be 15V, then is the potential difference between A and B 1V? Yes.

I'm just blatantly copy-pasting the relevant part of my original reply.

So if I say that point A has a pot. of $14V$ and B has a pot. of $15V$ it means it takes $14J$ to bring a unit charge to A from infinity and $15J$ to bring a unit charge to B from infinity . Now Electrostatic force is a conservative force. That means that work done by it is not path-dependant. So we can take any path. If we take a path of "point at infinity" -> A -> B, we get that $W_{\infty \rightarrow A} + W_{A \rightarrow B} = W_{\infty \rightarrow B}$ or $V_{\infty \rightarrow A} + V_{A \rightarrow B} = V_{\infty \rightarrow B}$ or

$V_{\infty \rightarrow B} - V_{\infty \rightarrow A} = V_{A \rightarrow B}$ . Putting the values, we can see that $V_{A \rightarrow B} = 1V$ , which is what we wanted to prove.

(2) - There's a potential difference of 1V. Now what causes a negative test charge (or just a charge) to flow from the 15V plate to the 14 V terminal? Well, the answer largely depends on how you answer (1).

A potential difference does not necessarily mean that charge will flow. You can see that in a pipe example. Or even simply, just look at a single battery. There is a potential difference between the terminals of a battery. So why doesn't charge flow through the battery itself, even when it's not connected with a wire?

I'll leave that for now and answer your main question. Why does charge usually flow when there is a potential difference?

WLOG, I'll just talk about positive potentials. Charge flows from higher pot. to lower pot. What does "higher pot." mean? It means that it took more work to bring a unit charge to that point. Why? Because the repulsive (electrostatic) forces were larger. This difference in repulsive force causes the particle to be pushed towards the point which has lower potential.

But this requires the points to be adjacent to each other. If the points are not adjacent to each other, like in the examples I gave you, there is a chance that there exists a point of higher potential between them which the charge can't move through.

Also, this explains why there is not electric field between the terminal and the plates. It is not because the electric field due to terminal and plate cancel out, it is because the electric field by both of them are negligible/zero.

So what causes current to flow? The small potential difference between two adjacent points in the wire. A wire initially has zero potential at all its points. When it's connected to the terminal, there is a pot. diff. which causes charge to flow to the first point. This point now is at a higher pot. which causes the charge on it to move forward again and this process repeats till the charge reaches the other terminal.

Siddhartha Srivastava - 6 years, 1 month ago

@Siddhartha Srivastava – AN AHEAD NOTE:

This got complicated REAL fast... Umm... yeah I'm probably wasting too much of your time - and my time. Maybe I should come back to this a bit later. Yeah. For now I'll just take it for granted and move on. But I will read anything you write - if you choose to write so.

You just love flipping my view on the universe upside down, don't you? Woah. Alright. That explained a lot... and... brought up a lot too...

So first off, I don't understand the pipe example. Is the test charge positive? Why are the two end-charges outside the pipe? How do they set up a voltage inside the pipe? Isn't electric current zero inside the pipe? It would seem more convenient to place the two charges inside the pipe and just enlarge the pipe. But then I still would have no clue why the test charge would not flow - there is a clear potential difference.

"This difference in repulsive force causes the particle to be pushed towards the point which has lower potential."

Meaning, towards the area with weaker electric fields. Or, the net electric field dominates at a region with a higher potential. Right?

However, like I said, for me, potential for current only makes sense when you calculate potentials with respect to a test charge. Then you calculate the test charge's potential. Say, a plate's potential is 100V - but it's really far. And then there's a 10V. And we have a negative test charge. If the 10V is closer, I would expect it to go towards it, not the 100V source. And this, assuming we calculate each source's potential from infinity. Now, if we calculate potential when setting the position of the test charge to be the point of zero potential, the 10V source will get more (let's just assume). Thus the electron will flow to the higher potential source - with respect to itself.

Now my problem with the capacitor - yes, the plate has its own potential and so does the terminal. But if we were to place a negative test charge somewhere along the wire between positive plate and terminal - would we still expect it to flow to the higher potential? If so, there must be an electric field (since motion is caused by force - electric force per charge.) But you said the most controversial statement so far:

" It is not because the electric field due to terminal and plate cancel out, it is because the electric field by both of them are negligible/zero."

IS THAT SO?? So... Umm... What about this diagram?

Is an electric field not set up throughout the wire? In fact, according to that large blob of text above the diagram, it sure is!

If we connected our wire full of conductive copper atoms to the battery, that electric field will influence the negatively-charged free electrons in the copper atoms. Simultaneously pushed by the negative terminal and pulled by the positive terminal, the electrons in the copper will move from atom to atom creating the flow of charge.

According to this list of misconceptions,

charge barely flows.

Now now now... What you're saying is, when connected to a terminal, there's a slight induction of electric charge that sets up an adjacent electric field - causing a slight flow due to the high potential. Then the electrons will continue flowing through the neutral (which, relative to the path behind, is a LOWER POTENTIAL) wire until it reaches the other terminal? Buuut... how does this work with the capacitors, whose initial flow from the positive plate is caused by the positive terminal? This entire idea either contradicts every text above, or I am strongly misinterpreting it.

To be straight, the only best way to communicate to me the idea of a potential is through the integration of the electric field through a distance - say, along the wire. You know - that formula: V equals negative integral of E dotted with ds. The "E" is probably the trickiest part.

I mean, just the idea that by simply having an electric potential difference between two terminals, then the electric fields generated by the charge concentrations on the two terminals will set up that very potential difference across the entire wire no matter the shape or length of the wire. FANTASTIC!

And look at what I found! Read that last box:

Now THAT... just... made me confused to infinity.

Again, thanks a lot for all the replies.

John M. - 6 years, 1 month ago

@John M. – It's three in the morning, so I'll only address the text. I can't believe that is a physics text book. How could they actually try to clear a misconception by simply stating "This simply isn't true", without stating any reason why it is not true. Ugh.

Anyways. The book's diagram on the battery's electric field seems to be horribly wrong. For example,If I connect the wire in the form of an "S", it would imply that current is going in opposite directions in different parts of the wire. Also, a more ridiculous implication would be that if I place two batteries opposite to each other such that the field cancel out, and connect a wire to only one of them, then there would be no flow of current. I could also increase or decrease the current flowing through a wire just by bringing a battery in its vicinity.

Also, for the pipe thing, the test charge is positive. I placed the other charges outside the pipe to avoid any collisions. And if you ignore the higher potential to lower potential thing,you can see by coulombs law that the massive like charge will repel the test charge and not allow it to pass through the pipe.

Siddhartha Srivastava - 6 years, 1 month ago

@Siddhartha Srivastava – Woah 3 AM you're so hardcore

Alright so, this (and the rest of the stuff, you know, in the previous comment) is for when you wake up:

I really don't think that diagram is horribly wrong. After all, the electric field lines are nearly identical to that of a dipole - we may treat the $(+)$ terminal and the $(-)$ terminal as point charges.

And I don't think there's any feasible orientation in by which you could bring in another battery to "cancel" that electric field. I mean, if you, say, line up opposite terminals, it will simply straighten the electric field lines. If you line them up sign to sign, they electric field lines will diverge. Now... when you connect a wire... dunno. But it does make sense that the wire would somewhat divert the electric field line - because there are free charges in the wire and they partially may set up or re-direct the electric field along their path. Now maybe this works only when the wire is conveniently set up, I don't know, but surely a zig-zagged wire or an S-shape like you said would not have the same degree of electric field line-up. In fact, this article elaborates on that issue.

And the pipe thing - well I think the test charge would surely move a certain distance towards the larger charge - but obviously not past it. But that could be justified with voltage - that is, there is some point between the two test charges at which the potential difference between the test charge and the two charges are equal. Well of course this doesn't have to be true if the large charge is overwhelmingly larger, in which case the test charge would shoot out of the other side (towards no potential), but point is taken. Right?

John M. - 6 years, 1 month ago

@John M. – Okay. I finally kind of understand what's going on and how to explain it. Huge wall of text. Maybe you can read it after the AP test.

Imgur Imgur

First. The pipe example. What I'm trying to say here is that using test charges to measure potential is not always possible. In the pipe example, we can see that A has more potential than B. But if we place a positive test charge at A, then the test charge won't move to B. Why? Because of the large positive charge in the middle. But if the test charge does not move, that would imply that A is a t a lower potential than B, which is mathematically not true.

Why does this happen? Because we were looking at only two points far away from each other. Look at a third point in the middle called C. Clearly this has a huge potential, larger than both the potential at A or B. A large amount of work will have to be done by the test charge to move from A to C. But on the other hand, a large amount of work will be done on the test charge to take it from B to C, which cancels a lot of the work done by the charge. In the end, by the definition of potential, we see that the net work done on it in this path is $> 0$ . So why does the test charge not move from A to B? Because it will attempt to, but there is no way for it to get to C, since getting to C requires lots of work/energy which the test charge do not have. And the only way to get to B is through C. Therefore, the test charge will not go from A to B, even thought there is a potential difference.

Since the test charge has no initial energy, it can never go to a point which has a higher pot. since that would imply the charge had to do work to get there, which is impossible, since the test charge has no energy. So if all paths from the point A of higher pot. to point B lower pot. involve going through a point which has a higher pot. than both those points, then the test charge will not be able to go from A to B, even though there is a pot. diff.

And it's not like this is a very rare thing. This is the reason charge does not flow through a lone battery/capacitor even though there is a pot. diff.. The air and the interior of the battery both are at a higher pot, which prevents current from flowing. And when we add a wire, we are doing nothing but providing the terminals a path where no point has a higher pot. than the positive terminal, which is why current flows.

Second. The diagram. In hindsight it's not that bad, but it's still shouldn't be in a physics text. The diagram implies that the electric field from the terminals of the battery is responsible for the flow of current. That is to a certain extent correct. I can't find out any reliable numbers about how strong the electric field is, but that doesn't really matter. The problem is that anyone who sees the diagram is going to infer that current flows solely due to the electric field of the terminals, which is wrong. The article you gave points out why. The electrons in the wire also play a role, and a significant one at that in some parts of the wire.

Third. Capacitors. To recap, when we connect a discharged capacitor (just two plates) to a battery, there exists a potential difference between the terminal and the respective plate and a path for the charge to flow through (wire), so charge flows to neutralize this potential difference. So eventually, we see that the terminal, plate, and wire connecting them to be at the same potential.

Now your problem was that this implies the electric field along the wire is zero at all points. This in turn, would mean that the field from the capacitor and battery is equal at all points.

The fact that the electric field is zero at all points is true. This can seen from the fact that all the points of the wire are at the same potential. Or more simply, if there was a electric field, current would flow to remove this field.

What is not true is that the field from the capacitor and battery must be equal. What was forgotten was that a third entity can also exert a electric field, namely the electrons in the wire. So this build up or absence of electrons neutralizes any electric field caused the battery and capacitor. Also, this implies that changing the properties of the capacitor (Q,A,d), does not matter as long as the voltage between the plates remains the same. Any change will be compensated by electrons in the wire.

The only problem that comes here is that this would mean that plates of the capacitors need not have the same charge. But the difference should be negligible. And when we talk about an ideal circuit, we assume that the battery/capacitor don't exert any electric field, so in an ideal circuit, the capacitor has the same charge on both plates.

Also, for that last example, there is no problem. It is describing how current flows in general. It is not electrons meandering their way from the terminal all the way to the plate. The flow of electrons is really just the electrons moving a bit due to an electric field. At the terminal of the battery, the electric field is from battery. After that, a major part of the electric field is from the electrons behind it.

Kind of like Newton's cradle.

Siddhartha Srivastava - 6 years, 1 month ago

@Siddhartha Srivastava – So basically... I've been presented an overly simplistic perspective on this issue and my depth of scrutiny demands far more explanation than the designed course intends to convey. So the wire-capacitor-battery system makes things work out by self-balancing mechanisms that are more than simply the electric fields set up by the terminal and the plates. However, I'd like to clarify a few things for closing:

Pipe. Oh the pipe. So, like I said, potential differences have no meaning for me unless they are "relevant" - that is, when measuring differences across two points, one must know what's going on BETWEEN the points - not necessarily the exact electric fields, but approximate potential distributions. If we place the pipe-charges system in a 2D plane and map out a 'potential field' describing voltage distributions, we would clearly see one of two possible scenarios (as I've stated before): $(1)$ There is a point between the two source charges with respect to which the potential of the test charge is the same. This implies that the particle would stop at that point and be trapped between the two charges - progressing not much further along the pipe other than by basic quantum motion - unless influenced by an external agent. I'll get back to this one. $(2)$ The potential set up by the large charge dominates the potential set up by the smaller charge and hence causes the test charge to shoot out of the pipe from the smaller source charge's side (if it's positive).

Now, $(1)$ is an excellent example of my yet-persisting problem with capacitors; we double the distance of separation between two parallel flat capacitor plates. The potential difference between them doubles. SO WHAT? Why is this supposed to have any effect on the charge flow, from electric fields perspective? I mean, after all, this voltage is purely determined from finding the potential difference BETWEEN the plates!

Now I suppose one may argue that since the charges on both plates is equal in magnitude (which I will scrutinize shortly), they will each produce equal and opposite electric fields - and hence the contribution to the potential difference between the plates is equal from each source. So, if we have $12V$ , one must have $-6V$ and the other $6V$ . No? Well, I think no - what if the potential at the terminals is $6V$ and $18V$ ? Boo-yah... NOW WHAT??

Besides, the relativity issue persists: what does the potential derived from work done on a test charge along a path between the plates have anything to do with potential derived from work done on a test charge along a path set up by the wires?!?!?! NONSENSE!!

Now, I surely can make sense of your "charge will flow till potential equalizes) concept, but I simply cannot visualize the condition of the system when in the process of charging, or fully charged. So I can only say "alright the capacitor will work its magic - and the details aren't important."

At the moment a more practically-pressing question is regarding the last paragraph of the last diagram:

So... now the charge is due to electrons moving in the capacitor?! So, this surely makes sense in the 'lighting-the-bulb' example - electrons already in the bulb oscillate due to the electric field set up by the battery, but... Hold on. What happened to electrons being pulled out of the positive plate and migrating to the negative plate?? And then that same book argues the following for series capacitors; it starts out on previous page "When the battery is first connected to the series of capacitors, it...

Is "moving the charge" implying the upper plate of the bottom capacitor sets up an electric field that influences the bottom plate of the capacitor above it? My head is ready to implode.

John M. - 6 years, 1 month ago

@John M. –

So basically... I've been presented an overly simplistic perspective on this issue and my depth of scrutiny demands far more explanation than the designed course intends to convey.

That accurately describes anything you are ever taught in school. xD

that is, when measuring differences across two points, one must know what's going on BETWEEN the points

Therein lies the problem. You don't always know what is going on between the points. And you don't necessarily want to know at all times either. You might be just concerned with only those two points. Your definition makes sense intuitively, but is severely hindered as it only works in a few select scenarios. OTOH, by using the work definition, we don't need to know anything about what is going on between the points.

In the end, this is a compromise you'll have to make. Either intuitively understand your definition but be limited by its scope, or use a non intuitive definition but be able to use it in more cases. This is also why people use analogies. To be able to understand the non-intuitive definition to some extent.

Why is this supposed to have any effect on the charge flow, from electric fields perspective? I mean, after all, this voltage is purely determined from finding the potential difference BETWEEN the plates!

The effect on charge is not caused by the change in electric field of the capacitor. The change of the electric field is immaterial. Any change in the electric field caused by the capacitor will be compensated by the electrons in the wire.

What does influence the charge is the potential difference between the plates. And when we increase the distance between the points, we are inevitably increasing the potential diff. between the plates (The test particle will have to travel double the distance. in the same field, thus double the work.). To compensate for this increase in potential diff., we have to decrease the charge on the plates.

Likewise for area. When we change the area, we change the surface charge density on the plates, which results in a change in electric field between the plates.

Well, I think no - what if the potential at the terminals is 6V and 18V?

There was an assumption when we started talking about capacitors. That there is not net charge in the (ideal) system. This is why we see $\pm Q$ charge on the plates of the capacitors.

But look at your battery. There is a positive potential on both of the terminals. This implies there is a positive charge on both of your terminals. There must be a net charge on the battery.So when we connect this battery to the system, there will be a net charge on our system, which means that our initial assumption holds false.

what does the potential derived from work done on a test charge along a path between the plates have anything to do with potential derived from work done on a test charge along a path set up by the wires

This is where the fact that electrostatic force is a conservative force comes into play.If the only forces acting on a body are conservative, It doesn't matter how we go from point A to point B, the work done is path indepenndant. Some MIT lecture notes on why electrostatic force is conservative. This means when I'm talking about the potential between two points, I don't have to specify the path taken, because the work done along all paths will be identical. The path could be the line joining the two points or a path which goes through Mt. Everest. Doesn't matter. Both paths will give the same work.

practically-pressing question is regarding the last paragraph of the last diagram:

I did mention this in my last comment. Anyways, when we talk about capacitors, we sometimes say the charge moves from one plate to another. What some people might infer is that we literally take electrons from one plate and move the same electron to the other plate. That inference is what the book is calling wrong. The charge on the plates is caused by slightly pushing electrons near the plate towards the plate in the case of the negatively charged plate and pushing away electrons from the positive plate. This pushing is done by the electric field.

Siddhartha Srivastava - 6 years, 1 month ago

@Siddhartha Srivastava – AHEAD NOTE

COLOSSAL BODY OF TEXT AHEAD!!! Spent two hours typing, drawing, thinking. Please proceed on your own time/mental capacity.

For some reason texts either feel the need to stretch their explanations more than they need to be or not explain a concept at all. With the conservative force - the way I see it, and the way a text or a lecturer has NEVER told me to see it as, is as follows:

So, I see that, say, if I move from A to B through the green line, and work that has been done on me that is not parallel to a vector stretching from A to B will be cancelled and yield a net-work of zero along any other vector component. With the green path, we can perceive A to B to be vertical and the arc formed to be the result of a horizontal left-ward work done - until the curve rounds up on the arc - in which case now the work is being done rightward. But if we use the superposition principle and analyze the vertical and horizontal paths separately, we will see that horizontally we got nowhere (hence $F \cdot d = F (d - d ) = 0$ and no net work done). But vertically we clearly moved a distance - and hence work has been done along that path. Same could be argued for the blue path. It's all relative.

Right? Correct me if I'm wrong on that one. And to make a stricter note, that work is path-independent only due to the source of the conservative force. So if we slide a ball across a rigid floor, we can't say that the total work done will be the same if we take the blue path because now air resistance, friction, and cosmic rays are involved - which are not conservative. And this works because the magnitude of the conservative force is conserved throughout the path - on average. And this may be the only hole in my reasoning - what if, say, we wanted to move a positive test charge from left to right of this picture:

Now say when we get really close to the charge, we take the bottom curve, and do not get back up until all the way at the end. Is net work still zero? The net distance is zero, but the average force applied in bringing down the particle through the same displacement is far greater than the force-distance product in bringing the particle back up. So, more work has been done by the electric field in bringing the particle down than up due to the fact that the force field is unevenly distributed. Now the only way I have of re-interpreting this is: the work done on a particle along a defined axis is independent of path taken to get from one point to another on that axis. Then clearly as long as we get from A to B we will have only one net displacement $d$ - though we are not guaranteed that no net work has been done along any other axis we chose to neglect. Right?

Woah I typed a lot for that subject matter. Moving on.

And... oooh... ?? O REALLY?! THOSE ANNOYING DIAGRAMS SHOWING ELECTRONS CLEARLY FLOWING ONE BY ONE FROM ONE PLATE TO ANOTHER - I GUESS THEY'RE ALL BULL$@%& HUH?? Okay! Then THIS model should make more sense:

-except you apply this exact idea to plates themselves. Like this:

Now, with your answer to

"what does the potential derived from work done on a test charge along a path between the plates have anything to do with potential derived from work done on a test charge along a path set up by the wires"

Well yes, conservative force. But, I can surely tell you that if, for example, in the charge near the ground in a diagram above example, the test charge could not simply take such a curve only due to the influence of the field. By conservative force we say that the work done by the field along a defined axis is independent of path taken to get from one point on that axis to another. But to take any sort of path, most likely an external agent will have to do work to direct the particle along that path - in the exception that the test charge moves exactly parallel to an electric field line generated by a source charge. So once again, I don't know how a potential difference derived from moving a test charge between the terminals relate to a potential difference derived from moving a test charge between the plates. Here's my main problem:

I can only make sense of potentials with electric fields - or average electric fields - around a test charge. So, while there may be a potential difference between the plates, that is due to the electric field generated between the terminals - same goes to the plates. But, the electric fields are anything but radially outward - as with a point charge. From what I know, the total electric field contribution of a plate to its respective wire is much less than its electric field contribution to another plate since much less charge is concentrated near the wire than farther away from it. So, I don't know how charges on plates influence the electric fields within their respective wires. But then you may say we need not know the complicated details of electric fields between two points - as long as we know potentials at those points. But then again - the potentials derived seem irrelevant. Here let me draw an ultimate visual I have in my head:

Took forever to draw. I tried to duplicate the following electric fields for the plates on the left:

So, what you're trying to say is, it makes no difference if I take a test charge and move it from infinity along whatever path I desire to the positive test plate, then repeat for the negative test plate, and the potential difference would be the same if I moved a test charge from the negative plate to the positive plate?? Even though the electric field line distributions are totally different in the two scenarios? And - if I repeat the same for the battery - and the potentials for terminals match that of plates - then we can state that there is no potential difference between a terminal and its respective plate?? And you say when we double the distance we double potential - hence we store twice less charge because the potentials at plates match those of terminals for twice less charge?? Nope. Lost it all.

In fact, maybe if you explain to me a much simpler question, it will make more sense:

Say potential difference between the terminals is $12V$ . Now: Why will a concentration of positive test charges of magnitude $1C$ at the terminal have 12J of energy with motion directed from the positive terminal to the negative terminal through the wire?

The only way I make sense of this is if wire is in a straight line:

Then the electric field stretches nicely from positive to negative and we have a well-defined potential. But, if a wire is curved, takes a U-turn, or is simply not straight, I cannot see this happening - especially when it takes a $360$ degree turn. Like with all batteries ever. So...ooOOOooo wait... Your Newton's craddle... Are you going to point me back to this?:

"So what causes current to flow? The small potential difference between two adjacent points in the wire. A wire initially has zero potential at all its points. When it's connected to the terminal, there is a pot. diff. which causes charge to flow to the first point. This point now is at a higher pot. which causes the charge on it to move forward again and this process repeats till the charge reaches the other terminal."

Sort of like this:

Where blue dots represent polarized atoms (read below).

So... the terminal generates a potential, say -6V, and generates an electric field at the adjacent wire atomic lattice, which changes the polarity of the atoms in the fashion shown in diagrams above, influencing the next lattice to repeat the process - essentially inducing an electric field across the wire - until this alignment terminates at the 6V terminal. Or, rather, this process starts simultaneously at both terminals and terminates somewhere mid-day along the wire. But then... what is the meaning of, say, 12J per 1C? If electrons aren't really flowing, then, is it implied that this energy is transferred to the energy sink? Ex. the bulb, or, the capacitor? Well if so, it still does not explain the discrepancy of how we can consider the plate and its respective terminal to be at the same potential from the ambiguity of calculating the plate and terminal potentials in the first place. It seems that the potential DIFFERENCES are easy to calculate - differences BETWEEN the sources (plates, terminals), but not individual potentials in all directions. Basically, my point is, the potential differences between plates and terminals tell us that if we place a positive test charge at the positive plate, it will acquire, say, 12J of energy per 1C of charge by the time it reaches the other end - and, the same will happen for the battery. However, now for the battery it's more ambiguous because a test charge wont move through the battery - rather along and around the wire it links. But it doesn't tell me anything about what happens if I place a test charge somewhere along the wire. But then it's even worse because we confirmed it's not the test charges that move through the wire but it's the electric field. Now, the only thing that makes sense to me is to forget about CURRENT FLOW permanently and only think in terms of charge collecting on plates as a mere CONSEQUENCE or a RESULT rather than an active agent in the process of ceasing the charging of plates. Then, I can imagine the battery attempting to influence the electric field along the wire and between the plates - until a common electric field strength has been established along the entire circuit. This would yield because the electrons in the atoms would continue to be aligned (polarized) due to the presence of a stronger inducer in the previous lattice until it's as polarized as the lattice before it. And so on throughout the entire circuit. And this polarization would also continue through the plates - as illustrated by that "last diagram last paragraph" example. Polarization will continue until the alighnments produce respective surface charges that generate an electric field similar in strength to that of the wire - hence, since this field is approx. uniform, doubling the distance will not affect the electric field. And then the induction ceases at the other terminal. Now, you will say, I should replace the word "electric field" with "electric potential." But I simply can't. Here's probably the best - number one reason why. Look:

This is the 'double the distance' issue. Yeah, we have twice the potential - but the force per charge of each plate is the same. So, the polarization across the other plate should be the same. Moreover, if you could hypothetically imagine the following scenario:

Now, if you take bring a negative test charge from infinity to that blue point between those plates, you get twice the potential with respect to the left plate vs. the right plate. So, is the negative test charge going to shoot on towards the left plate? I DON'T THINK SO!!! THIS is my issue with doubling the distance! So what if the potential is doubled - the charges are just as attracted, repelled, or polarized regaradless of the potential! Potential tells me how much energy a coulomb of charge would acquire if we were to place it at that location and released it - not how polarized charges would get on the sources (plates). AAAAAAAAAAH!!!

John M. - 6 years, 1 month ago

@John M. – So first, conservative forces.

Your initial reasoning holds because you implicitly assume a rectillinear field, i.e. a field where all the field lines are parallel. However you can not apply rectilinear reasoning to a radial field.

In the radial field, instead of moving horizontally and vertically, we move away from the fixed point and around the fixed point. See polar coordinates. So just like you broke up the path into horizontal motion and vertical motion, we break the path into rotation and movement away from the point. Any rotation produces no work, so only movement away from the point produces work.

Second, Polarization is kind of a different topic, so don't assume we're talking about polarization here. Polarization occurs when there is a positive charge holder (proton) and negative charge holder(electron) together,for example in an atom. When an electric field is applied, one charge is attracted, the other is repelled, creating a dipole. The two charges however, are still very much attached to each other.

Electrons, on the other hand are relatvely free to move around. An indivivual electron, however, won't move very far or very fast.You can see "drift velocity" and see it is quite slow. But the "current" moves fast. This disperancy means that current is not just indiviual electrons moving from one battery terminal to another.

Third, is the double the distance problem. Remember in what context we're talking about when we say double the distance. When we are talking about two seperately charged sheets. After that, try to answer the following.

Why does votage double when distance doubles?

Why does it take double the energy when we double the distance?

Why does it take double the work (Fd) when we double the distance even though the attraction (F) is the same?

If you notice, all the three questions are analogous.

Siddhartha Srivastava - 6 years ago

@Siddhartha Srivastava – Yes, it takes twice the work. But so what? It's like saying that if you were to hold two magnets from a tall building over the edge, the magnet you're not holding (but trying to attract) will fall because potential energy in that direction (due to gravity) is far greater than potential energy above it (due to the electric force). But this simply isn't true now, is it?

This is how I see it. It simply makes no difference to me if potential is greater - unless the intensity of the electric field is greater, I don't see the reason for charges to stop flowing.

John M. - 6 years ago

@Siddhartha Srivastava – So we never really definitively came to an agreement now, did we?

Don't know if you'll read this but - hope you had a great summer. I'm in an engineering school right now (NJIT) on full tuition - majoring in Electrical Engineering (so far). I have applied to a CLEP exam for Wednesday - and I have 6 weeks of work to get done by that time... Sounds familiar. But yeah I'm CLEP-ing because I got a 2 on the AP exam for E&M - for obvious reasons. But now - it's on. And yet this fundamental question goes unanswered. I think I need to understand current itself a little better - would you agree? Then I may interpret your responses a little better. But if you have anything to respond to my May 29 reply, please go right ahead. I find your explanations very useful.

Other than that, I'll probably be posting notes around soon asking for conceptual help - and I hope I'll see you around there, because you're the total best in this field xD If not of course I won't mind - people have responsibilities. Just letting you know - I remember your awesomeness and would love to see more of it ;)

Enjoy your weekend!

John M. - 5 years, 9 months ago

@John M. – Hope you had a great summer too.

So how's college life? It must be great to talk to professors and get your problems solved there instead of posting them on forums and waiting for someone to help a few hours later.

Also, how did you give the AP in your senior year? That means you gave the AP after you applied to colleges, right? Which means you couldn't have given you AP score when applying? I'm just asking because I have to apply this year, and the whole process is so confusing as an international student.

And I'll try to respond to the May 29 comment. I remember replying, but I'm not sure what happened. Anyways, I have my midterms this month so I might not be able to reply.

Anyways, best of luck with your CLEP exams.

Siddhartha Srivastava - 5 years, 9 months ago

@Siddhartha Srivastava – College life, eh? Well my trip to the Harvard Model Congress program certainly paid off. Most of the students are so introverted that it is negatively affecting their chances at leadership and networking. Unfortunately the "book-smart street-dumb" stereotype is mostly true around my area. But this, of course, gives me a definitive edge over everyone else, for I'm "all-smart" ;) Will be running for President of Freshman Students in debates and elections so... hope that goes well.

As for AP, you can apply to college first and go through all the processes, and later on send the AP scores. That's what I did. However, there's something called an "orientation" for most of the colleges, where you go to basically get known to the campus and the resources available to you, as well as meet new people and scout the surrounding area. A tip - if it says "free breakfast," don't skip breakfast - they're usually cheap and will only provide some bagels, donuts, muffins, chopped melons, and some juice. Weell if you're into that stuff.... But yeah so in my case, there were two orientations - and I had to miss the first one because my AP scores weren't in yet. On the later days of the orientation, you REGISTER for your courses - and your AP scores will determine whether or not you will be allowed to skip certain classes and go straight to the advanced ones. One downside to missing the first orientation is that your desired classes and their times throughout the week may be taken up - so you may have to work with an undesired schedule, such as taking some Friday classes, or evening classes (unless you're cool with that).

But generally colleges in US will be flexible with such issues. What you should do, pretty much always, is be very political. Meaning, don't just mindlessly follow the system. Try to work your way around it. In my case, I have demanded the CLEPs and was not going to be happy with my 2 in AP E&M. I could have just let it go and taken Physics II - but instead I looked for any other possibilities. And I'm doing good so far ;) So what you should do is give them a call and ask them this question of yours. Ask them if you can register during the first orientation without your AP scores, and if you choose any classes that require proof of AP credit you can show it to them later. Generally this will be OK, because students are allowed to add/drop classes even a few weeks after the SECOND orientation. So give it a go, and see what happens. Remember, it's just like chess - you don't HAVE TO defend the piece. You can knock down the attacking piece instead - or leave the chessboard entirely if the game shows no promise. If working inside a system isn't cutting it, work around it.

If I missed something let me know.

Cheers

John M. - 5 years, 9 months ago

@John M. – Don't you have the AP on Monday. I'll reply after that. Better for the both of us.

Siddhartha Srivastava - 6 years, 1 month ago

@Siddhartha Srivastava – You're right.

John M. - 6 years, 1 month ago

@Siddhartha Srivastava – Alright I'm through with all that jumbo.

I'm also more familiar with currents now, as I went ahead.

But the questions persist. It'd be super-awesome if you could answer them - but if not, I'll just wait till I get to college, I guess. I understand this is probably really bothersome ;p

John M. - 6 years ago

@Siddhartha Srivastava – Hello.

John M. - 5 years, 7 months ago

@John M. – Hey. Sorry for not replying. Stuff came up. Haven't been able to use Brilliant much either.

I can't make heads or tails of our previous conversation. Brilliant's format makes it hard to figure out which comment is where chronologically.

Do you still have a problem with this stuff? If you do, could you restate in a reply to this comment? Thanks. And how did your CLEP exam go?

Siddhartha Srivastava - 5 years, 7 months ago

@John M. – Here's the original:

Here's the modified one:

Alright so here's my idea: In the second image, we've isolated a certain region of the wire and assumed it has a weaker electric field than the rest of the wire. It also follows it has a smaller current. (I mean, I don't know about the last one - didn't study currents in depth yet). So, sticking with electric fields, the battery will 'induce' an electric field across that region to 'equilibrate' it with the rest of the wire - so that the electric field is the same everywhere. Now, from what I know, the voltage of the battery determines the voltage across the circuit. But... WHY? Woah I have no idea. I think that's the problem. I mean, I can only think about the voltage between the battery's terminals - but I don't know how that applies to the entire circuit!

Anyway, the idea is - it's the same as with the capacitor - except now we have the plates. And the plates will allow no current flow. However, they will still allow an electric field. And, what I do know is, the electric field will cease 'intensification' once its field strength matches that of the rest of the circuit. Maybe there's something to this.

But I feel like I should move on and will find answers later on - unless you already have 'em. Anyway, sorry for the super-long blocks of text - I get carried away sometimes ;p

Cheers!

John M. - 6 years, 1 month ago

You have a point. Apparently, both of us are going through the same syllabus and I feel cheated at the physics class for poorly explained physical phenomena

Agnishom Chattopadhyay - 6 years, 1 month ago

It seems they expect you to simply take facts for granted and just know how to solve physics problems. For me, if I don't see the concept in my head exactly the way it's supposed to be, I'm not moving on. It has to be as simple and clear as $\overrightarrow {a } = \frac{\overrightarrow { F_{net}}}{m}$ ;)

John M. - 6 years, 1 month ago

electrons stop flowing btw the capacitor and the battery because the capacitor and the battery are now like an equipotential surface.trust me you know that the workdone on an equipotential surface is zero.so there no flow of electrons

Benjamin ononogbu - 6 years ago

Math	Appears as
Remember to wrap math in `\(` ... `\)` or `\[` ... `\]` to ensure proper formatting.
`2 \times 3`	$2 \times 3$
`2^{34}`	$2^{34}$
`a_{i-1}`	$a_{i-1}$
`\frac{2}{3}`	$\frac{2}{3}$
`\sqrt{2}`	$\sqrt{2}$
`\sum_{i=1}^3`	$\sum_{i=1}^3$
`\sin \theta`	$\sin \theta$
`\boxed{123}`	$\boxed{123}$