Fallacy of the Isosceles Triangle

Throughout the last of couple of weeks, we've been having some fun with geometrical fallacies. We've seen what makes them work and how to spot the wrong arguments that constitute the fallacious proofs. Today we're going to end the series with a classic geometrical fallacy; one that is possibly the most popular when it comes to geometric fallacies: the "proof" that all triangles are isosceles.

This fallacy has been attributed to Charles Dodgson who is better known as Lewis Carroll. Most people know Carroll as the author of the children's book, Alice's Adventure In Wonderland. But what some people don't know is Carroll was also a noted mathematician and logician who loved some recreational mathematics.

This post is going to be pretty much like the earlier ones. I first present you with the "proof". Then I ask you think about what went wrong and why. Finally I walk you through the steps and reveal the invalid argument. So let's get on with it!

Imgur

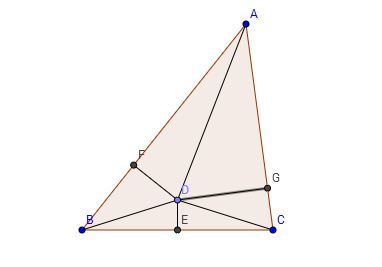
Let $ABC$ be a triangle. Draw the angle bisector of $\angle A$ and the perpendicular bisector of $BC$. Notice that if they are the same line, $\triangle ABC$ is isosceles. If they are not the same line, they're going to intersect at a point. Let's call that $D$.

Let $E$ be the midpoint of $BC$. $F$ and $G$ are the feet of perpendiculars from $D$ to $AB$ and $AC$ respectively. Join $B, D$ and $C, D$.

Notice that $\triangle BDE$ and $\triangle CDE$ are congruent [SAS], because $BE=CE$, $DE=DE$ and $\angle DEB=\angle DEC$ since they are equal to a right angle.

So, now we can write $BD=CD$ $\cdots (1)$.

Triangles $ADF$ and $ADG$ are also congruent [AAS] as $\angle DFA=\angle DGA=90^\circ$, $\angle FAD=\angle GAD$ [remember that $AD$ was the angle bisector of $\angle BAC$] and $AD=AD$.

From that we can write $DF=DG$ $\cdots (2)$

And $AF=AG$ $\cdots (3)$.

Now we move on to triangles $DBF$ and $DCG$.

From $(1)$ and $(2)$ and from the fact that $\angle DFB$ and $\angle DGC$ are right angles, we can conclude that triangles $DBF$ and $DCG$ are also congruent.

And that means $FB=GC$ $\cdots (4)$.

Now add $(3)$ and $(4)$ together and see what happens.

$AF+FB=AG+GC$

$\Rightarrow AB=AC$

That means $\triangle ABC$ is isosceles! How did that happen? You can use the similar arguments to prove $BC=AC$ and prove that all triangles are actually equilateral!

Now comes the slightly over-asked question: what went wrong? Go back to the steps and try to figure it out. Don't continue reading if you don't want to know the solution without giving it a shot.

I take it that you've thought about the fallacy for a while. Have you figured it out? If repeatedly talking about fallacies taught us one thing, it's this: drawing a sufficiently accurate picture always helps. And that advice isn't restricted only to fallacies like this. You can use this even while solving normal geometry problems. An accurate figure helps you make good educated guesses [for example, "the lines sure look like they're parallel, let's prove it! And that angle looks like a right angle. Can I prove it?...]. So, let's take a look at a more accurate picture:

Imgur

This actually makes sense. The point $D$ is actually outside the triangle. We never proved where $D$ was. We only assumed [incorrectly] that it was inside. This also tells us that everything up to the last step was absolutely correct. The lengths were equal. The triangles were congruent as well. Even $AF+FB=AG+GC$ was correct. But the transition to the final step was not. We made an incorrect assumption that $AG+GC=AC$. If $AB>AC$, $AC=AG-GC$, not $AG+GC$. This is what made us arrive at a wrong conclusion.

So, there you have it! This is the last post of the geometric fallacies series. I hope you had as much fun reading the posts as I had writing them. And thank you for staying with me till the end. If you missed one or two posts, you can check out my feed here or try the #mathematicalfallacies tag.

Until next time!