G-filtered Polycules

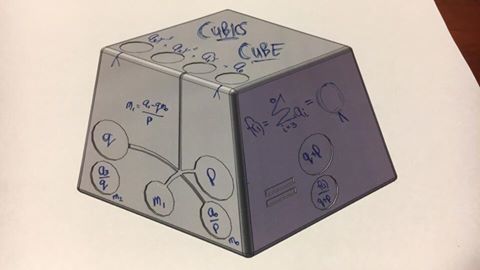
The strongest thing i can say about G-filtered Polycules, is that "someday, grade school kids will be rational-factoring cubic polynomials without even knowing it". So you can only imagine what they will be capable of when they enter High School, College, and Beyond, and NOT just in Mathematics !!! A design for the "game" they play after recess might look like the image below, "CUBICS CUBE" sounds catchy for a title. Find $m$ and the factors of the cubic $x^3-3x^2-11x+21$ magically appear, the BIG CIRCLE equation reveals the Rational factor, and the LITTLE CIRCLE equation reveals the quadratic factor. 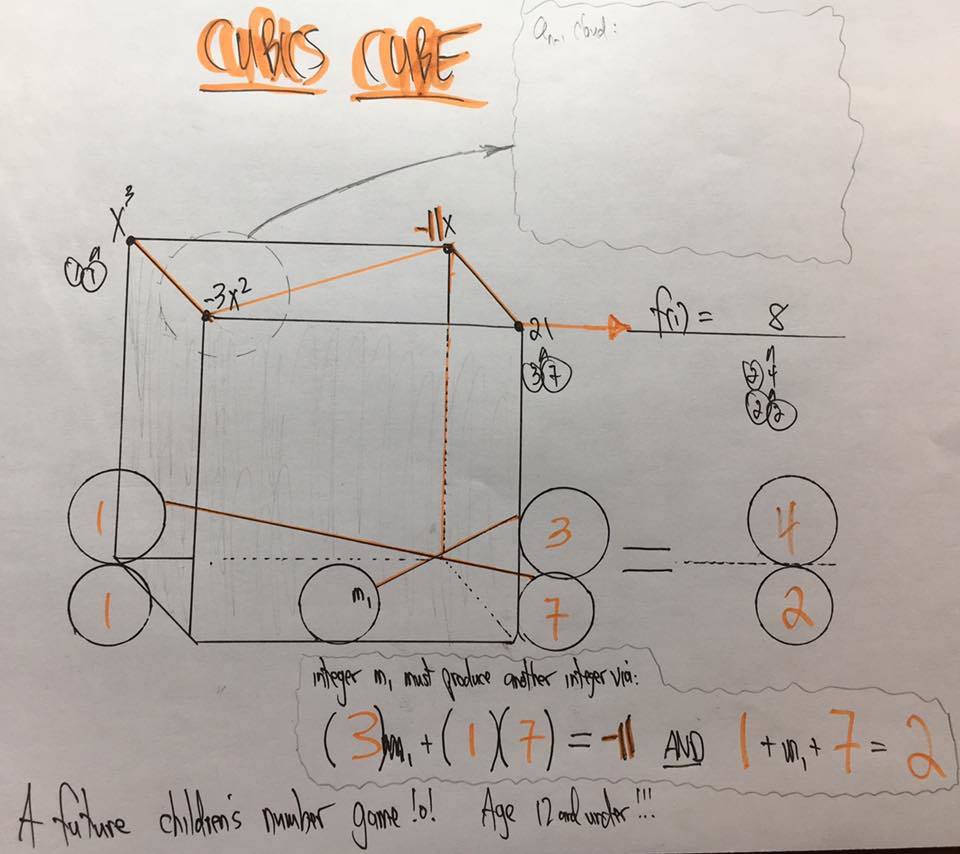The pic below shows the formal Mathematics in factoring $x^3-3x^2-11x+21$ via Unit Conjugate G-filtration. Note: DROS is Descartes' Rule Of Signs, which when used with Conjugate G-filtration makes a powerful factoring Tool for ANY polynomial !!! Junior High School grads can now factor an integer-coefficient polynomial of ANY degree. The pic below shows the work required in factoring the cubic $2x^3-7x^2-16x+35$ . How would you find all the rational factors ??? This facebook video explains the Game of G-filtered Polycules for Cubics; leave a comment:

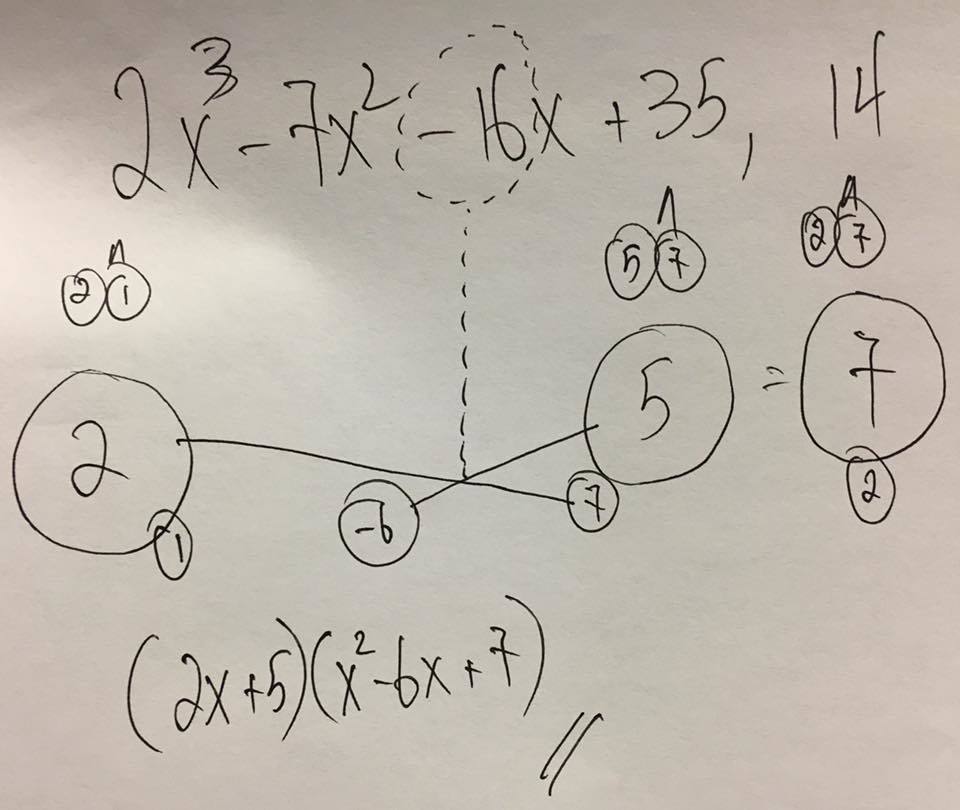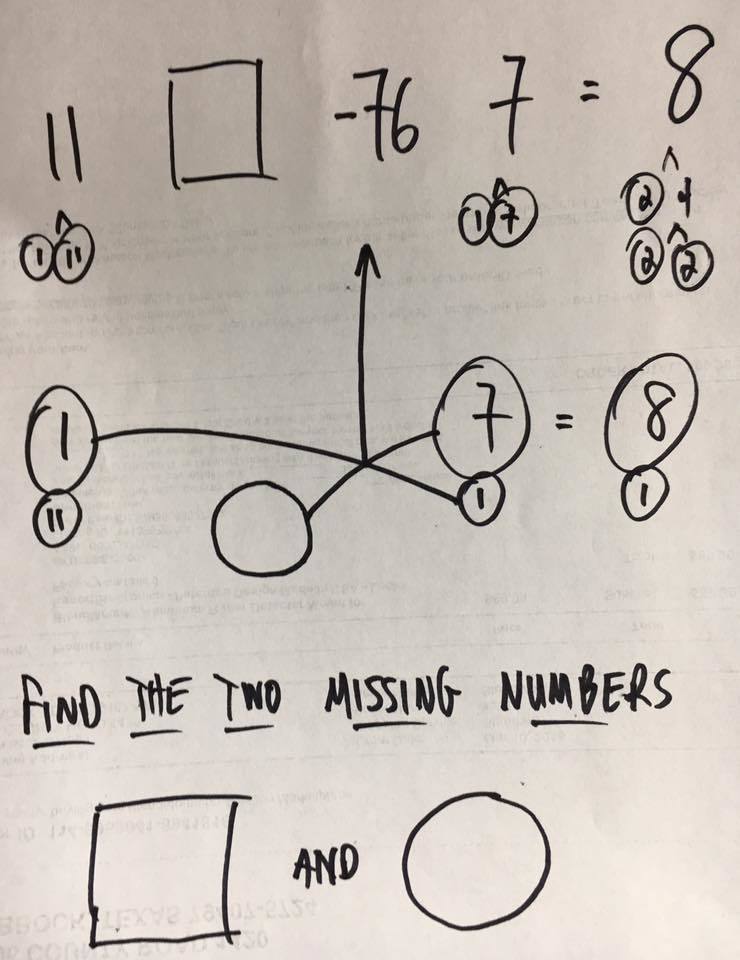
https://www.facebook.com/TruSpot/videos/vb.1021243805/10208395010805791/?type=2 And here is the Introduction to my Book(1), and below that, the Quadratic $x^2-7x+12$ factored via Unit G-filtration. And finally, this is the "G-filtration Cumulative Summary" from Book(1):

Markdown	Appears as
`italics` or `_italics_`	italics
`bold` or `__bold__`	bold
- bulleted - list	bulleted list
1. numbered 2. list	numbered list
Note: you must add a full line of space before and after lists for them to show up correctly
paragraph 1 paragraph 2	paragraph 1 paragraph 2
`[example link](https://brilliant.org)`	example link
`> This is a quote`	This is a quote
# I indented these lines # 4 spaces, and now they show # up as a code block. print "hello world"	# I indented these lines # 4 spaces, and now they show # up as a code block. print "hello world"

Math	Appears as
Remember to wrap math in `\(` ... `\)` or `\[` ... `\]` to ensure proper formatting.
`2 \times 3`	$2 \times 3$
`2^{34}`	$2^{34}$
`a_{i-1}`	$a_{i-1}$
`\frac{2}{3}`	$\frac{2}{3}$
`\sqrt{2}`	$\sqrt{2}$
`\sum_{i=1}^3`	$\sum_{i=1}^3$
`\sin \theta`	$\sin \theta$
`\boxed{123}`	$\boxed{123}$

G-filtered Polycules

Comments