Gauss: The Prince of Mathematics

As you progress further into college math and physics, no matter where you turn, you will repeatedly run into the name Gauss. Johann Carl Friedrich Gauss is one of the most influential mathematicians in history. Gauss was born on April 30th, 1777 in a small German city north of the Harz mountains named Braunschweig. The son of peasant parents (both were illiterate), he developed a staggering number of important ideas and had many more named after him. Many have referred to him as the princeps mathematicorum, or the “Prince of Mathematics.”

As part of his doctoral dissertation (at the age of 21), Gauss was one of the first to prove the fundamental theorem of algebra. He went on to publish seminal works in many fields of mathematics including number theory, algebra, statistics, analysis, differential geometry, geodesy, geophysics, electrostatics, astronomy, optics, etc. Number theory was Gauss’s favorite and he referred to number theory as the “Queen of Mathematics”.

One of the reasons why Gauss was able to contribute so much math over his lifetime was that he got a very early start. There are many tales of his childhood precociousness. The most famous anecdote of young Gauss, is the time he found the shortcut for calculating sums of an arithmetic progression at the tender age of 10.

One day at school, Gauss's teacher wanted to take a rest and asked the students to sum the integers from 1 to 100 as busy work. After a few seconds, the teacher saw Gauss sitting idle. When asked why he was not frantically doing addition, Gauss quickly replied that the sum was 5050. His classmates and teacher were astonished, and Gauss ended up being the only pupil to calculate the correct answer.

$1+2+3+ \cdots +(n-1) = ?$

The story may be apocryphal, and is told different ways in different sources. Nobody is sure which method of summing an arithmetic sequence Gauss figured out as a child. Though there are several ways young Gauss might have solved it, one of them has a concise, intuitive, and elegant visual representation.

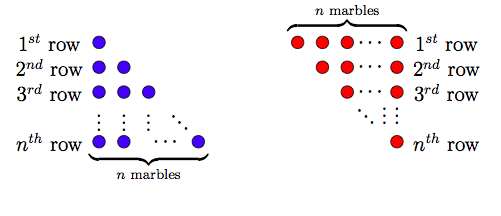Arithmetic Progression

Consider two sets of marbles as shown in the Figure 1. The left pile has $n$ rows of blue marbles, where the $j$th row contains $j$ marbles. The right pile has $n$ rows of red marbles, where the $j$th row contains $n+1-j$ marbles.

The total number of blue marbles is given by

$1+2 +3+ \cdots + (n-1)+n,$

while the total number of red marbles is given by

$n+(n-1)+(n-2) + \cdots + 2 + 1,$

and clearly both contain the same number of marbles. Now if we were to add these piles together as shown in Figure 2, we would then get a stack with $n$ rows, where each row contains $n + 1$ marbles.

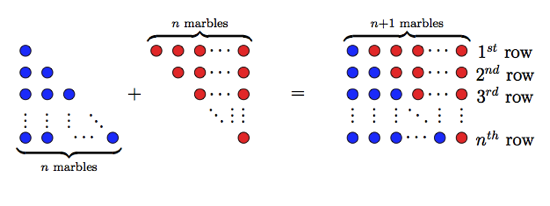Arithmetic Progression

The total number of marbles in the added pile would be $n(n + 1)$. Since both the red pile and the blue pile have an equal number of marbles, each pile must have contributed $\frac{n(n + 1)}{2}$ marbles. Hence, we obtain:

$1+2+3+...+(n-1)+n= \frac{n(n-1)}{2}.$

To sum all the numbers from 1 to 100, Gauss simply calculated $\frac{100\times (100+1)}{2}=5050$, which is immensely easier than adding all the numbers from 1 to 100. All sane humans would rather do one addition, one multiplication, and one division than do lots of tedious addition operations. Note that $1+2+3 + \ldots +(n-1)+n$ must always be a natural number. Even though the above formula divides by 2, the result will always be a natural number. This is because the numerator will always be conveniently even due to the multiplication properties of parity. For example, $n$ could either be even or odd. If $n$ is even, then $n+1$ is odd and hence

$n \times (n + 1) = even \times odd = even.$

Similarly, if $n$ is odd, then $n + 1$ is even and hence

$n \times (n + 1) = odd \times even = even.$

Therefore, the numerator is always even and $n(n + 1)$ is always a natural number.

Numbers of the form $\frac{n(n + 1)}{2}$ are called triangular numbers, for reasons well illustrated in the above figures. The first few triangular numbers are

$1,3,6,10,15,21,28,36, \ldots.$

It is commonplace to encounter an application of summing an arithmetic sequence, both in classroom problems, and in describing the broader world. It is less common to meet 10 year olds who figure out the tricks of arithmetic progression for themselves. It is even less common for a precocious 10 year old, to grow up to be nearly as prolific as Gauss. Check out this list of things named after Gauss.