Geometry with approximations

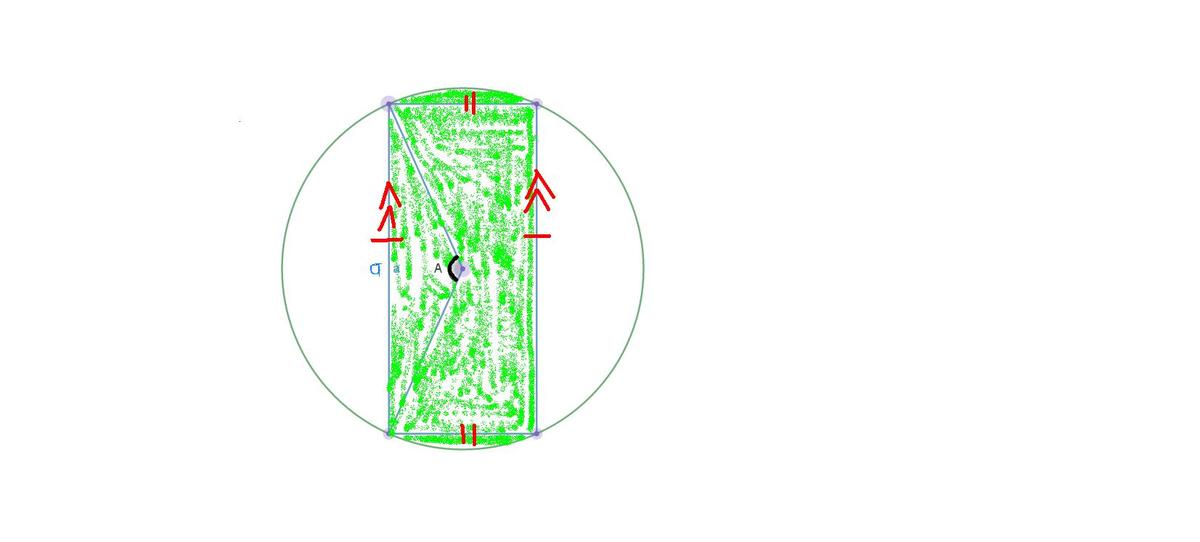
Given: Area of the shaded region (green) =14×Area of the whole circle (Diagram not to scale)\text{Area of the shaded region (green) } = \dfrac{1}{4} \times \text{Area of the whole circle} \text{ (Diagram not to scale)}

The vertical lines(each length a) are drawn parallel and equidistant from the axis of symmetry of the figure.\text{The vertical lines(each length a) are drawn parallel and equidistant from the axis of symmetry of the figure.}

The angle A is between two radii of the circle joining with the left line.\text{The angle A is between two radii of the circle joining with the left line.}

Let R = radius of the circle\text{Let R = radius of the circle}

12×(R)2×(A)−12×(R)2×sin⁡(A)=12⋅34×π(R)2\dfrac{1}{2} \times (R)^2 \times (A) - \dfrac{1}{2} \times (R)^2 \times \sin(A) = \dfrac{1}{2} \cdot \dfrac{3}{4} \times \pi (R)^2 [Region of one unshaded part]\text{[Region of one unshaded part]}

(A)−sin⁡(A)=3π4 (A) - \sin(A) = \dfrac{3\pi}{4}

sin⁡(A)=A−3π4 \sin(A) = A - \dfrac{3\pi}{4}

a=2×sin⁡(A2)×Ra = 2 \times \sin{ \left( \dfrac{A}{2} \right) } \times R

Perimeter of the unshaded region =2×(a+(R)(A)) = 2 \times ( a + (R) (A) )

Using (3rd order) Taylor Series approximation:\text{Using (3rd order) Taylor Series approximation:}

A−A33!≈A−3π4A - \dfrac{A^3}{3!} \approx A -\dfrac{3\pi}{4}

A3≈9π2 A^3 \approx \dfrac{9\pi}{2}

A≈2.417988 radA \approx 2.417988 \text{ rad}

Perimeter of unshaded region≈2×[(2×sin⁡(2.4179882)×R)+(2.417988)(R)]\text{Perimeter of unshaded region} \approx 2 \times \left[ \left( 2 \times \sin{ \left( \dfrac{2.417988}{2} \right) } \times R \right) + ( 2.417988)(R) \right]

Perimeter of unshaded region≈8.57702R\text{Perimeter of unshaded region} \approx 8.57702 R

Note : The answer is inaccurate because the approximation only works well near  \text{Note : The answer is inaccurate because the approximation only works well near }

x=0x = 0.  Nevertheless, Taylor Series is useful to approximate difficult calculations.\text{ Nevertheless, Taylor Series is useful to approximate difficult calculations.}

Note by Lin Shun Hao
1 year, 1 month ago

No vote yet
1 vote

  Easy Math Editor

This discussion board is a place to discuss our Daily Challenges and the math and science related to those challenges. Explanations are more than just a solution — they should explain the steps and thinking strategies that you used to obtain the solution. Comments should further the discussion of math and science.

When posting on Brilliant:

  • Use the emojis to react to an explanation, whether you're congratulating a job well done , or just really confused .
  • Ask specific questions about the challenge or the steps in somebody's explanation. Well-posed questions can add a lot to the discussion, but posting "I don't understand!" doesn't help anyone.
  • Try to contribute something new to the discussion, whether it is an extension, generalization or other idea related to the challenge.
  • Stay on topic — we're all here to learn more about math and science, not to hear about your favorite get-rich-quick scheme or current world events.

MarkdownAppears as
*italics* or _italics_ italics
**bold** or __bold__ bold

- bulleted
- list
  • bulleted
  • list

1. numbered
2. list
  1. numbered
  2. list
Note: you must add a full line of space before and after lists for them to show up correctly
paragraph 1

paragraph 2

paragraph 1

paragraph 2

[example link](https://brilliant.org)example link
> This is a quote
This is a quote
    # I indented these lines
    # 4 spaces, and now they show
    # up as a code block.

    print "hello world"
# I indented these lines
# 4 spaces, and now they show
# up as a code block.

print "hello world"
MathAppears as
Remember to wrap math in \( ... \) or \[ ... \] to ensure proper formatting.
2 \times 3 2×3 2 \times 3
2^{34} 234 2^{34}
a_{i-1} ai−1 a_{i-1}
\frac{2}{3} 23 \frac{2}{3}
\sqrt{2} 2 \sqrt{2}
\sum_{i=1}^3 ∑i=13 \sum_{i=1}^3
\sin \theta sin⁡θ \sin \theta
\boxed{123} 123 \boxed{123}

Comments

There are no comments in this discussion.

×

Problem Loading...

Note Loading...

Set Loading...