How Medicines Are Made

By Megan Blewett

The Challenges of Drug Development

Every year dozens of new medicines are approved by the Food and Drug Administration (FDA) to treat a range of diseases, but the stories of how these drugs transition from a research lab to a patient’s bedside are often not well known. The chemists and biologists who develop a promising compound into an effective drug have many factors to consider. For instance, how does the drug get to the desired location in the body? How do doctors know how much to give a patient? How do pharmaceutical scientists know how long the drug remains in the body before being broken down or excreted? These types of questions all have potentially fatal consequences for patients, and all fall within the important field of pharmacokinetics. Pharmacokinetics is the study of the fate of drugs in the human body. To illustrate the principles of pharmacokinetics, let’s examine the fate of the antimalarial drug chloroquine in the body.

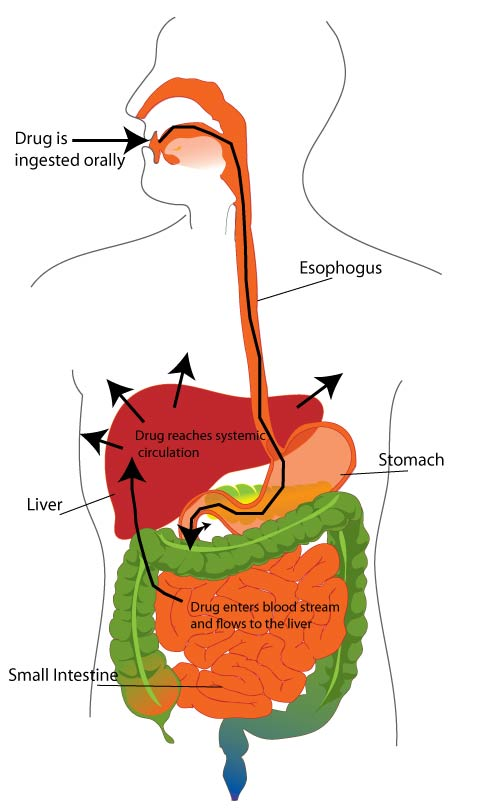Medicine Path

The path medicine takes from your mouth to your circulatory system.

How does a drug make it to the target organ?

When a drug is administered orally, it travels down the esophagus, through the stomach, and into the intestines. Absorption refers to the amount of drug that passes from the gut and into the blood that flows to the liver. As you might expect, many factors affect how well a drug is absorbed. For example, the drug needs to be stable in the highly acidic environment of the stomach, it needs to bypass the countless bacteria lining the intestines (known as our microflora), and it can’t get broken down in the complex mixture of foods we eat.

The next stop for the drug is the liver, where the drug will face a host of enzymes which metabolize and break down our food. Many of these enzymes are known as cytochrome P450’s, which oxidize chemical compounds to facilitate their excretion. Pharmaceutical drugs have to be specially designed so that they are not oxidized to an inactive form by cytochrome P450’s.

Once a drug makes it past the liver and reaches the systemic circulation (the blood flowing throughout the body), it can stay in the blood or it can travel into different bodily tissues. Where the drug goes is determined in large part by 1) how hydrophilic (i.e. water-loving) it is and 2) how big it is.Let’s look at the structure of chloroquine in order to figure out if it will be more hydrophobic or hydrophilic. Polar molecules with many heteroatoms (usually nitrogen, oxygen, or sulfur) are generally more hydrophilic because they can form hydrogen bonds with water molecules. Carbon-carbon and carbon-hydrogen bonds are generally hydrophobic because these bonds are not polar and interact only weakly with water molecules. Looking at the structure of chloroquine, one can see that it has many more carbon-carbon and carbon-hydrogen bonds than it does carbon-heteroatom bonds. Therefore this is a very hydrophobic molecule.

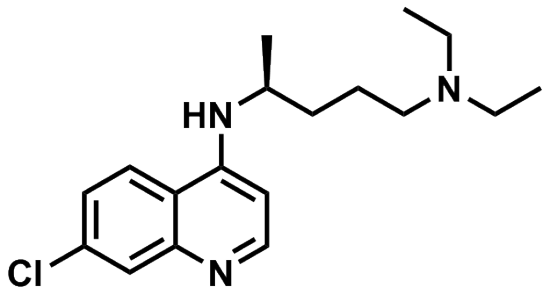Chloroquine Skeletal

Skeletal diagram of chloroquine.

Hydrophilic molecules will tend to stay in the blood because this is an aqueous environment, however, chloroquine and other hydrophobic molecules will travel into surrounding tissues. Likewise very large molecules (such as proteins) are usually unable to enter cells and are therefore limited to the blood.

How do scientists quantify the partitioning between the blood and the rest of the body? They use a term called “volume of distribution”. The volume of distribution is the total amount of drug in the body (usually in mg) divided by the plasma drug concentration (usually in mg/L). You can see that when the units are cancelled, this gives a volume in liters. The lower the plasma drug concentration, the higher the volume of distribution. The drug is moving into other tissues and is literally being distributed over a larger volume.

Let’s look at some sample volumes of distribution. Immunoglobulin G is a protein known as an antibody. It is very large (150 kDa) and cannot easily get into cells. It is therefore localized predominantly to the blood and has a low volume of distribution: 5 L. This is essentially the volume of blood in an adult human at any given time. Imipramine, on the other hand, is a small, hydrophobic molecule that can easily cross lipid membranes (remember that lipids are extremely hydrophobic). It therefore easily traverses other tissues and has a large volume of distribution: 2100 L. Chloroquine is even more hydrophobic and has one of the highest volumes of distribution of any approved drug: 12950 L! This means that, if you take chloroquine, most of the drug is found not in the blood but in tissues like fat.

How do doctors find the right dose?

Figuring out how much of a drug to give a patient can literally be a life or death decision: too little and the drug concentration may be too low to produce the desired effect, too much and adverse side effects or toxicity could seriously harm the patient. It turns out that the volume of distribution is critical to this calculation. The amount of drug given to a patient is the volume of distribution (in L) multiplied by the target plasma concentration (in mg/L). You can think of the drug as being “dissolved” in the volume of distribution (body tissue).

But how do doctors know what the target plasma concentration is? This is related to the steady state plasma concentration. Imagine that a drug is being administered at a continuous rate to a patient. If the drug is taken orally, it takes some time to reach the blood, during which time the plasma concentration rises. After this period though, the plasma concentration plateaus as the body begins to clear the drug at the same rate it is administered.

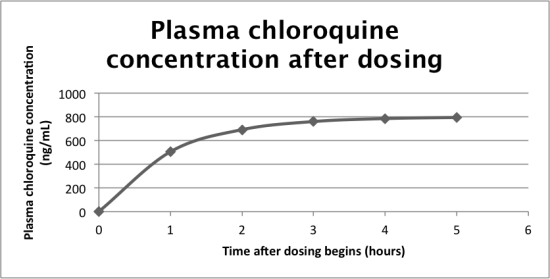Data Edwards 1

Data from Edwards et al, 1988.

The drug can be cleared in one of two ways: metabolism in the liver or excretion from the kidneys. The faster a drug is cleared by metabolism or excretion, the lower the steady state plasma concentration will be. Scientists usually take this steady state concentration as the target plasma concentration.

Clearance is measured in L/hour and is taken to be the liters of blood cleared of drug per hour. Chloroquine has a very high clearance rate of 45 L/hour and so its steady state plasma concentration is quite low.

How long does a drug stay in the body?

Once researchers know the clearance rate of a drug and the volume of distribution, they can calculate how long the drug will remain in the body. One of the most widely used measures of a drug’s duration in the body is the half-life; this is the amount of time required for a drug’s plasma concentration to decrease by half. Let’s look at how the half-life can be calculated from the parameters we’ve already discussed. The plasma drug concentration decreases exponentially after dosing according to:

$C_t=C_0 e^{-kt}.$

Where Ct is the plasma concentration of the drug at time t, C0 is the initial concentration (usually taken to be steady state), k is the elimination rate constant, and t is the amount of time passed. The elimination rate constant, as you might guess, has units of inverse time and is the proportion of the drug in the body eliminated per unit time. It can be expressed as drug clearance (liters/hour) divided by the volume of distribution (in liters). The half-life is the time at which Ct/C0 = 0.5:

$0.5 = e^{\frac{-L}{V} {t_{1/2}}}.$

where $L$ is the clearance and $V$ is the volume of distribution.

Taking the natural log of both sides gives:

$t_\frac{1}{2}=ln{2} {\frac{V}{L}}.$

Therefore, we can see that the half-life is directly proportional to the volume of distribution and inversely proportional to the clearance. The more widely distributed the drug is throughout the body, the harder it will be to remove the drug; blood has much higher turn-over than, for example, fat. Likewise, the higher the clearance rate, the faster a drug is metabolized or excreted, and the shorter the half-life.

Given what we know about chloroquine, we can now determine its half-life. Chloroquine has an extremely high volume of distribution: 12,950 L. It also has a high clearance rate of 45 L/hour. This means its half-life is roughly 200 hours! If you have been taking chloroquine and your blood has reached a steady state concentration, it will take over 8 days for the plasma concentration to decrease by 50%. In the world of medicine, this is a very long time.

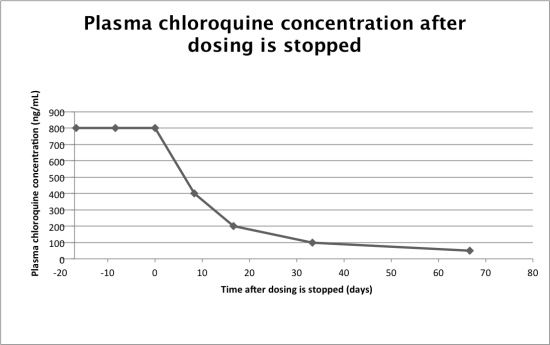Data Edwards 2

Data from Edwards et al, 1988.

Why Am I interested in Pharmacokinetics?

Pharmacokinetics interests me because it is incredibly important for treating disease and because it unites the fields of chemistry and biology in a quantitative way

For more reading on pharmacokinetics:

Birkett, DJ. Pharmacokinetics made easy. New York: McGraw Hill, 2010

For more background on Malaria and chloroquine check out:

Krafts, K.; Hempelmann, E.; Skorska-Stania, A. Parasitol Res 2012, 111, 1-6

Chloroquine data are from:

NCBI