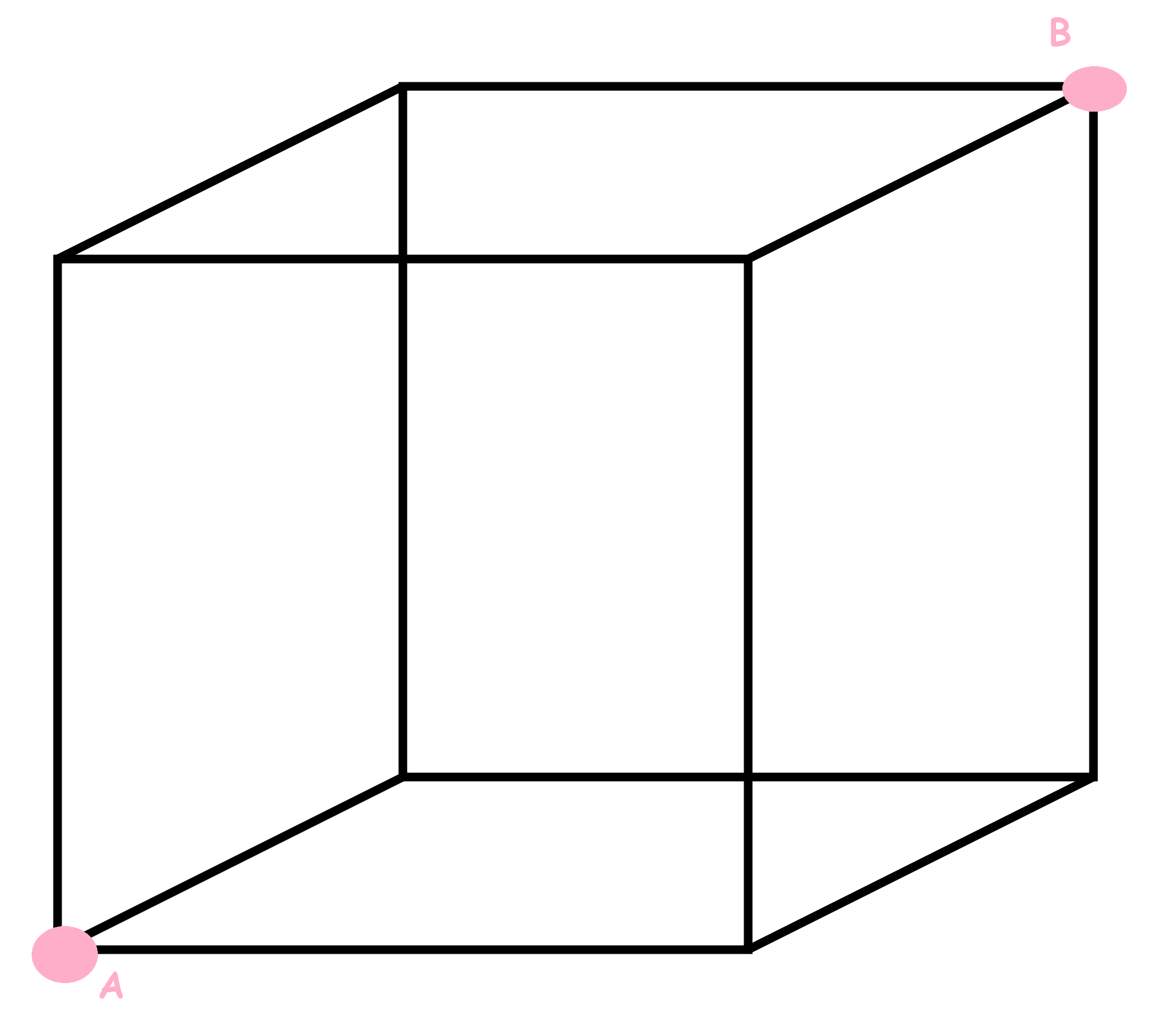
Infinite cubic grid of resisrors

Can we find net resistance between the body diagonal points of a infinite cubic lattice ?

The objective is to find net resistance between A & B of the given cube which is part of infinite grid. Let the resistance between any two adjacent vertices is R

#ElectricityAndMagnetism #Resistance #Grids #infinite

Easy Math Editor

This discussion board is a place to discuss our Daily Challenges and the math and science related to those challenges. Explanations are more than just a solution — they should explain the steps and thinking strategies that you used to obtain the solution. Comments should further the discussion of math and science.

When posting on Brilliant:

Use the emojis to react to an explanation, whether you're congratulating a job well done , or just really confused .
Ask specific questions about the challenge or the steps in somebody's explanation. Well-posed questions can add a lot to the discussion, but posting "I don't understand!" doesn't help anyone.
Try to contribute something new to the discussion, whether it is an extension, generalization or other idea related to the challenge.
Stay on topic — we're all here to learn more about math and science, not to hear about your favorite get-rich-quick scheme or current world events.

Markdown	Appears as
`italics` or `_italics_`	italics
`bold` or `__bold__`	bold
- bulleted - list	bulleted list
1. numbered 2. list	numbered list
Note: you must add a full line of space before and after lists for them to show up correctly
paragraph 1 paragraph 2	paragraph 1 paragraph 2
`[example link](https://brilliant.org)`	example link
`> This is a quote`	This is a quote
# I indented these lines # 4 spaces, and now they show # up as a code block. print "hello world"	# I indented these lines # 4 spaces, and now they show # up as a code block. print "hello world"

Math	Appears as
Remember to wrap math in `$` ... `$` or `\[` ... `\]` to ensure proper formatting.
`2 \times 3`	$2 \times 3$
`2^{34}`	$2^{34}$
`a_{i-1}`	$a_{i-1}$
`\frac{2}{3}`	$\frac{2}{3}$
`\sqrt{2}`	$\sqrt{2}$
`\sum_{i=1}^3`	$\sum_{i=1}^3$
`\sin \theta`	$\sin \theta$
`\boxed{123}`	$\boxed{123}$

Comments

Oh my.

Josh Silverman Staff - 5 years, 9 months ago

Yes, I know about 2 d version for resistors and fourier series for getting across square diagonal 2r/pi.[you know that Ishan :D ] But this is quite different fom those, much more difficult in getting the approach. You should share this so that someone reaches at the final ansWeR.

Pranjal Prashant - 5 years, 9 months ago

Here is the 2-d version of the problem.

Calvin Lin Staff - 5 years, 9 months ago

A simpler version would be two find the equivalent resistance between two diagonally opposite points in an infinite grid of resistors, which is still quite difficult. See the page I have linked Infinite grid of resistors

Ishan Tarunesh - 5 years, 9 months ago

It must be R/3

Jatin Chauhan - 5 years, 2 months ago

Solution ?

Rajdeep Dhingra - 5 years, 2 months ago

I asked for diagonally opposite points. Not adjacent ones

Pranjal Prashant - 5 years, 1 month ago

Total resistance (across points A and B) = ( 5/6 ) * R
I can't post a picture of my solution here

Vincent Miller Moral - 5 years, 9 months ago

Incorrect, This answer holds if there was a single cube of resistances rather than an infinite one.

Ishan Tarunesh - 5 years, 9 months ago

Yes it is haha, I considered only a 'cube'.

Vincent Miller Moral - 5 years, 9 months ago

but infinite series of resistors just push the "single cube value" to an exact number so my idea is that effective resistance is near (5/6)R (like 0.85R or 0.9R max but not less than (5/6)R). Please notify me if there is a mistake in my assumption.

Yeshas Bharadwaj - 5 years, 9 months ago

@Yeshas Bharadwaj – I could not understand "push the single cube value to an exact number". Also think of 2-D grid of infinite resistors in which we have to find the equivalent resistance between adjacent points which everyone knows to be R/2. What will be your argument in this one? Just because other resistances are present does not mean that the net would be greater than 5R/6 because resistances in parallel decrease the value

Ishan Tarunesh - 5 years, 9 months ago

@Yeshas Bharadwaj – Now what significance pushing values is of, the question is not an mcq, I want to know how it can be done, and I am sure that it is solvable. {although answer would not be beautiful perhaps}.

Pranjal Prashant - 5 years, 9 months ago

@Pranjal Prashant – got it but I dont know how to solve the way you told and yes the answer sure will be weird

Yeshas Bharadwaj - 5 years, 9 months ago

@Yeshas Bharadwaj – NO, IT IS NOT SO. In the fourier series , steps of integration are nasty, but answer is simply 2r/ $\pi$

Pranjal Prashant - 5 years, 9 months ago

@Pranjal Prashant – thanks for the info I will surely try to get the steps for your answer.

Yeshas Bharadwaj - 5 years, 9 months ago

see 2nd answer to the question in the link https://physics.stackexchange.com/questions/2072/on-this-infinite-grid-of-resistors-whats-the-equivalent-resistance It's for n dimensional grid

Anasua Dogra - 1 year ago

Math	Appears as
Remember to wrap math in `\(` ... `\)` or `\[` ... `\]` to ensure proper formatting.
`2 \times 3`	$2 \times 3$
`2^{34}`	$2^{34}$
`a_{i-1}`	$a_{i-1}$
`\frac{2}{3}`	$\frac{2}{3}$
`\sqrt{2}`	$\sqrt{2}$
`\sum_{i=1}^3`	$\sum_{i=1}^3$
`\sin \theta`	$\sin \theta$
`\boxed{123}`	$\boxed{123}$