JEE Advanced 2015- Incorrect problem, open discussion.

If you can in any way contribute, please do.
The above problem appeared in the JEE- Advanced 2015 examination in the second paper. The answer provided in the official answer key is . However, the problem is widely believed to be incorrect. The authorities conducting the examination accept suggestions related to the correctness of the published key till 11- Jun 2015, 12 noon (Indian standard time). I want to send them a fax related to the error in this problem and to do so, it would really help if the community could offer valuable suggestions about the problem.
Here is an (incorrect) solution which yields the supposed answer:
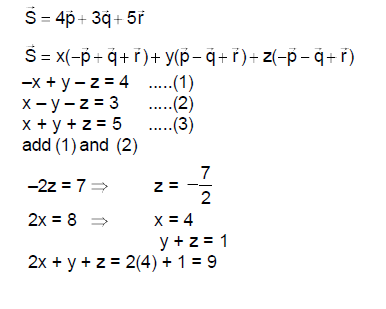
No vote yet
1 vote
Easy Math Editor
This discussion board is a place to discuss our Daily Challenges and the math and science related to those challenges. Explanations are more than just a solution — they should explain the steps and thinking strategies that you used to obtain the solution. Comments should further the discussion of math and science.
When posting on Brilliant:
*italics*or_italics_**bold**or__bold__paragraph 1
paragraph 2
[example link](https://brilliant.org)> This is a quote# I indented these lines # 4 spaces, and now they show # up as a code block. print "hello world"\(...\)or\[...\]to ensure proper formatting.2 \times 32^{34}a_{i-1}\frac{2}{3}\sqrt{2}\sum_{i=1}^3\sin \theta\boxed{123}Comments
If you think a solution is wrong, always work on finding out what the potential issue is, and why you disagree with it. In the case, since the solution appears correct / has no mistakes, then you should ask "How can this problem yield multiple answers"?
If you cannot convince yourself that there should be multiple/different answers, nor justify why this seemingly correct solution is wrong, then it is most likely correct. IE The burden of proof is on you.
The misconception that you have is in thinking that "the components of this vector are obtained from the projection down to the individual axis". This statement is only true if the axis are orthogonal to each other, like in the ijk case that we're used to.
As an easy (counter)example, think about the point (1,1,0) and the axis of (1,0,0),(0,1,0),(1,1,1). Clearly, the projections onto these axis are (1,0,0),(0,1,0) and something non-zero.
However, the components of (1,1,0) is not " (1,0,0)+(0,1,0)+ something non-zero".
Thanks for all your help. The final answer key was released today after considering feedback from public. It has been declared that all candidates be given marks for this particular problem regardless of the answer they have marked.
@Ronak Agarwal @Mvs Saketh @Sandeep Bhardwaj @Calvin Lin @Azhaghu Roopesh M
Th answer provided is absolute incorrect.
Log in to reply
Thanks for your comment sir. I just wanted to make sure that it was indeed incorrect before sending them a suggestion.
Log in to reply
A little thing you can add in your suggestion in against of the answer is :
If we take p=i^,q=j^,r=k^, then s=4i^+3j^+5k^ and now the question can be solved easily. and the answer comes out to be 43 (hope I made no calculation errors) :P
Log in to reply
Yes, you are right. I marked 4 as the answer due to this reason. Although now I feel like 7 might have been a better choice.