Mathematical and Statistical Probability of a Good Marriage in Pride and Prejudice.

$~$

In this note, I will prove that math can be used to derive a formula for love and detail the probabilities of perfect marriages. This is an assignment for English class in which we were instructed to do a project relating to $\textit{Pride and Prejudice}$, by Jane Austen. Naturally, I incorporated math into this... Hope you enjoy!

$\text{Mathematical and Statistical Probability of Perfect Marriages in Pride and Prejudice}$

$\text{By Trevor Arashiro}$

$~$

$\textbf{Abstract:}$ Beginning with a hypothetical situation, I will explain the probability that both A) a couple in pride and prejudice will be happiest in marriage B) the possibility of the optimal situation is maximized. This problem is based heavily off of the much more famous problem known as “The Secretary Problem” Of course, since this is a real life problem, mathematics is limited in its power to accurately analyze as is the greatest flaw of $P_{observed}$ vs $P_{calculated}$

Assumptions

• We assume a prior, random distribution with all elements being distinct

• The order of the set is both finite and known to all applicants and suitors (this will be explained below)

• We will be maximizing $P_{calculated}$ and not $P_{observed}$

• Each suitor will chose a wife regardless of her wishes as is customary of the time for parents to marry their daughters off. However, each woman may present herself differently to each man to influence his decision.

• Most importantly, the each man's perfect wife defined as $S_i$ where $0<i~\epsilon ~\Bbb{N} \leq n$ is 1 distinct element and the set of intersection of all $S_i$ is $I(S_i)=S_j\neq S_k$ for $j\neq k$. The same doesn’t hold for each woman as there are more women than men, thus naturally there is an overlap for women. This holds true throughout the story except for one case which should minimally affect our calculations.

Terms

$W(w_i)$ is the set of all women with elements $w_i$. $M(m_i)$ is the set of all men with elements $m_i$

$S_i$ is each man's woman of choice and $T_i$ is each woman's man of choice.

$I(S_i)$ is the set of every man's perfect wife which is the equivalent to the set $S(s_i)$. For convenience, I will refer to the latter from now on. The same can be said to the set of every woman's perfect husband.

Characters: 9 Women 6 Men (only 4 get married). Remember that each person is distinct.

Introduction: Hypothetical Situation

$\begin{array}{l|c|r}\text{n} & \text{r}& \text{P(r)} \\ \hline 1 & 1 &1.00\\ \hline 2&1&0.500 \\ \hline 3&2&0.500\\ \hline 4&2&0.458\\ \hline 5&3&0.433 \end{array}$ Assume a hypothetical situation as follows:

A man is looking for a single wife to spend the rest of his life with and propagate future generations with. A man and a woman may only be married to one person at a time as these should be monogamous relationships. A house exists where all the women from the story are looking to be married. One male suitor enters and interviews each woman (aka, each set element) one at a time. He will walk from room to room in a random order. He will try to pick the best wife for him knowing only the relative ranking of that woman to the women he interviewed previously and not knowing the overall ranking WRT all the candidates. However, once he says no to a possible wife, he may not recall his decision and is not allowed to choose anyone from before.

Combinatorics and Total Possibilities

We have 15 possible suitors for marriage, 9 women and 6 men, 4 are to get married. Thus we have a total of $\dbinom{9}{4}\dbinom{6}{4}=1,890$ possible outcomes and 54 possible couples.

Before we continue, I will try to put this in simple terms through a "function analogy". For each man, there is a best wife and for each wife, there is a best husband. However, due to there being more women than men, we must start thinking in terms of a non-linear function. Define the 9th degree function $y=f(x)$ where $x\epsilon W(w_i)$ with 4 real roots, represent the function pairing women with men. Each root of this polynomial represent a successful marriage (the wife loves the husband and the husband loves the wife). Since this function isn't surjective, for each woman, there is only only 1 man right for her. However, for men, all of them have only one right wife for him, but half are best for two women. These are the 5 imaginary roots, women who either aren't right for any man or are right for a man but he does not marry as only 4 of the 6 men choose a wife.

Let's assume that the men chose at random. Then the probability that 1 gets the correct wife is

The probability that each man gets the correct wife is statistically

$\dfrac{1}{\dbinom{9}{4}}=\dfrac{1}{126}\approx 0.00794$.

Our goal is to maximize this probability and in the worst case have it be $> \dfrac{1}{126}$.

Getting Down and Dirty With Math

Some math yields us

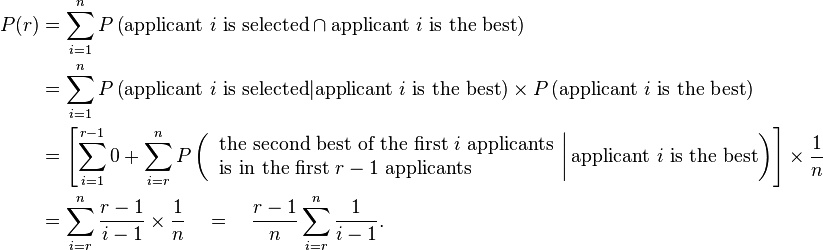
image credit Wikipedia

Our formula that we obtained is ($P(r)$ is the probability of 4 successful marriage.)

$P(r)=\dfrac{r-1}{n}\displaystyle \sum_{i=r}^{n} \dfrac{1}{i-1}$

Let me try to clear up a few complicated things. Wife $r$ is the first wife after the stopping point. AKA, wife $r-1$ is immediately rejected while wife $r$ is wedded assuming that she is better than the first $r-1$ applicants.

We need to solve for a ratio between n and r. If we plug and chug values for $n$ and $r$, we get.

$\begin{array}{l|c|r}\text{n} & \text{r}& \text{P(r)} \\ \hline 1 & 1 &1.00\\ \hline 2&1&0.500 \\ \hline 3&2&0.500\\ \hline 4&2&0.458\\ \hline 5&3&0.433 \end{array}$

Now, say that n tended towards infinity. Let $v$ represent the ratio of $\dfrac{r}{n}$ and $q$ represent the limit of $\dfrac{i}{n}$. Note that initially, $q=1$ but as it tends infinity, it approaches $v$, this is why we have our integral starting at $v$ and ending at 1. That will maximize the probability. Using some nifty approximations, we can see that

$P(v)=v\displaystyle \int_v^1 \dfrac{1}{t} \Bbb{d}t=-v\log(v)$

To maximize this function over the set of reals, we take the derivative and set it equal to 0.

$P'(v)=-v\log(v) \Bbb{d}v$

Using product rule for differentiation.

$P'(v)=-log(v)-1$

Setting this equal to 0 and solving,

$-log(v)-1=0 \longrightarrow v=\dfrac{1}{e}$

We find that as $\displaystyle \lim_{n\rightarrow \infty}$, the optimal value of $\dfrac{n}{r}=\dfrac{1}{e}$. In fact, it can be observed that for all $n$, $r=\lceil\dfrac{n}{e}\rceil$.

Here, we have $n=9, 8,7,6$ after each suitor chooses the correct wife for him. Thus we have our probability that each of the four suitors gets the correct wife for him to be $0.428\cdot 0.414 \cdot 0.410 \cdot 0.406\approx \dfrac{1}{33.9}\approx 0.0295$

As we can clearly see, we have multiplied our original probability of chosing the correct wife for all by 371%. Thus we have accomplished our goal.