Mathematics & Music

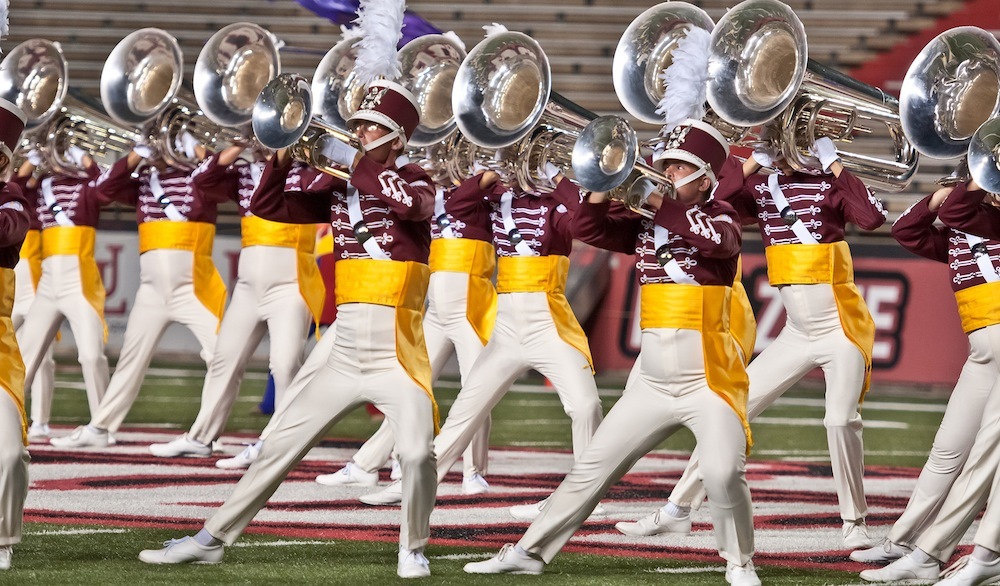
It's no secret that mathematics and music go hand in hand. Frequencies, subdivisions, tempos; it all comes back to mathematics. One of my favorite intersections of mathematics and music is predominantly an American activity: drum corps. A drum corps, for those of you who are unfamiliar with this activity, consists of 100 or so members. There are trumpets, mellophones, baritones, tubas, a large percussion section, and a color guard. These members run around the field playing extremely difficult and entertaining music. The color guard tosses rifles into the air at heights of around 25 feet an catches them with ease! It's a huge step up from the traditional marching band; it's faster, louder, requires true athleticism, and it draws crowds in by the thousands night after night in the summer.

The most mathematical part about drum corps, however, is the drill, or the sets of movements that all the members make. Visual artists from each corps draw inspiration from geometry, especially symmetry. There have been some truly phenomenal shows with delightfully mathematical drill. I'll share some links below to some of my favorites!

Here are a few really cool drum corps shows y'all should check out:

The Cadets, 2011: (here)

The Cadets, 2013: (here)

Carolina Crown, 2012: (here)

Carolina Crown, 2013: (here)

The Blue Devils, 2013: (here)

Do you have a cool intersection of mathematics and music? Play an instrument that makes you think of mathematics? Think drum corps is pretty cool? Have mathematical music or musical mathematics? Share something below!

Markdown

Appears as

*italics* or _italics_

italics

**bold** or __bold__

bold


- bulleted
- list

bulleted
list


1. numbered
2. list

numbered
list

Note: you must add a full line of space before and after lists for them to show up correctly

paragraph 1

paragraph 2

paragraph 1

paragraph 2

[example link](https://brilliant.org)

example link

> This is a quote

This is a quote

    # I indented these lines
    # 4 spaces, and now they show
    # up as a code block.

    print "hello world"

# I indented these lines
# 4 spaces, and now they show
# up as a code block.

print "hello world"

Math

Appears as

Remember to wrap math in $ ... $ or \[ ... \] to ensure proper formatting.

2 \times 3

2 \times 3

2^{34}

2^{34}

a_{i-1}

a_{i-1}

\frac{2}{3}

\frac{2}{3}

\sqrt{2}

\sqrt{2}

\sum_{i=1}^3

\sum_{i=1}^3

\sin \theta

\sin \theta

\boxed{123}

\boxed{123}

Easy Math Editor

This discussion board is a place to discuss our Daily Challenges and the math and science related to those challenges. Explanations are more than just a solution — they should explain the steps and thinking strategies that you used to obtain the solution. Comments should further the discussion of math and science.

When posting on Brilliant:

Use the emojis to react to an explanation, whether you're congratulating a job well done , or just really confused .
Ask specific questions about the challenge or the steps in somebody's explanation. Well-posed questions can add a lot to the discussion, but posting "I don't understand!" doesn't help anyone.
Try to contribute something new to the discussion, whether it is an extension, generalization or other idea related to the challenge.
Stay on topic — we're all here to learn more about math and science, not to hear about your favorite get-rich-quick scheme or current world events.

Markdown	Appears as
`italics` or `_italics_`	italics
`bold` or `__bold__`	bold
- bulleted - list	bulleted list
1. numbered 2. list	numbered list
Note: you must add a full line of space before and after lists for them to show up correctly
paragraph 1 paragraph 2	paragraph 1 paragraph 2
`[example link](https://brilliant.org)`	example link
`> This is a quote`	This is a quote
# I indented these lines # 4 spaces, and now they show # up as a code block. print "hello world"	# I indented these lines # 4 spaces, and now they show # up as a code block. print "hello world"

Math	Appears as
Remember to wrap math in `$` ... `$` or `\[` ... `\]` to ensure proper formatting.
`2 \times 3`	$2 \times 3$
`2^{34}`	$2^{34}$
`a_{i-1}`	$a_{i-1}$
`\frac{2}{3}`	$\frac{2}{3}$
`\sqrt{2}`	$\sqrt{2}$
`\sum_{i=1}^3`	$\sum_{i=1}^3$
`\sin \theta`	$\sin \theta$
`\boxed{123}`	$\boxed{123}$

Comments

Being a percussionist, I'm a big fan of The Blue Devils, so I'm glad there's a fellow fan amongst us.

20th century classical music (Iannis Xenakis' is the most prominent and relevant example) has maths very heavily involved. I play the timpanis; some parts for timpani have a nice structural symmetry to them. I also listen to lots of IDM: Aphex Twin, Squarepusher, and Venetian Snares all have pretty technical tracks to their names.

Also, you link URLs like so:

[text](URL)

Have an example.

Jake Lai - 6 years, 5 months ago

Xenakis definitely comes to mind when I think of math and music! Great stuff.

Ryan Tamburrino - 6 years, 5 months ago

Do you listen to any Steve Reich or John Adams? They're my idols; just made a remix of Different Trains. I don't enjoy Philip Glass or Arvo Part though, their minimalism feels a bit dry.

Also, have you watched any talks by Terry Tao? He describes the PNT poetically as listening to the music of the primes; the von Mangoldt function is "noisy" as primes and "silent" at others, and after subjecting the function to the Mellin transform, notes arise from the function. Some notes can never appear in the music; this is the PNT.

duni ka sabse bada pachchhi kaun hai?

Balaji shanker - 6 years, 5 months ago

well, music can't be composed without mathematics because regularity of beats are nothing but examples of periodic functions like sine,cos graph.nevertheless mathematicians love periodicity because then functions are easy to examine. And mathematicians love to find symmetry and music is nothing but a game of symmetry which give rest to the mathematical brains

Babin Mukherjee - 6 years, 5 months ago

What about aleatoric/stochastic music? What about music in free time? Listen to a bit of John Cage's works or Terry Riley's In C, you'll see what I mean.

Have you heard of overtones? It's what much of most cultures build their harmony on, due to its natural consonance: you take a string with some frequency $f$ . Halve the length and you get an octave up; a third and you get an octave and a perfect fifth from that; a quarter - two octaves; a fifth - two octaves plus a major third; etc.

Succeeding composers like Wagner, Debussy, Ravel, and Mussorgsky who brought about a revolution in sound (melody, timbre, structure, etc), the emancipation of dissonance in the 20th century threw that symmetry out of the window entirely. Go listen to Schoenberg, Ives, Messiaen, Ligeti, or Bartok.

Math	Appears as
Remember to wrap math in `\(` ... `\)` or `\[` ... `\]` to ensure proper formatting.
`2 \times 3`	$2 \times 3$
`2^{34}`	$2^{34}$
`a_{i-1}`	$a_{i-1}$
`\frac{2}{3}`	$\frac{2}{3}$
`\sqrt{2}`	$\sqrt{2}$
`\sum_{i=1}^3`	$\sum_{i=1}^3$
`\sin \theta`	$\sin \theta$
`\boxed{123}`	$\boxed{123}$