Open Question About Minimizing Arc Length

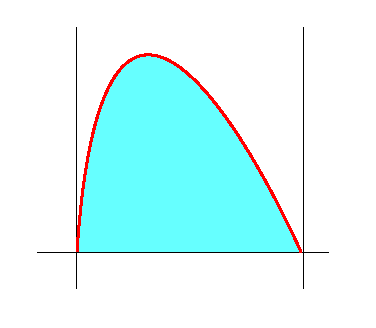
Consider all continuous, (piecewise) differentiable functions $f: [0,1] \rightarrow \mathbb{R}^+$ such that
1) $f(x) \geq 0$ ,
2) $f(0) = 0, f(1) = 0$ ,
3) The area bounded by $f$ and the x-axis, is 1.

What is the smallest possible arc-length $L$ of $f$ from 0 to 1?

I believe that this is an open question, and would be interested in hearing about any thoughts that you have. You might need to apply numerical integration techniques to calculate arc length using calculus

By the iso-perimetric theorem, we know that the curve with the smallest perimeter which has an area of 1, is the circle with radius $\frac{1}{\sqrt{\pi}}$ . This has a perimeter of $2 \sqrt{\pi}$ , and hence the smallest possible arc-length is bounded below by $2 \sqrt{\pi}-1 = 2.545$ . Of course, this doesn't yield a function, nor does it have a straight line segment, so it doesn't qualify. Can you improve this bound?

For an easy upper bound, we can take the $1 \times 1$ square, which has a perimeter of 3. Of course, this strictly speaking isn't a function, but we could approximate it with piecewise linear functions. Can you improve this bound?

We have shown that $2.545 \leq L \leq 3$ . Can you tighten this further? To within $\pm 0.1? \pm 0.01?$

Markdown

Appears as

*italics* or _italics_

italics

**bold** or __bold__

bold


- bulleted
- list

bulleted
list


1. numbered
2. list

numbered
list

Note: you must add a full line of space before and after lists for them to show up correctly

paragraph 1

paragraph 2

paragraph 1

paragraph 2

[example link](https://brilliant.org)

example link

> This is a quote

This is a quote

    # I indented these lines
    # 4 spaces, and now they show
    # up as a code block.

    print "hello world"

# I indented these lines
# 4 spaces, and now they show
# up as a code block.

print "hello world"

Math

Appears as

Remember to wrap math in $ ... $ or \[ ... \] to ensure proper formatting.

2 \times 3

2 \times 3

2^{34}

2^{34}

a_{i-1}

a_{i-1}

\frac{2}{3}

\frac{2}{3}

\sqrt{2}

\sqrt{2}

\sum_{i=1}^3

\sum_{i=1}^3

\sin \theta

\sin \theta

\boxed{123}

\boxed{123}

Easy Math Editor

This discussion board is a place to discuss our Daily Challenges and the math and science related to those challenges. Explanations are more than just a solution — they should explain the steps and thinking strategies that you used to obtain the solution. Comments should further the discussion of math and science.

When posting on Brilliant:

Use the emojis to react to an explanation, whether you're congratulating a job well done , or just really confused .
Ask specific questions about the challenge or the steps in somebody's explanation. Well-posed questions can add a lot to the discussion, but posting "I don't understand!" doesn't help anyone.
Try to contribute something new to the discussion, whether it is an extension, generalization or other idea related to the challenge.
Stay on topic — we're all here to learn more about math and science, not to hear about your favorite get-rich-quick scheme or current world events.

Markdown	Appears as
`italics` or `_italics_`	italics
`bold` or `__bold__`	bold
- bulleted - list	bulleted list
1. numbered 2. list	numbered list
Note: you must add a full line of space before and after lists for them to show up correctly
paragraph 1 paragraph 2	paragraph 1 paragraph 2
`[example link](https://brilliant.org)`	example link
`> This is a quote`	This is a quote
# I indented these lines # 4 spaces, and now they show # up as a code block. print "hello world"	# I indented these lines # 4 spaces, and now they show # up as a code block. print "hello world"

Math	Appears as
Remember to wrap math in `$` ... `$` or `\[` ... `\]` to ensure proper formatting.
`2 \times 3`	$2 \times 3$
`2^{34}`	$2^{34}$
`a_{i-1}`	$a_{i-1}$
`\frac{2}{3}`	$\frac{2}{3}$
`\sqrt{2}`	$\sqrt{2}$
`\sum_{i=1}^3`	$\sum_{i=1}^3$
`\sin \theta`	$\sin \theta$
`\boxed{123}`	$\boxed{123}$

Comments

No calculus of variations is necessary, once we recognize that any curved segment of this function has to be a segment of a circle. So, for symmetry reasons, we first presume that it could be an arc of a circle of some radius $r$ , spanning from $x = 0$ to $x = 1$ , where $f(0) = f(1)$ , so that the total length is $f(0) + f(1) +$ the length of the arc of the circle. When worked out, given the parameter $r$ and that the area must be $1$ , the expression for $L$ is: $\frac { 1 }{ 2 } (4+\sqrt { 4{ r }^{ 2 }-1 } -4r(r-1)ArcCot(\sqrt { 4{ r }^{ 2 }-1 } ))$ Even calculus isn't needed, since for any $r < 1/2$ , it becomes imaginary, and that's the least real value this expression can have. The curve of least arc length is nothing more than a semicircle raised above $y = 0$ such that the area under it is $1,$ and which the total arc length works out to $2+\frac { \pi }{ 4 } =2.7854...$

Michael Mendrin - 7 years ago

"once we recognize that any curved segment of this function has to be a segment of a circle". How do we know that ?

Abhishek Sinha - 7 years ago

Check out "isoperimetric theorem", which says, among other things, "among all curves of fixed length with fixed endpoints, a circular arc encloses a maximum area between it and the line joining its endpoints". Draw a line from $f(0)$ to $f(1)$ , and let's assume that the curve doesn't intersect that line. If the curve of minimum length given bounded area is something other than an arc of a circle, it's not hard to show that there could exist an oval other than a circle that has less length of perimeter than a circle, given an enclosed area.

@Michael Mendrin – Thanks! Didn't know that. Was thinking about cycloid.

Vijay Raghavan - 7 years ago

That's another way of defining curvature.

Milly Choochoo - 7 years ago

I believe that to be the optimum case; placing a semicircle on top of a rectangle of height $1 - \frac{\pi}{8}$ . Note that you still have to check out various other cases, like when we have other straight line segments.

Note that what you defined isn't a function, so we'd likely need limiting cases to it. I believe that we can get approximately close to, but not equal to, that value. That would also be interesting to study.

Calvin Lin Staff - 7 years ago

It's also not hard to show that if the path starts out as a vertical line before going off in another direction, the point where it leaves the straight part is going to be a tangent, not a discontinuity. Right away, then you know the path has to be a semi-circle, spaced above $y=0$ .

As a matter of fact, I've just now posted a problem related to this: The Stingy Rancher...

What about the super-trivial stretching of the non-function circle segment (that we'd want) into a half ellipse that satisfies your conditions?

Here is the equation for an ellipse of major axis ( $a$ ) $\frac{1}{2}$ and minor axis ( $b$ ) $\frac{2}{\pi}$ . Choosing those came from knowing that the area of an ellipse is $\pi ab$ .

$f(x) = \frac{2}{\pi}\sqrt{1-4(x-\frac{1}{2})^2}$

The area above the x-axis is the $1$ , and the perimeter of half of the ellipse plus the line from $0$ to $1$ is approximately $2.791853$ .

I've read the comments here, and this seems to be a much nicer function (in the reals) than the others.

An elliptical arc won't yield the shortest arc length, given a fixed enclosed area.

I'd most certainly say that also. The reason I put that was because on every other suggestion that I've seen here so far, I see that Calvin has said it isn't actually a function.

It seems to me that minimizing the average curvature is the key here. Also, nice 'Stingy Rancher' problem Michael.

How about a Calculus of Variation based approach ?

Woah that is so cool I've never even though about that. :O

Finn Hulse - 7 years ago

It is also easy to reduce the upper bound to $\approx 2.683$ . For this consider a segment of a circle passing through the two end points with its radius chosen such that the enclosed area is $1$ .

I believe that doesn't give us a function.

Math	Appears as
Remember to wrap math in `\(` ... `\)` or `\[` ... `\]` to ensure proper formatting.
`2 \times 3`	$2 \times 3$
`2^{34}`	$2^{34}$
`a_{i-1}`	$a_{i-1}$
`\frac{2}{3}`	$\frac{2}{3}$
`\sqrt{2}`	$\sqrt{2}$
`\sum_{i=1}^3`	$\sum_{i=1}^3$
`\sin \theta`	$\sin \theta$
`\boxed{123}`	$\boxed{123}$