Proof for area of any regular polygon

This note is a follow up for my circle area proof here

In this note I will prove that the area of any regular polygon can be written as $\frac{ap}{2}$ where a is the anothem and p is the perimeter. I will try my best to make this understandable but it is much easier to understand this proof through video.

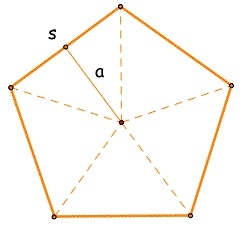
In the picture above, we see that all regular polygons can be divided into congruent triangles. The area of a triangle is of course bh/2. However, the triangle's height is equal to the apothem of the original polygon. Thus we have a(b/2).

Now, if we look at all the bases of the triangles, they add up to the perimeter of the original polygon. So the total area of the original polygon is the total area of all the triangles which is $a\frac{bn}{2}$ where n is the number of sides.

Finally, since bn= the perimeter of the polygon, we arrive at the conclusion that $\frac{ap}{2}$ is the area of the original polygon.

Thank you for the challenge @JubayerNirjhor: In my next note, I will prove that the area of any regular polygon can be represented as

$\dfrac{1}{4} na^2\cot\dfrac{\pi}{n}$ . Where n is the number of sides and a is the side length. You can find it here

Markdown

Appears as

*italics* or _italics_

italics

**bold** or __bold__

bold


- bulleted
- list

bulleted
list


1. numbered
2. list

numbered
list

Note: you must add a full line of space before and after lists for them to show up correctly

paragraph 1

paragraph 2

paragraph 1

paragraph 2

[example link](https://brilliant.org)

example link

> This is a quote

This is a quote

    # I indented these lines
    # 4 spaces, and now they show
    # up as a code block.

    print "hello world"

# I indented these lines
# 4 spaces, and now they show
# up as a code block.

print "hello world"

Math

Appears as

Remember to wrap math in $ ... $ or \[ ... \] to ensure proper formatting.

2 \times 3

2 \times 3

2^{34}

2^{34}

a_{i-1}

a_{i-1}

\frac{2}{3}

\frac{2}{3}

\sqrt{2}

\sqrt{2}

\sum_{i=1}^3

\sum_{i=1}^3

\sin \theta

\sin \theta

\boxed{123}

\boxed{123}

Easy Math Editor

This discussion board is a place to discuss our Daily Challenges and the math and science related to those challenges. Explanations are more than just a solution — they should explain the steps and thinking strategies that you used to obtain the solution. Comments should further the discussion of math and science.

When posting on Brilliant:

Use the emojis to react to an explanation, whether you're congratulating a job well done , or just really confused .
Ask specific questions about the challenge or the steps in somebody's explanation. Well-posed questions can add a lot to the discussion, but posting "I don't understand!" doesn't help anyone.
Try to contribute something new to the discussion, whether it is an extension, generalization or other idea related to the challenge.
Stay on topic — we're all here to learn more about math and science, not to hear about your favorite get-rich-quick scheme or current world events.

Markdown	Appears as
`italics` or `_italics_`	italics
`bold` or `__bold__`	bold
- bulleted - list	bulleted list
1. numbered 2. list	numbered list
Note: you must add a full line of space before and after lists for them to show up correctly
paragraph 1 paragraph 2	paragraph 1 paragraph 2
`[example link](https://brilliant.org)`	example link
`> This is a quote`	This is a quote
# I indented these lines # 4 spaces, and now they show # up as a code block. print "hello world"	# I indented these lines # 4 spaces, and now they show # up as a code block. print "hello world"

Math	Appears as
Remember to wrap math in `$` ... `$` or `\[` ... `\]` to ensure proper formatting.
`2 \times 3`	$2 \times 3$
`2^{34}`	$2^{34}$
`a_{i-1}`	$a_{i-1}$
`\frac{2}{3}`	$\frac{2}{3}$
`\sqrt{2}`	$\sqrt{2}$
`\sum_{i=1}^3`	$\sum_{i=1}^3$
`\sin \theta`	$\sin \theta$
`\boxed{123}`	$\boxed{123}$

Comments

In most circumstances, people don't know the length of the apothem, but the length of a side. So it's useful to have an area formula with side length and number of sides argument.

If $n$ is the number of sides and $a$ is the length of one side, then the formula is $\dfrac{1}{4} na^2\cot\dfrac{\pi}{n}$ . (Prove it)

Jubayer Nirjhor - 6 years, 9 months ago

I will try. But trig is not my strong suit.

Trevor Arashiro - 6 years, 9 months ago

Got it! It was a fun proof to do, took me about 10 mins because I was thinking of it the wrong way (trying to use identities). I'll post the proof in just a sec.

@Trevor Arashiro Can you add this discussion (along with part 2) to the Regular Polygons - Area wiki page? Thanks!

Calvin Lin Staff - 6 years, 8 months ago

Math	Appears as
Remember to wrap math in `\(` ... `\)` or `\[` ... `\]` to ensure proper formatting.
`2 \times 3`	$2 \times 3$
`2^{34}`	$2^{34}$
`a_{i-1}`	$a_{i-1}$
`\frac{2}{3}`	$\frac{2}{3}$
`\sqrt{2}`	$\sqrt{2}$
`\sum_{i=1}^3`	$\sum_{i=1}^3$
`\sin \theta`	$\sin \theta$
`\boxed{123}`	$\boxed{123}$