Quantum Computing 1 -- The qubit

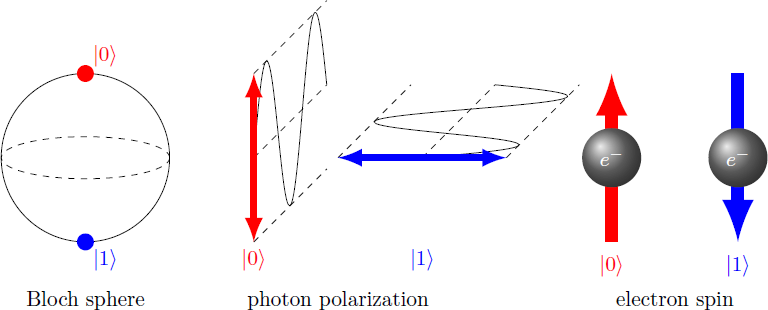
The qubit is the smallest storage unit in the quantum computer and represents the simplest nontrivial quantum system. The qubit can assume one of two distinguishable states. These eigenstates of the qubit are denoted by $| 0 \rangle$ and $| 1 \rangle$ and correspond to the two setting possibilities of a classical bit. They can also be represented as two-dimensional unit vectors $|0\rangle = \left( \begin{array}{c} 1 \\ 0 \end{array} \right), \quad |1\rangle = \left( \begin{array}{c} 0 \\ 1 \end{array} \right)$ In contrast to the classical bit, the qubit can also assume a superposition of the states $| 0 \rangle$ and $| 1 \rangle$. The general state $| \psi \rangle$ of the qubit is therefore a linear combination of the two eigenstates $|\psi\rangle = \alpha |0\rangle + \beta |1 \rangle = \left( \begin{array}{c} \alpha \\ \beta \end{array} \right), \quad \alpha, \beta \in \mathbb{C}$ The vectors $| 0 \rangle$ and $| 1 \rangle$ thus form a basis of the vector space. The state $| \psi \rangle$ is characterized by the two complex numbers $\alpha$ and $\beta$.

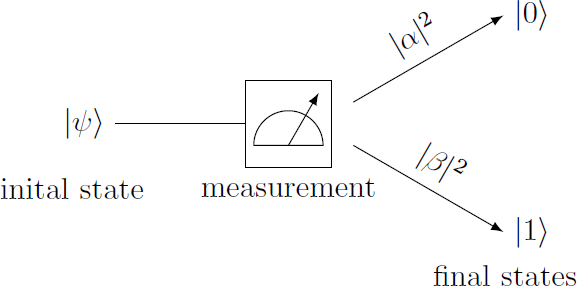
If the qubit is in such a superposition and you take a measurement, you get a random result. The probability of finding the qubit in the state $| 0 \rangle$ or $| 1 \rangle$ is proportional to the absolute square $| \alpha | ^ 2$ or $| \beta | ^ 2$ of the respective pre-factor. After the measurement, the qubit is in one of the eigenstates $| 0 \rangle$ or $| 1 \rangle$, so that the information about the original state is lost (wave function collapse)

$\begin{aligned} \text{before measurement:} & & |\psi \rangle &= \alpha |0 \rangle + \beta |1\rangle \\ \text{after measurement:} & & |\psi'\rangle &= \begin{cases} |0 \rangle & \text{with probability } \frac{|\alpha|^2}{|\alpha|^2 + |\beta|^2} \\ |1 \rangle & \text{with probability } \frac{|\beta|^2}{|\alpha|^2 + |\beta|^2} \end{cases} \end{aligned}$ Usually, state vectors will be normalized (i.e. $| \alpha | ^ 2 + | \beta | ^ 2 = 1$), so the probabilities for the states $| 0 \rangle$ and $| 1 \rangle$ are given directly by absolute squares $| \alpha | ^ 2$ and $| \beta | ^ 2$. In addition, a phase factor can be chosen freely, so that the qubit can be described by two real numbers $\theta$ and $\varphi$: $|\psi\rangle = \cos \frac{\theta}{2} |0\rangle + e^{i \varphi} \sin \frac{\theta}{2}|1\rangle$ If one interprets these numbers $\theta$ and $\varphi$ as angles, the qubit can be represented as a point on a sphere surface (Bloch sphere).

A qubit in the normalized state $|\psi\rangle = \frac{\sqrt{3}}{2} |0\rangle + \frac{1}{2} |1\rangle$ is measured. What are the probabilities of the two different outcomes $| 0 \rangle$ and $| 1 \rangle$?

---

$\begin{aligned} |0\rangle & \quad \text{ with probability } \left|\frac{\sqrt{3}}{2}\right|^2 = \frac{3}{4} = 75\,\%,\\ |1\rangle & \quad \text{ with probability } \left|\frac{1}{2}\right|^2 = \frac{1}{4} = 25\,\% \end{aligned}$

Conclusion: The state of a qubit is described by a two-dimensional vector, so that a qubit contains much more information than a classical bit. However, if the qubit is read out, most information is deleted, so that the result is only one of the two states $| 0 \rangle$ or $| 1 \rangle$. The readable part of the quantum information therefore only corresponds to one classical bit. However, the full quantum information be can used for quantum operations, as we will see later.