Resistance Measurement
Here is a problem that was recently sent to me:
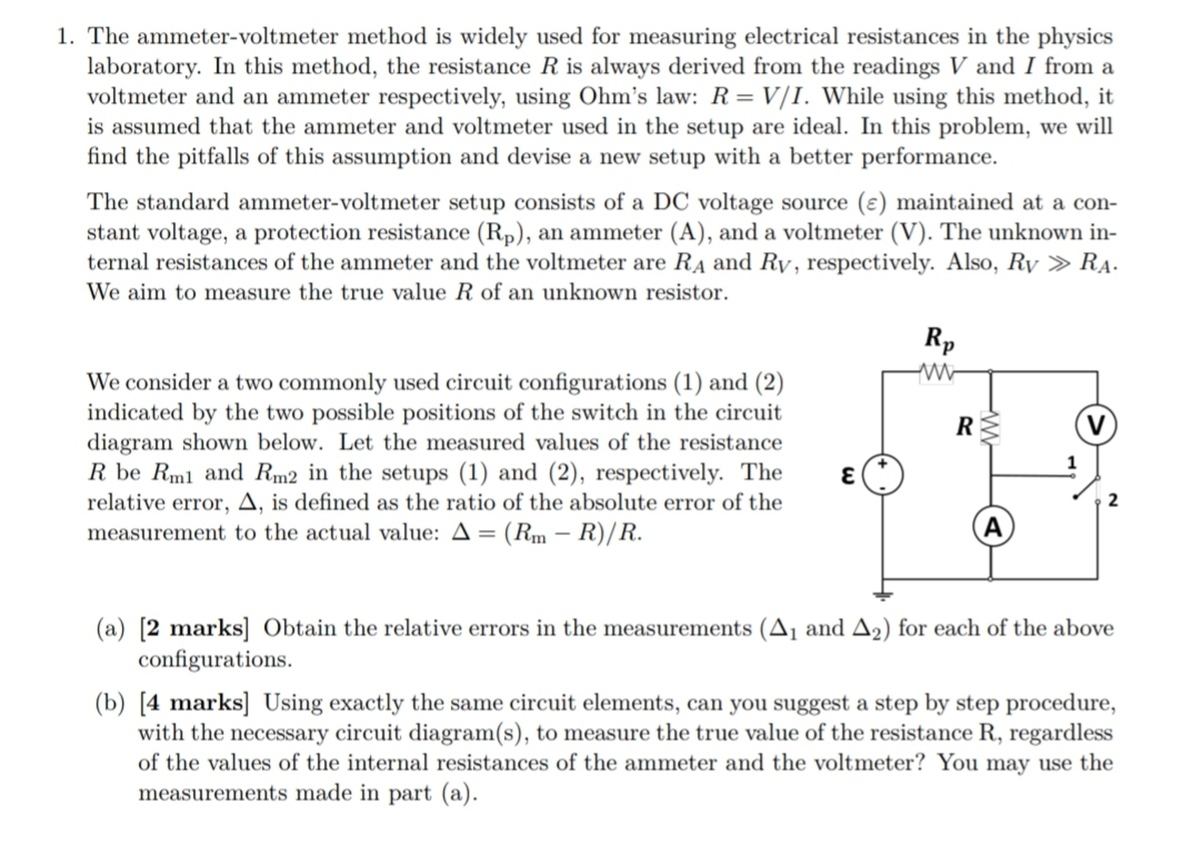
To solve, I will refer to the following four configurations. The first two are the ones presented in the problem (with the switches in different state), and the third and fourth are more useful configurations.
Configuration 1:
The voltage across the voltmeter can be expressed in terms of the current through the ammeter:
The measured resistance in configuration 1 is therefore:
Configuration 2:
The current through the ammeter can be expressed in terms of the voltage across the voltmeter:
The measured resistance in configuration 2 is therefore:
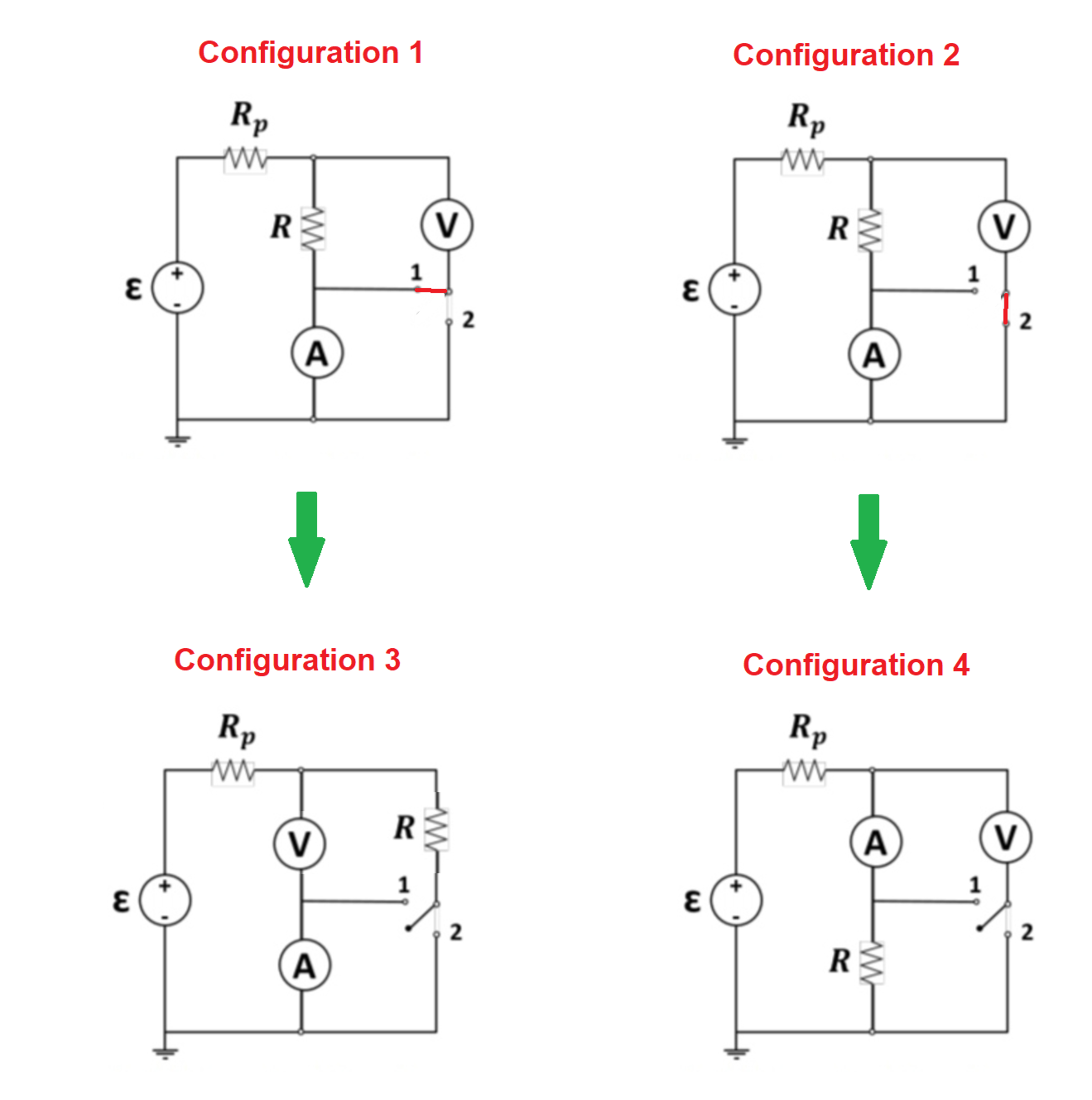
There are two ways to answer part (b) of the question: using either configuration 3 or configuration 4.
Configuration 3:
Configuration 3 is a more useful form of configuration 1. Starting with the switch in position 2, we know the current through as well as the voltage across it, which gives us . Then with the switch in position 1, we get (which is the same result from configuration 1). And since the first measurement gave us , we can solve for .
Configuration 4:
Configuration 4 is a more useful form of configuration 2. Starting with the switch in position 1, we know the current through as well as the voltage across it, which gives us . Then with the switch in position 2, we get (which is the same result from configuration 2). And since the first measurement gave us , we can solve for .
Easy Math Editor
This discussion board is a place to discuss our Daily Challenges and the math and science related to those challenges. Explanations are more than just a solution — they should explain the steps and thinking strategies that you used to obtain the solution. Comments should further the discussion of math and science.
When posting on Brilliant:
*italics*or_italics_**bold**or__bold__paragraph 1
paragraph 2
[example link](https://brilliant.org)> This is a quote# I indented these lines # 4 spaces, and now they show # up as a code block. print "hello world"\(...\)or\[...\]to ensure proper formatting.2 \times 32^{34}a_{i-1}\frac{2}{3}\sqrt{2}\sum_{i=1}^3\sin \theta\boxed{123}Comments
@Talulah Riley Here is my answer to the problem
This is the first problem of InPho!
Log in to reply
Did I get it right?
Log in to reply
I think you did!, I had no clue about how to do the second part, and spent a lot of time getting an accurate error for the first part (some current goes into the voltmeter, that thought messed me up, I ended up obtaining a huge expression for error even after using binomial approximations multiple times)
Log in to reply
The problem doesn't do a great job explaining what assumptions you are allowed to make. That annoyed me a bit
Log in to reply
I assume minimal assumptions was best, also could I ask you the solution for another problem of the same paper
Log in to reply
I can take a look, but I can't promise that I'll be able to solve it
Log in to reply
I assumed this would be easy, but got stuck in middle when I had to perform an integration between time and distance, I am now unclear of how to approach this now
Log in to reply
@Jason Gomez I think you have probably stucked in part (c).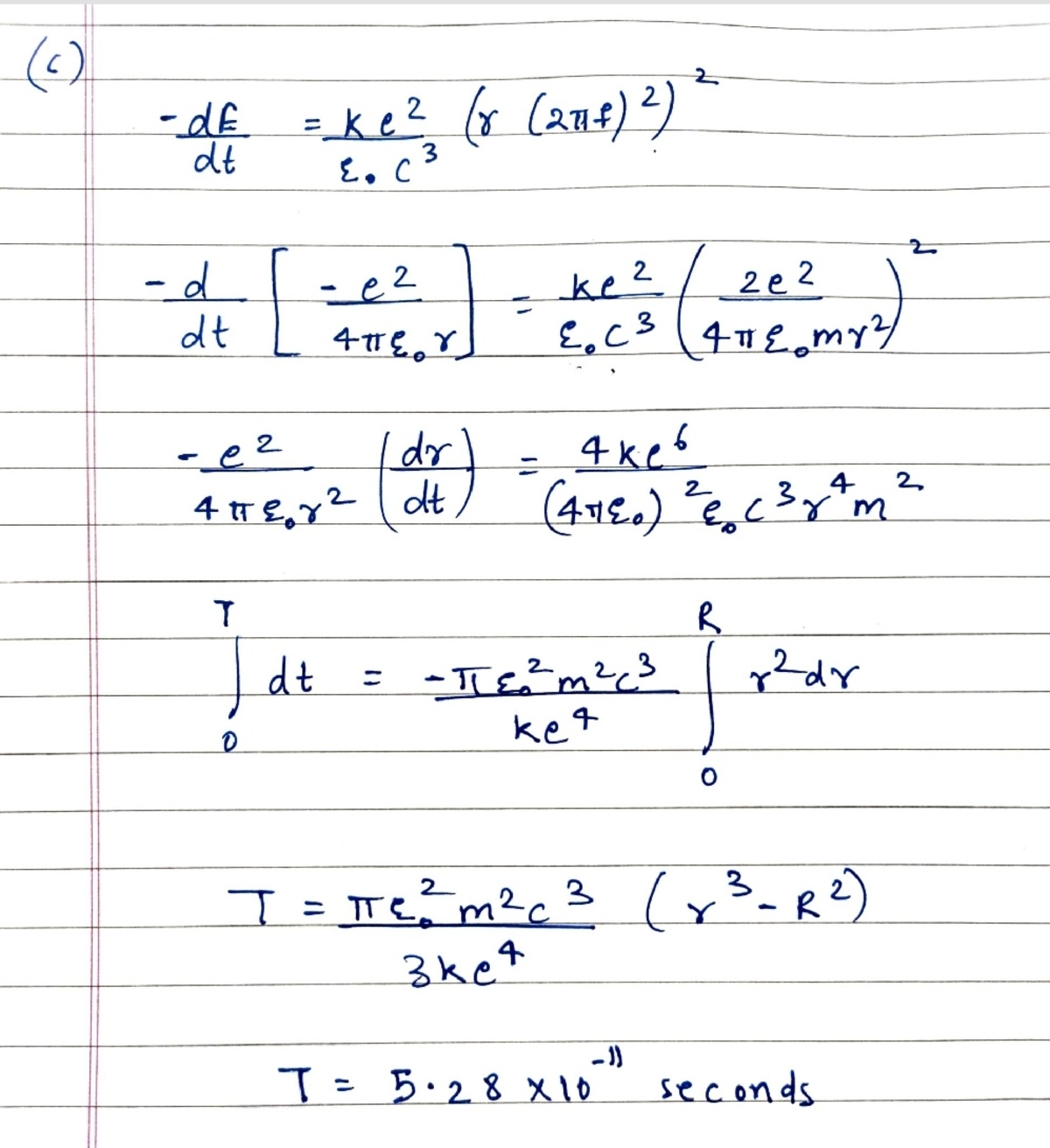
Here is the solution:
Log in to reply
Thanks a lot! But how is the factor (γ(2πf)2)=a (and what is γ?)
Log in to reply
Oh nevermind, just realised that’s an r
Also why is the acceleration taken as only centripetal acceleration?
Thank you for trying, even if you get it or not (I hope you do)
Log in to reply
OK, it's just about bed time on my side of the planet, so I'll try tomorrow.
Log in to reply
It’s fine even if you give the answer a week later
@Steven Chase Thanks ,you rocked , your answers are correct.
At last did you get any quadratic equation for R which you have not written in your solution ?
@Steven Chase
@Steven Chase i have posted a new discussion on mechanis. Please solve that problem.