The Feynman Point!

Have you ever seen or heard of someone who has memorized a lot of digits of $\pi$ ? Have you ever tried doing that in your life?

Whatever your answers are, there is another question that is related to all of this: why on earth would anyone want to do that? What is the motivation behind it?

I have a good answer for that.

The Feynman Point.

What? I know most of you are now scratching your head. Well, stop scratching and take a look at what Wikipedia has to say about this:

The Feynman point is a sequence of six $9$ 's that begins at the $762$ nd decimal place of the decimal representation of $\pi$ . It is named after physicist Richard Feynman, who once stated during a lecture he would like to memorize the digits of $\pi$ until that point, so he could recite them and quip "nine nine nine nine nine nine and so on", suggesting, in a tongue-in-cheek manner, that $\pi$ is rational.

I can't speak for anyone else, but to me, that is one of the coolest [if not the coolest] motivations behind memorizing the digits of $\pi$ .

So, if you're interested, take out a list containing the first thousand digts of $\pi$ and start memorizing!

Last but not the least, whenever I write something about the digits of $\pi$ , I can never resist putting this comic in it. It's funny because it's true!

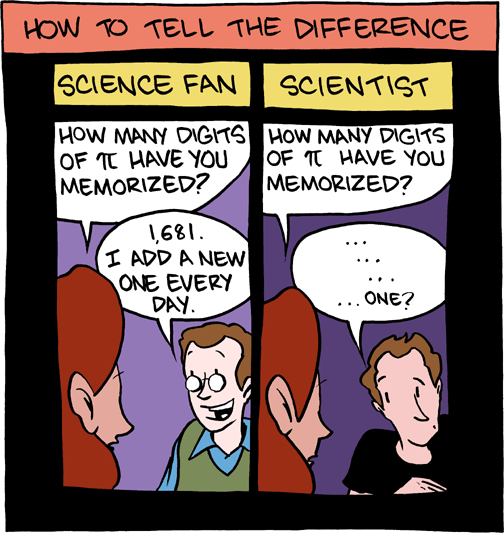Alt text

The comic was taken from here.

Markdown

Appears as

*italics* or _italics_

italics

**bold** or __bold__

bold


- bulleted
- list

bulleted
list


1. numbered
2. list

numbered
list

Note: you must add a full line of space before and after lists for them to show up correctly

paragraph 1

paragraph 2

paragraph 1

paragraph 2

[example link](https://brilliant.org)

example link

> This is a quote

This is a quote

    # I indented these lines
    # 4 spaces, and now they show
    # up as a code block.

    print "hello world"

# I indented these lines
# 4 spaces, and now they show
# up as a code block.

print "hello world"

Math

Appears as

Remember to wrap math in $ ... $ or \[ ... \] to ensure proper formatting.

2 \times 3

2 \times 3

2^{34}

2^{34}

a_{i-1}

a_{i-1}

\frac{2}{3}

\frac{2}{3}

\sqrt{2}

\sqrt{2}

\sum_{i=1}^3

\sum_{i=1}^3

\sin \theta

\sin \theta

\boxed{123}

\boxed{123}

Easy Math Editor

This discussion board is a place to discuss our Daily Challenges and the math and science related to those challenges. Explanations are more than just a solution — they should explain the steps and thinking strategies that you used to obtain the solution. Comments should further the discussion of math and science.

When posting on Brilliant:

Use the emojis to react to an explanation, whether you're congratulating a job well done , or just really confused .
Ask specific questions about the challenge or the steps in somebody's explanation. Well-posed questions can add a lot to the discussion, but posting "I don't understand!" doesn't help anyone.
Try to contribute something new to the discussion, whether it is an extension, generalization or other idea related to the challenge.
Stay on topic — we're all here to learn more about math and science, not to hear about your favorite get-rich-quick scheme or current world events.

Markdown	Appears as
`italics` or `_italics_`	italics
`bold` or `__bold__`	bold
- bulleted - list	bulleted list
1. numbered 2. list	numbered list
Note: you must add a full line of space before and after lists for them to show up correctly
paragraph 1 paragraph 2	paragraph 1 paragraph 2
`[example link](https://brilliant.org)`	example link
`> This is a quote`	This is a quote
# I indented these lines # 4 spaces, and now they show # up as a code block. print "hello world"	# I indented these lines # 4 spaces, and now they show # up as a code block. print "hello world"

Math	Appears as
Remember to wrap math in `$` ... `$` or `\[` ... `\]` to ensure proper formatting.
`2 \times 3`	$2 \times 3$
`2^{34}`	$2^{34}$
`a_{i-1}`	$a_{i-1}$
`\frac{2}{3}`	$\frac{2}{3}$
`\sqrt{2}`	$\sqrt{2}$
`\sum_{i=1}^3`	$\sum_{i=1}^3$
`\sin \theta`	$\sin \theta$
`\boxed{123}`	$\boxed{123}$

Comments

That's nothing! I remembered ALL the digits of the largest known prime number. Here it goes:

$\large \underbrace{111111111111111111111111111111111111111111111111111 \ldots 1}_{\text{fifty-seven million eight hundred eighty-five thousand one hundred sixty-one } 1 \text{'s} }$

in base 2.

Pi Han Goh - 7 years, 1 month ago

Show off! :)

For those who don't know what @Pi Han Goh is talking about: there are certain primes that are off the form $2^p-1$ where $p$ is a prime number. These prime numbers are called Mersenne primes. Mersenne primes tend to get really big really fast and it's not hard to see why. In fact the largest known prime number is a Mersenne prime, $2^{57885161}-1$ where $57885161$ is a prime number. And everyone knows that $2^k-1$ is just $k$ $1$ 's in binary.

Mursalin Habib - 7 years, 1 month ago

I saw that on Numberphile he's like "I memorized it all... what's more, in base 2!"

"No wayyyyy that's impossible"

"111111..."

"Oh ok that's hilarious"

Spock Weakhypercharge - 7 years, 1 month ago

I memorized 130 digits in an afternoon for a pi day competition at my school. The next day I learn that I'm going to seattle for a soccer tournament instead.😫

Robert Fritz - 7 years, 1 month ago

I've memorized about 400 digits. I had a really boring math class in fifth grade and I sat next to the giant pi poster my teacher had in her room.

Trevor B. - 7 years, 1 month ago

I remember the value of $\pi$ upto 3.14159265358979, which is because it was mentioned in a movie "Night at the Museum 2"....

Shabarish Ch - 7 years, 1 month ago

I memorised up to 314 digits for pi day.

Sharky Kesa - 7 years, 1 month ago

You're too good. I give up...

That was my first thought too. Do you remember the Einstein bobble-heads in the movie?

Pooja Priya - 7 years, 1 month ago

I remember them, but I forgot their significance in the movie..

I have memorized the whole continued fraction for the most irrational number.. $[1,1,1,1,1,1,....]$ . Isn't that much more coooooooool than $\pi$ ?

Snehal Shekatkar - 7 years, 1 month ago

Oh, I have memorized only 41! ♪`~

Swapnil Das - 6 years ago

@Mursalin Habib, that's a great note. But would you tell me how did you make the Wikipedia article look like that? (I mean the formatting & the code you used)

Ameya Salankar - 7 years, 1 month ago

Math	Appears as
Remember to wrap math in `\(` ... `\)` or `\[` ... `\]` to ensure proper formatting.
`2 \times 3`	$2 \times 3$
`2^{34}`	$2^{34}$
`a_{i-1}`	$a_{i-1}$
`\frac{2}{3}`	$\frac{2}{3}$
`\sqrt{2}`	$\sqrt{2}$
`\sum_{i=1}^3`	$\sum_{i=1}^3$
`\sin \theta`	$\sin \theta$
`\boxed{123}`	$\boxed{123}$