Uniform ring's axis

Suppose a circular ring of uniform mass density and having mass $M$ and radius $R$ .

Now suppose a point mass (in rest) of mass $m$ initially at a distance of $a$ from its center lying on it's axis as shown below :

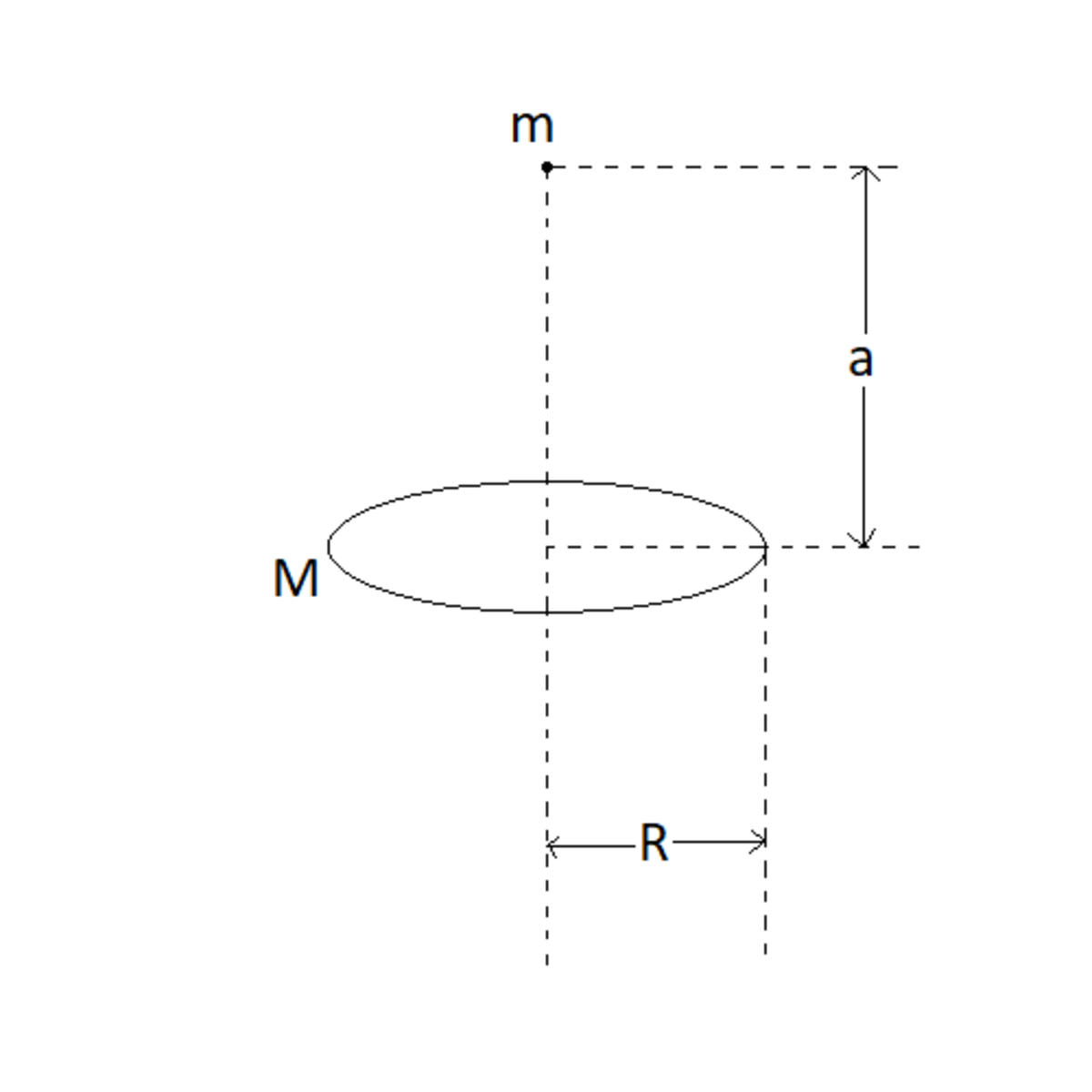
What will be the distance of the point mass from the center (measuring positive above while negative for below) after time $t$ have passed assuming that $M>>m$ and only the forces of gravity of the ring and point masses are involved?

My work :

Acceleration of ring due to the gravity of point mass = $0$ $(\because M>>m)$

If $v$ is the velocity of the point mass and $U$ as it's gravitational potential energy, then $U_{}=\int-\frac{GmdM}{\sqrt{a^2+R^2}}=-\frac{Gm}{\sqrt{a^2+R^2}}\int dM=-\frac{GmM}{\sqrt{a^2+R^2}}$ Now, if the point mass have moved from a distance of $a$ from the center to a distance $b$ then from Work energy Theorem we have : $\frac{1}{2}\cancel{m}(v^2-u^2)=-\triangle U = G\cancel{m}M(\frac{1}{\sqrt{b^2+R^2}}-\frac{1}{\sqrt{a^2+R^2}})$ Now as it is given that $u=0$ i.e. the point mass it at rest initially $\therefore v^2=2GM(\frac{1}{\sqrt{b^2+R^2}}-\frac{1}{\sqrt{a^2+R^2}})$ $\Rightarrow |v|=abs(\frac{db}{dt})=\sqrt{2GM(\frac{1}{\sqrt{b^2+R^2}}-\frac{1}{\sqrt{a^2+R^2}})}$ $\Rightarrow \triangle t={^+_-}\int \frac{1}{\sqrt{2GM(\frac{1}{\sqrt{b^2+R^2}}-\frac{1}{\sqrt{a^2+R^2}})}}db$ Now I just can't solve the integral. The integral can also be written as : $\triangle t={^+_-}\int\frac{1}{\sqrt{\frac{k}{\sqrt{b^2+R^2}}-c}}db$ Where $k=2GM$ and $c=\frac{2GM}{\sqrt{a^2+R^2}}$

Can someone solve this integral, or may have any other way of solving this?

Markdown

Appears as

*italics* or _italics_

italics

**bold** or __bold__

bold


- bulleted
- list

bulleted
list


1. numbered
2. list

numbered
list

Note: you must add a full line of space before and after lists for them to show up correctly

paragraph 1

paragraph 2

paragraph 1

paragraph 2

[example link](https://brilliant.org)

example link

> This is a quote

This is a quote

    # I indented these lines
    # 4 spaces, and now they show
    # up as a code block.

    print "hello world"

# I indented these lines
# 4 spaces, and now they show
# up as a code block.

print "hello world"

Math

Appears as

Remember to wrap math in $ ... $ or \[ ... \] to ensure proper formatting.

2 \times 3

2 \times 3

2^{34}

2^{34}

a_{i-1}

a_{i-1}

\frac{2}{3}

\frac{2}{3}

\sqrt{2}

\sqrt{2}

\sum_{i=1}^3

\sum_{i=1}^3

\sin \theta

\sin \theta

\boxed{123}

\boxed{123}

Easy Math Editor

This discussion board is a place to discuss our Daily Challenges and the math and science related to those challenges. Explanations are more than just a solution — they should explain the steps and thinking strategies that you used to obtain the solution. Comments should further the discussion of math and science.

When posting on Brilliant:

Use the emojis to react to an explanation, whether you're congratulating a job well done , or just really confused .
Ask specific questions about the challenge or the steps in somebody's explanation. Well-posed questions can add a lot to the discussion, but posting "I don't understand!" doesn't help anyone.
Try to contribute something new to the discussion, whether it is an extension, generalization or other idea related to the challenge.
Stay on topic — we're all here to learn more about math and science, not to hear about your favorite get-rich-quick scheme or current world events.

Markdown	Appears as
`italics` or `_italics_`	italics
`bold` or `__bold__`	bold
- bulleted - list	bulleted list
1. numbered 2. list	numbered list
Note: you must add a full line of space before and after lists for them to show up correctly
paragraph 1 paragraph 2	paragraph 1 paragraph 2
`[example link](https://brilliant.org)`	example link
`> This is a quote`	This is a quote
# I indented these lines # 4 spaces, and now they show # up as a code block. print "hello world"	# I indented these lines # 4 spaces, and now they show # up as a code block. print "hello world"

Math	Appears as
Remember to wrap math in `$` ... `$` or `\[` ... `\]` to ensure proper formatting.
`2 \times 3`	$2 \times 3$
`2^{34}`	$2^{34}$
`a_{i-1}`	$a_{i-1}$
`\frac{2}{3}`	$\frac{2}{3}$
`\sqrt{2}`	$\sqrt{2}$
`\sum_{i=1}^3`	$\sum_{i=1}^3$
`\sin \theta`	$\sin \theta$
`\boxed{123}`	$\boxed{123}$

Comments

@Aryan Sanghi @Mahdi Raza @David Stiff @Kriti Kamal @Kumudesh Ghosh @Marvin Kalngan @Justin Travers @jordi curto

Zakir Husain - 5 months, 4 weeks ago

The gravitational field has a direction. Unless a>>R, I think we need to take account of the angle at m between the central axis and the line between m and the ring.

Justin Travers - 5 months, 3 weeks ago

The direction of the gravitational field will be towards the center of the ring, due to symmetry (in this case). I have used that angle you are talking about, but it's cosine will be used not the angle itself. So I multiplied (\frac{a}{\sqrt{a^2+R^2}} instead of its cosine.

Zakir Husain - 5 months, 3 weeks ago

@Karan Chatrath @Alice Smith @볼트 Bolt @Mikael Marcondes @Logic Alpha @Inquisitor Math @Real Sakib25 @Ram Mohith

There is one problem in your solution. $v \ne \frac{db}{dt}$ . As the particle moves along the axis towards the ring, its speed increases from its initial state of rest. Essentially, as $b$ decreases, $v$ increases. So:

$v = -\frac{db}{dt}$

I don't think I can solve the integral analytically, but I would try $b = R\tan{\theta}$ as a substitution (which most likely does not work) for a start. If I had to solve this, I would proceed numerically, in which case, I would rewrite the integral as a definite integral, but I do not think that is the answer you seek.

If the system is left untouched, the particle would oscillate along the axis of the ring. Finding the time period of oscillation would be a nice problem. I would not be surprised if someone has thought of this already.

Karan Chatrath - 5 months, 3 weeks ago

$v$ is the velocity not speed. As $b$ decreases in first case this meant that velocity is negative i.e. it is directed downwards.
Finding $\triangle t$ is same as to find the time period.

@Zakir Husain – Here, $v$ is in fact speed. You have used kinetic energy which is a function of speed and not velocity. Just taking a square root of the speed squared does not yield velocity.

Moreover if $b$ decreases, then the right hand side of your equation must be negative. This is not the case in your working.

@Karan Chatrath – Hmm... Now is it correct?

@Zakir Husain – Physically speaking, no.

$\because v^2 = \left(\frac{db}{dt}\right) ^2$

$\implies v = \mathrm{abs}\left(\frac{db}{dt}\right)$

Mathematically, I understand this part. This gives rise to two possibilities. As the particle's speed starts to increase, $b$ decreases, or increases. An increase in $b$ would correspond to a violation of Newton's gravitational law. That is why we introduce the negative sign cause $b$ decreases with an increase in speed as the particle starts from rest.

@Karan Chatrath – $\forall x\in \mathbb{R},\sqrt{x^2}=|x|\cancel{=}x$

for example: $\sqrt{(-3)^2}=\sqrt{9}=3\cancel{=}-3$

@Zakir Husain – I understand the mathematics behind your thought. That is why I made a distinction between the mathematical argument and its physical interpretation. I would execute these steps as follows:

$v = \sqrt{\frac{k}{\sqrt{b^2+R^2}} -c}$ $-\frac{db}{dt} = \sqrt{\frac{k}{\sqrt{b^2+R^2}} -c}$

Notice why I introduced the negative sign. The RHS is positive. The LHS must also be so. By writing $v = \frac{db}{dt}$ , I infer that $v$ is a negative quantity, which is not correct. Speed cannot be negative, but it so happens here that as $v$ increases, $b$ decreases. Hence $v = -\frac{db}{dt}$ . $-\frac{db}{\sqrt{\frac{k}{\sqrt{b^2+R^2}} -c}} = dt$ Integrating (introducing a dummy variable $x$ ) from initial state of the point mass:

$t = -\int_{a}^{b}\frac{dx}{\sqrt{\frac{k}{\sqrt{x^2+R^2}} -c}}$

$t = \int_{b}^{a}\frac{dx}{\sqrt{\frac{k}{\sqrt{x^2+R^2}} -c}}$

This, to me is correct as I can physically interpret my steps.

@Zakir Husain

This website shows a different value for the potential energy of the point mass due to the disk: GPE due to uniform density disk

And an IIT video with the same conclusion as the website I mentioned earlier: IIT video

Edit: I thought this post was about a uniform disk before I posted this comment. My bad.

Krishna Karthik - 5 months, 3 weeks ago

@Krishna Karthik The video talks about Uniform Disk which is very different from Uniform Ring. The video in start mentions :

Gravitational potential (due to a Uniform Ring) $(V)=-\frac{GM}{\sqrt{a^2+R^2}}$

and $\therefore$ Gravitational potential energy (due to a Uniform Ring) $(U)=mV=-\frac{GMm}{\sqrt{a^2+R^2}}$

Oh woops... I probably read too lazily and didn't see that it said uniform ring. My bad. It's just that your diagram looks a little like a disk, but I read too carelessly lol

Haha it literally says "Uniform ring's axis" in the title; that totally whooshed over my head lol.

@Zakir Husain I think sir above integral has to be evaluated numerically between the limits. Had $a \lt\lt R$ , then above motion would resemble $S.H.M.$ with

$A = -\frac{GMx}{(R^2 + x^2)^{3/2}} = -\frac{GMx}{R^3} = -\omega^2x$

$\omega = \sqrt{\frac{GM}{R^3}}$

$T = \frac{2\pi}{\omega}$

$\boxed{T = 2\pi\sqrt{\frac{R^3}{GM}}}$

For your question's integration, you could use this Integral Calculator sir

Aryan Sanghi - 5 months, 3 weeks ago

@Aryan Sanghi What you think? Is the integral non - elementary ?

Maybe sir, I can't say.

Aryan Sanghi - 5 months, 2 weeks ago

So here's where I've got to so far. There's a gravitational field along the central axis that points towards the center of the ring on either side. The field is proportional to $Ma/s^3$ where $s=\sqrt{a^2+R^2}$ , the slant distance from $m$ to the ring. Acceleration is proportional to the strength of the field in the direction of the field. Acceleration will increase to a maximum then decrease to zero as $m$ moves towards the center from it's initial location $a_{0}$ . On passing the center, the velocity is maximal. The direction of the field reverses and the velocity of the point mass will start to decrease, reaching zero at $-a_{0}$ . It will then fall back to the center in a symmetric fashion and oscillate indefinitely. I think this means that velocity can be calculated from integrating the acceleration as $1/s+c_{1}$ in the direction of the center. Distance after time might then be calculated as $log{(s)}+c_{2}$ or $\sinh^{-1}(a/R)+c_{2}$ . Still haven't worked out the details.

Justin Travers - 5 months, 2 weeks ago

Math	Appears as
Remember to wrap math in `\(` ... `\)` or `\[` ... `\]` to ensure proper formatting.
`2 \times 3`	$2 \times 3$
`2^{34}`	$2^{34}$
`a_{i-1}`	$a_{i-1}$
`\frac{2}{3}`	$\frac{2}{3}$
`\sqrt{2}`	$\sqrt{2}$
`\sum_{i=1}^3`	$\sum_{i=1}^3$
`\sin \theta`	$\sin \theta$
`\boxed{123}`	$\boxed{123}$