1500 followers problem! For my friend Xuming!
In Δ A B C , m ∠ B = 4 5 ∘ , m ∠ C = 5 4 ∘ . Point O is the circumcenter. Let O E ⊥ A C , O F ⊥ A B . If the ratio O F O E can be expressed as b − b a where a , b ∈ Z + and b is square free,
Find the value of ⌊ a + b 1 5 0 0 ⌋ .
The answer is 214.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
4 solutions
Method 1:
Let H be orthocenter and H L ⊥ A B , H K ⊥ A C . Note that H and O are the isogonal conjugates of each other. By one of the properties of isogonal conjugates ,
\begin{aligned}{\dfrac{OE}{OF} = \dfrac{HL}{HK} \\ = \dfrac{2R\cos A \cos B}{2R\cos A \cos C} \\ = \dfrac{\cos B}{\cos C} \\ = \dfrac{\cos 45^\circ}{\cos 54^\circ} \\ = \dfrac{2}{\sqrt{5-\sqrt{5}}}}\end{aligned} \\ \Rightarrow a+b=2+5=7 \Rightarrow \left \lfloor \dfrac{1500}{7} \right \rfloor = \lfloor 214.28 \rfloor = \boxed{214}
Method 2:
We know that O E = 2 1 ( B H ) = 2 2 R cos B , O F = 2 1 ( C H ) = 2 2 R cos C
Clearly , it follows that O F O E = cos C cos B and compute the required quantities same as in method 1 . :)
It is not true that O E / O F = H L / H K ; since you are dropping the perpendiculars from H , you have to do the same with O . If P is the foot of the perpendicular from O to A C , and Q is the foot of the perpendicular from O to A B , then O P / O Q = H L / H K .
Log in to reply
Sorry , my misconception. I read the page of Isogonal Conjugates from Wolfram Mathworld which did not mention the data properly. Thanks , let me edit the question :)
Did you construct H for the fun of it? because it is really not necessary lol
btw as you have experienced, online resources even the likes of Wolfram may contain incorrect information. For this "property" you learned, you should have tried to prove it yourself first. The topic of Isogonal Conjugates is very interesting and still considered modern and fresh. It is very probable that one could discover some unknown properties involving its configuration(It happened to me).
Log in to reply
Haha , I think I did construct it for fun xD ;P .I have added method 2 also.
I have already proved this property of perpendiculars (Using cyclicity and similarity). Yeah , I got mislead due to inadequate information on Wolfram Mathworld.
What are isogonal conjugates?? @Nihar Mahajan
Log in to reply
In Δ A B C , cevians A P 1 a n d A P 2 are said to be isogonal conjugates of each other if and only if bisector of ∠ A also bisects angle between them.
Log in to reply
Log in to reply
@Harsh Shrivastava – Actually I am not much concentrating on NTSE bcoz its much based on school sylabi. I am concentrating on RMO , JEE , NSEA.
@Harsh Shrivastava – How's your preparation going on?
Log in to reply
@Nihar Mahajan – I am also not focussing on NTSE... :P
N o t e t h a t A F = F B , A E = E C , A O = R = S i n 5 4 A F , S i n 5 4 = 4 1 + 5 , S i n 4 5 = 2 1 , b y S i n L a w A E = 4 1 + 5 2 ∗ A F = 1 + 5 4 2 ∗ A F ∴ A E 2 = 3 + 5 4 ∗ A F 2 A O 2 = 3 + 5 8 ∗ A F 2 . ∴ O F O E = A O 2 − A F 2 A O 2 − A E 2 = ⎩ ⎪ ⎪ ⎨ ⎪ ⎪ ⎧ 3 + 5 8 − 1 3 + 5 8 − 3 + 5 4 ⎭ ⎪ ⎪ ⎬ ⎪ ⎪ ⎫ = { 8 − ( 3 + 5 ) 8 − 4 } = 5 − 5 2 = b − b a . ∴ ⌊ 2 + 5 1 5 0 0 ⌋ = 2 1 4
Hey @Nihar Mahajan
Which standard you are actually in,Are you 14 years old???
Yes I am 14 years old. I am studying in Std. 10. What about you?
Advice: Please refrain from posting comments as a solution. If you want to comment/ask something , you can click the "Reply button" at the bottom of solution section and type your comment/opinion in the box saying "Write a comment or ask a question...".Thanks.
Log in to reply
So awesome in geometry at the age of 14;You are a Genius!
We first construct segments O A , O B , and O C . Since O is the circumcenter, we know that these three segments have the same length, and triangles A O B , A O C , and B O C are isosceles. Thus, E and F are bisectors of sides A C and A B , respectively.
We then assign the measure of ∠ B A O as α , and from here we angle-chase a little bit until we reach ∠ A B O = 7 2 − α . Since ∠ A B O = ∠ B A O = α = 7 2 − α , α = 3 6 . Thus, ∠ F A O = α = 3 6 , and ∠ O A E = 8 1 − α = 4 5 .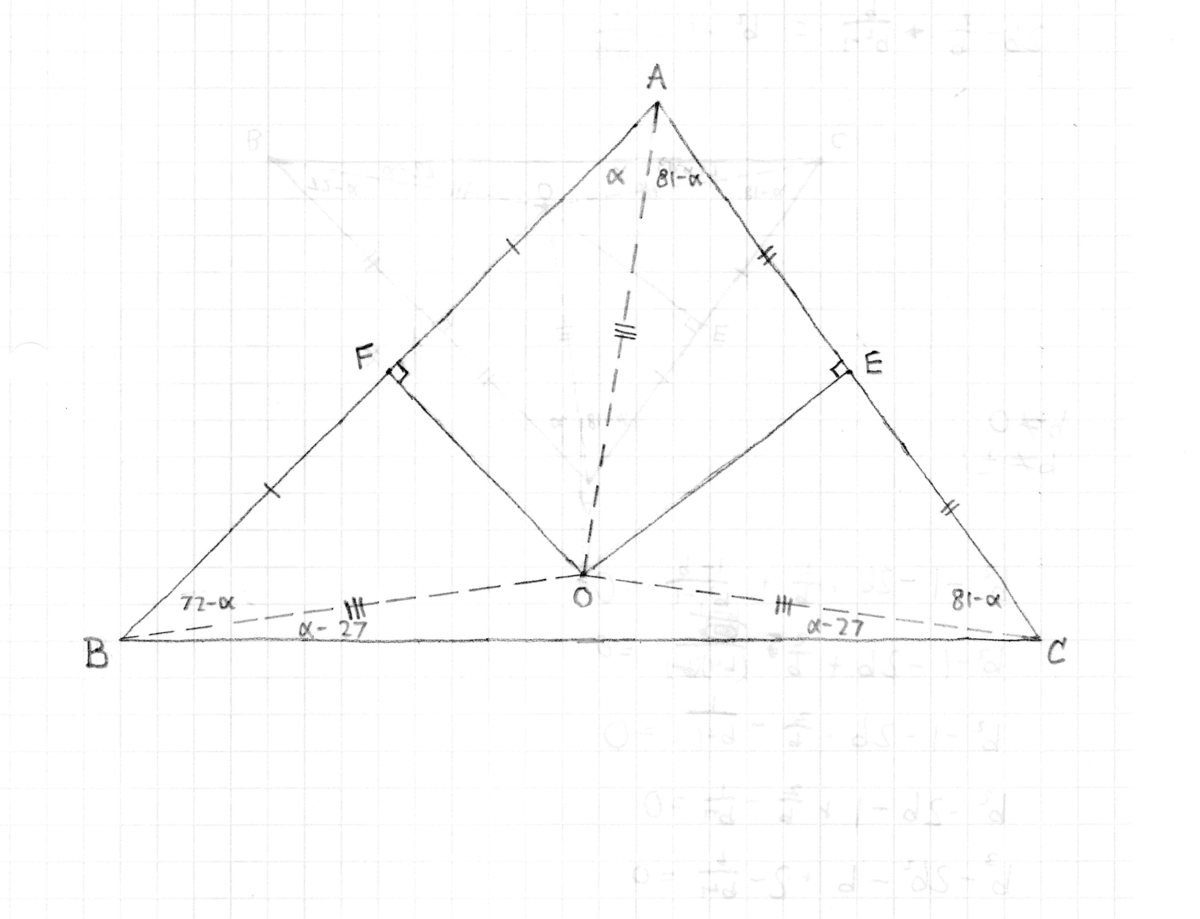 We then have
O
F
=
O
A
×
sin
3
6
, and
O
E
=
O
A
×
sin
4
5
, so
O
F
O
E
=
sin
3
6
sin
4
5
=
4
1
0
−
2
5
2
2
=
5
−
5
2
.
We then have
O
F
=
O
A
×
sin
3
6
, and
O
E
=
O
A
×
sin
4
5
, so
O
F
O
E
=
sin
3
6
sin
4
5
=
4
1
0
−
2
5
2
2
=
5
−
5
2
.
⌊ 2 + 5 1 5 0 0 ⌋ = 2 1 4