#20 of June 2017 Grade 10 CSAT (Korean SAT) mock test
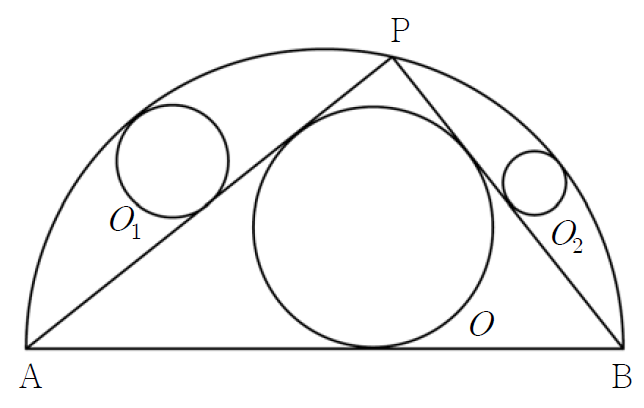
As shown above, there is a half circle whose diameter is segment and radius is .
Let be on the half circle.
A line segment that has midpoints of chord and arc as its ends is circle 's diameter. Similarly, a line segment that has midpoints of chord and arc as its ends is circle 's diameter.
Circle is an inscribed circle of .
The minimum of the sum of the areas of , , and is .
Find the value of .
This problem is a part of <Grade 10 CSAT Mock test> series .
The answer is 675.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
Like the picture above, let Q, R be midpoints of AP , BP respectively.
Also, AP , BP and AB contact with circle O at S, T, H respectively.
Midpoint of AB is C .
Radii of O , O 1 and O 2 are r , r 1 and r 2 respectively.
Let PQ = a and PR = b .
.
Since CQ = PR and CR = PQ ,
r 1 = 2 5 − b , r 2 = 2 5 − a .
.
Meanwhile, since AS = AH and BT = BH ,
( 2 a − r ) + ( 2 b − r ) = 5 × 2 ;
r = a + b − 5 .
.
The sum of the areas of O , O 1 and O 2 is equal to:
π { ( 2 5 − b ) 2 + ( 2 5 − a ) 2 + ( a + b − 5 ) 2 } ⋯ [ A ]
Let a + b = t ( 5 < t ≤ 5 2 ) , and the expression [ A ] is equal to:
π ( 4 a 2 + b 2 − 1 0 ( a + b ) + 5 0 + ( t − 5 ) 2 ) = π ( 4 7 5 − 1 0 t + t 2 − 1 0 t + 2 5 ) ( ∵ a 2 + b 2 = CP 2 = 2 5 ) = π ( t 2 − 2 2 5 t + 4 1 7 5 ) = π ( t − 4 2 5 ) 2 + 1 6 7 5 π
Therefore, S = 1 6 7 5 π .
π 1 4 4 S = 1 4 4 × 1 6 7 5 = 6 7 5 .