3 Logicians Walk Into A Juice Bar
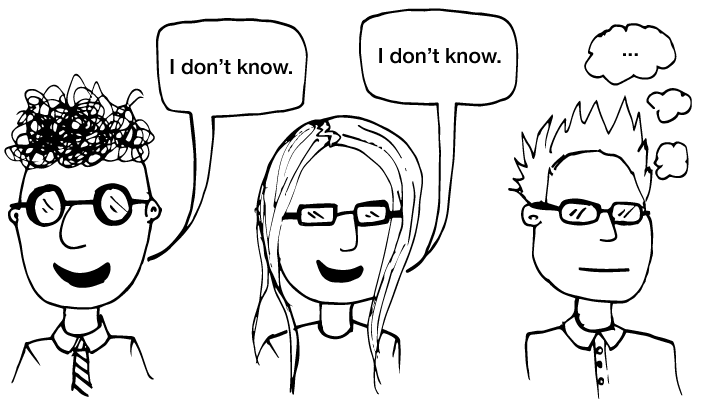
Three logicians enter a juice bar. Each either wants a drink or doesn't.
The barman asks, "Does everybody want a drink?"
The first logician says, "I don't know."
The second logician says, "I don't know."
What does the third logician say?
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
11 solutions
Neither the first or second logician reveals any information. The question is: Does EVERYBODY want a drink? The logician that answers first may or may not want a drink, this is irrelevant, he can't speak for everyone. He has no way of knowing whether 2 or 3 want drinks. This is an identical case to 2, as 1 saying "I don't know" doesn't reveal whether he wants a drink or not. Now we get to 3 He has no way of knowing what the others want either, so his answer should be "I don't know" as 1 and 2 could both want drinks, but they could also want to have no drinks. 3 has no way of knowing, as no information has been revealed.
The ways the answer you give could be correct are: 1 and 2 individually talked outside the bar to 3 about their choice, or the question should be asked differently: The bartender would have to ask 1 as an everyone ,then 1 and 2 collectively as an everyone, then finally 1, 2, and 3 as everyone.
The answer you give is technically correct. They either all want drinks or at least 1 doesn't, but that is the same as "I don't know" as the true answer could be either Yes or No, but neither could be definitive because no information has been revealed.
Log in to reply
I agree with James. You have to look at the barman's question the way a logician would: "Does EVERYBODY want a drink?". There is know way of any of the 3 of them knowing if all 3 of them want a drink. Unless they talked ahead of time, which is not part of the story so I assume they didn't.
They all wants to drink. Why? For what is the reason they entered a bar then?
Log in to reply
Yeah, unless that they are just going in with eachother
@Amirul Asman - by your logic, the answer should be Yes. Because that's why they entered the bar....
I believe the 3rd logician's answer should've been "yes" ... Otherwise he would first answer "No" to the barman question ;p.
That been said - I answered "Yes or No" ^^
I had the same reasoning just didn't think it through like you did.
WHY DID ALL 3 GO INTO A BAR AND WALK UP TO A BARTENDER!??? This, I don't like. We are to be thinking logically, no? For a funny example: Two of the logicians say they don't know because they are nerds. The 3rd is an alcoholic, so he says, "Uh... I brought you all to a bar. Bars are where drinks happen. We'll all 3 of us have a drink, barkeep." My point is that given ALL the information, why would 3 people walk into a bar and up to a bartender if none of them want a drink? Seems super illogical to me that that is a possibility. It seemed like that detail was there on purpose. Misleading.
Excuse me, but this is a BS. How do you know that "The first two logicians want a drink" and "Similarly, the second logician knows that he wants a drink" ? You cannot know this for sure.
Log in to reply
It's not BS. If the first 2 logicians did not want a drink, they would have answered "No" to the question "Does everybody want a drink". Since they answered "I don't know", it's only because they DO want a drink.
Log in to reply
Wait. Why are we complicating the issue here? The logician is clearly being sarcastic towards the barman question. Logically assuming, why would 3 logicians enter a juice bar if they don't want to drink? Why would you enter a bar if you are not drinking?!
Log in to reply
@Amirul Asman – Agreed. At least one of them must 'know' that they want a drink, as otherwise logic dictates they would not have entered the juice bar in the first place........
"Does everybody want a drink?" is a question that requires all three logicians to want a drink for the answer to be "Yes." If there exists one person who doesn't want a drink then the "everybody" part will be false.
Therefore, if the first or second logician don't want a drink, then they can simply say "no" in response to the question. For each logician, they want to drink, but they don't know what the rest will say so they can't decisively say, "Yes."
Thus, when it's the third logician's turn, he knows that his friends want to drink but he may or may not want a drink for himself. Hence his answer could be yes or no.
if the third guy is as smart as the 53% who got it wrong his answer could be i dont know
Let's name the logicians Alice, Bob and Charles respectively.
Consider Alice. If she does not want a drink, she would know that the answer to the barman's question - "Does EVERYBODY want a drink?" - is "no". But she doesn't know the answer to the question. Therefore, she does want a drink (which makes sense, because she walked into a bar).
Now, using the above logic, Bob knows that Alice wants a drink. If Bob does not want a drink, he would know that the answer to the barman's question is "no". But since he doesn't know the answer, he wants a drink too (again, sensibly).
Charles now knows that Bob and Alice both want drinks, but he may or may not want a drink. If he does want a drink, he would answer "yes" and if he does not want a drink, he would answer "no."
Hence, both "yes" and "no" are possible answers.
(PS: The first answer that crossed my mind was "Ouch!", since the logicians walked into a bar, and that must be rather painful)
(PPS: This reminds me of a classic joke: After hearing that a logician's wife had just given birth, a friend asks: "Is it a boy or a girl?" The logician's reply? "Yes.")
oh c'mon now, they wouldn't be in a bar if they (or at least one of them) didn't want a drink, they'd be in a coffee shop. I stand by YES
Log in to reply
Two of them are just saying "I don't know" not "I don't drink".
Log in to reply
yes also they does not mention either they came in the bar and says dont know ...the 1st logician and 2 nd maybe wanted and 3rd one yet not at guess of i dont want...
At least one of them should be wanting a drink for this scenario to make sense. Those who don't drink could simply be accompanying those who do. We know logicians 1 and 2 do want to drink. Logician 3 could simply be a close friend of theirs coming to chat while they drink alcohol. But they don't know in advance whether he wants to drink or not, otherwise logician 2 would have answered yes or no respectively!
Agreed! Why would any one want to enter a bar without wanting a drink! even though they may not pay for it! again it depends who was to pay for the drinks! also if the 3rd is an employee there may be he is on duty and does not drink!
two of them followed the third person to the bar. And the third person answer;"yes, please give us a glass of vodka"...
actually, the puzzle doesn't say that they are the only three people in the bar, so, even if the third guy wants a drink, "no" is a possible answer in that scenario as well.
Log in to reply
XD I made the assumption that everybody refers to the three logicians. For all we know, one of the logicians could be named "Everybody".
May I ask why is then the option that he could have said "I don't know" included in the answer? As far as I understand, he could perfectly reply "yes" as well as "no", but when "I don't know"?
Log in to reply
Hey, sorry, but the question was changed from the original version, which had the correct answer "both yes and no are possible answers".
In the new question, I'm not sure why "I don't know" should be accepted, unless the third logician has not made up his mind.
Then again, there are no constraints imposed on the logicians: they need not even tell the truth!
This is a bad question. The question seems to want us to assume that the only possible answers are "yes" or "no", rather than a genuine "I don't know" irrespective of the other answers. If there is a genuine "I don't know" answer, then the answer is clearly "no", since both guys 1 and 2 are "I don't knows" rather than either "yes" or "no". But if "yes" or "no" are the only possible states of being, then the answer is "yes" because either guy 1 or 2 would have to have answered "no" if they themselves did not. Ambiguous.
That's exactly my line of thought, so you get "Yes" and "No" as possible answers. But why is "I don't know" a possible answer? Assuming, of course, that there's no catch and we're abstracting the fact that any answer at all is a possible answer.
There is no connection in all of the three persons which make them independent variable according to first statement "Each either wants a drink or doesn't". But question asked by the bartender make them one subject. According to your explanation if "I don't know" is considered as NO then answer given can be justified. Now lets interpret the answer if he say YES is means EVERYBODY want drink if NO vise versa. Which counter the previous assumption considering "I don't know" as NO. But three person are independent they may or may not drink according to first statement. According to me "I don't know" should be the answer. By the simple logic of maths three variable one equation no definite solution.
Log in to reply
you're overcomplicating this. If they did NOT want a drink, they would answer NO, b/c they would know that everyone doesnt want a drink, since they don't want one. Since they either do or do not want a drink, it's dependent on the third guy, and the question clearly states that they either do or do not. They aren't saying "i don't know" because they aren't sure if they want a drink, they're only saying they don't know bc person 1 doesnt know what 1 and 2 want, and person 2 doesnt know what person 3 wants. Person 3 can say either yes or no, but not i don't know.
Log in to reply
so basically person 1 and 2 definitely want a drink.
uhh... ya... for ONE its impossible to tell if EVERYONE wants a drink. Maybe only 1 person hence the purpose of going into a bar. Unless each person clearly understands what each person's intentions were PRIOR to entering the bar (which is already ruled out due to the first two people saying "i don't know") then it is literally impossible for the last person to know what the first person wants. if the bartender's question was "Does SOMEONE want a drink?" then the answer would be correct in it capable of being "yes", "no", or "i don't know"... otherwise as the question stands, the answer HAS to be "I don't know" (only 1 wants a drink, but nobody knows if 2 or more)
I don't understand that classic joke of yours.
Convoluted scenario. Flawed contextual information. The solution given requires a specific pattern of logic that doesn't rule out other logical reasonings.
third would clearly say,'no, only me',
They walked into a bar. A juice bar.
If the third person says no, then the answer the bartender's questions is wrong. Since the first two want the drink, replying 'No' means that none of them want a drink which is not true
Log in to reply
Note the phrasing of the question: Does EVERYBODY want a drink? A "yes" implies that every single person wants a drink. If even one person does not want a drink, the answer to the above question is "no". So if the third guy says "no", the answer is correct since he doesn't want a drink.
The fact the first two both reply with, 'I don't know', means that both of them want to have a drink, since if either of them didn't then they would instantly know that not everyone wants a drink.
Therefore, if the third person wants a drink he will answer yes, and if he doesn't want a drink he will answer no. Hence we see that both yes and no are possible answers (though if the third man doesn't want a drink one must question why he would enter the bar in the first place ).
The barman asks if EVERYBODY wants a drink.First we need to keep in mind that EVERYBODY includes oneself. Now the first logician says I don't know. Two possibilities: yes or no. Analyzing these two probabilities... if he/she wants a drink, then that is 1/3 vote and we need to wait for the decisions of the other 2 logicians. If he/she doesn't want a drink, then the answer is immediately obvious and there is no point in awaiting the decisions of the other two logicians. So now we can deduce the decisions of the first two logicians (yes, they want drinks). Final decisions depends on the third logician's decision. Without him saying anything, we cannot be sure of what his decision is, and thus the answer is "Both 'Yes' and 'No' are possible answers". Hope that helped!
P.S. Sorry for all those gender assumptions. Irrelevant to the solution, so please don't judge. :)
Screw the first two guy and push them aside, and then order yourself a beer. Their indecision is not your problem
Similar to my thoughts which also included, "Why would anyone enter a juice bar if they didn't want a drink?". Perhaps I'm not cut out for logic. :)
A few things one must decide out of the gate: "they" in the bartenders question does not assume that any character possesses omniscient knowledge of the entire group's desire or lack of desire for a drink (each character only has knowledge of his/her desire). Second, "they" really is referring to the group and is not just a placeholder for a third person singular pro-noun, as is is often the confusing case in English where there is no third person singular gender-neutral pro-noun. So, the bartender's question refers to the groups desire for a drink though each character knows only his/her personal desire. When asked "Does everybody want a drink", the first two characters could very well say 'no' if either of them didn't want a drink since the necessary condition for "everybody wanting a drink" could not be met if just one character doesn't want a drink. However, since the first two characters answer "I don't know", we know that each of the first two character's personal choice must be "yes", which is implied by the contingency of those character's ultimate response upon the decision of the third character. This leaves the third character to decide if if the necessary condition of "everybody wanting a drink" will be met. If the third character desires a drink, his answer will be "yes", 'everybody wants a drink.' If the third character does not want a drink, the necessary condition will not have been met and he could well say "no." With knowledge of the first two character's decisions, we understand that the third character's decision will determine whether the necessary conditions will be met. Thus, the third logician may answer either "yes" or "no."
we don't know if the 3rd guy wants a drink, but the answer is either yes or no, so combining these 2, yes or no are possible answers.
The only suggestion is , just to click randomly on a option , and it turns out to be the correct answer
Diqjal "A sji!" ed lasjter diqjal zi vii eu envii pakaler zi a va eu enva. Zi lh'eneo diqjad "a ensji" mensa qujoen a va fjoen rinsos, parqjoen zi envoeqned fjoen rinsos, diqja enis "a sji: nausjter!", parqjoen eeni lhi chriesos vii fjoen rinsos enis. Zi lho deseo diqjad "a ensji" a parqjoen a va fjoen rinsos ausjter, lha sjime'ehreh, sjimea sjongeyry. Lho chrieseo eu sja ses'ehrehe, qjoen lh'enei desos vii fjoen rinsos, eu laelloeloep, capia fjoen diqjos "a sji": zi va fjoen rinsos diqjal "a sji: ciege!" eu, "a sji, nausjter!", zi a y enva. Etues?
Obviously the first 2 Logicians wants a drink .. Based on the question phrasing "Does everybody want a drink?"
If the 1st one didn’t want then the answer would simply be "No" as he will break the Unity of the group "everybody" that might want a drink Same for the 2nd .. meaning the first 2 are not answering only for themselves but for the group Now the 3rd knew what the first 2 wanted if he also wants then he'll say "Yes" and if not he'll say "No"
The first two logicians want a drink. We know this because if say, the first logician didn't want a drink, then his answer would be no. But because he does want a drink, and he's unsure if the others do, the first logician he says, "I don't know."
Similarly, the second logician knows that he wants a drink and that the first logician wants a drink, but again he doesn't know if the third logician wants a drink, so he says, "I don't know."
Finally, since we don't know if the 3rd logician wants a drink, his answer could be "Yes" or "No."