Too much of calculation?

Algebra Level 2

Find the value of $24798^2 - 24796^2$ .

Note: Please don't use a calculator.

The answer is 99188.

55 solutions

Nihar Mahajan
May 19, 2015

Let $24797 = a$ .Thus the expression becomes :

$(a+1)^2 - (a-1)^2 \\ =(a+1+a-1)(a+1-a+1) \\ =2a(2)\\ = 4a \\ =\boxed{99188}$

Moderator note:

Yes, this is just the difference of two perfect squares identity. Bonus question: Find the best approach to evaluate $24798^3 - 24796^3$ without using a calculator.

Creative thinking. Honestly, I didn't think of this!

$\huge Thumbs\quad up!!!$

@Nihar Mahajan , it would be great if we extend our relationship by being friends! What do you have to say?

Sravanth C. - 6 years ago

Lol we are already friends!!!

$\huge Cheers !$

Nihar Mahajan - 6 years ago

I meant closer friends. . . Anyways thanks for accepting my request!

Sravanth C. - 6 years ago

Hi, I just got confused on the second line. I know the difference of squares means it can be factored but why is the expression in the second parentheses (a + 1 - a + 1)? If a^2 - b^2 = (a+b) (a-b), and by substitution of the values, shouldn't it read (a+1 + a-1)(a+1-a-1)?

Drew Davis - 6 years ago

Its $a+1-(a-1) = a+1-a+1 = 2$ .You must put the brackets before you perform subtraction.

Nihar Mahajan - 6 years ago

Oh! Forgot about that. Thank you!!

Drew Davis - 6 years ago

bro cant we take square as whole then it would be (24798-24796)^2 then the square of 2 =4

Sàháj Siñgh - 6 years ago

$a^2 - b^2$ is not equals to $(a-b)^2$ .

Brilliant Mathematics Staff - 6 years ago

That's how I thought it should be solved.

Neil Antonio - 6 years ago

Why make 2 polynomials when you can just do one. Try quantity -2 squared and you save half your effort!

Riley Thornton - 6 years ago

Does symmetry of polynomials increases your efforts?

Nihar Mahajan - 6 years ago

In response to the Challenge Master note:

Using the Difference of Cubes formula:

$a^{3}$ - $b^{3}$ =(a-b)( $a^{2}$ +ab+ $b^{2}$ )

You can simplify the latter half of the above equation into

(a-b)( $a^{2}$ +ab+ $b^{2}$ )=(a-b)[ $(a+b)^{2}$ -ab]

Let a= 24798 and b =24796

$24798^{3}$ - $24796^{3}$ =(24798-24976)[( $24798+24796)^{2}$ -24798*24796]

=(2)[ $(49594^{2}$ -24798*24796]

=(2)*[2459564836-614891208]

=(2)*(1844673628)

=3689347256

As you can see, this is a very calculation-intensive way to solve but it is one of the faster ways to solve it in my opinion, assuming you don't mess up the large multiplications. It is, however, a better way than cubing both 24798 and 24796 and taking the absolute value of their difference.

Timothy Vu - 6 years ago

There's an easier approach.

Note that $a = b + 2$ . I would have tried letting $c = 24797$ , then the expression becomes $(a+1)^3 - (a-1)^3$ . Expand with binomial expansion. Can you finish it off from here?

Brilliant Mathematics Staff - 6 years ago

Yes , indeed this is the simplest approach.

$(a+1)^3-(a-1)^3 \\= [a+1-(a-1)][{(a+1)-(a-1)}^2+3{(a+1)(a-1)}]\\ = 2[4+3a^2-3] = 2[1+3a^2] = 2[1+3(24747)^2] \\= 2[1837242027+1]=3674484056$

Nihar Mahajan - 6 years ago

@Nihar Mahajan – You can almost immediately jump to $2(1+3a^2)$ . Hint: Binomial expansion, $(A\pm B)^2 = \ldots$ , instead of applying the difference of perfect cubes identity.

Now, what is the best way to calculate $24747^2$ (and still without using calculator)? Hints: $24747 = 25000 - 250 - 3$ and expand $(a-b-c)^2$ .

We should not always be dependent on calculators all the time.

Brilliant Mathematics Staff - 6 years ago

The expression is wrong in the first place. If you intend to square the numbers, the right expression should be (24798)^2 - (24796)^2 not 24798^2 -24796^2. You are confusing the readers. Let us say a = 2, b = 4, c = 7, d = 9, can you say abcdc+1^2 the same as abcd(c+1)^2 the same? or abcdc-1^2 the same as abcd(c-1)^2?

Enrique Bargan - 5 years, 2 months ago

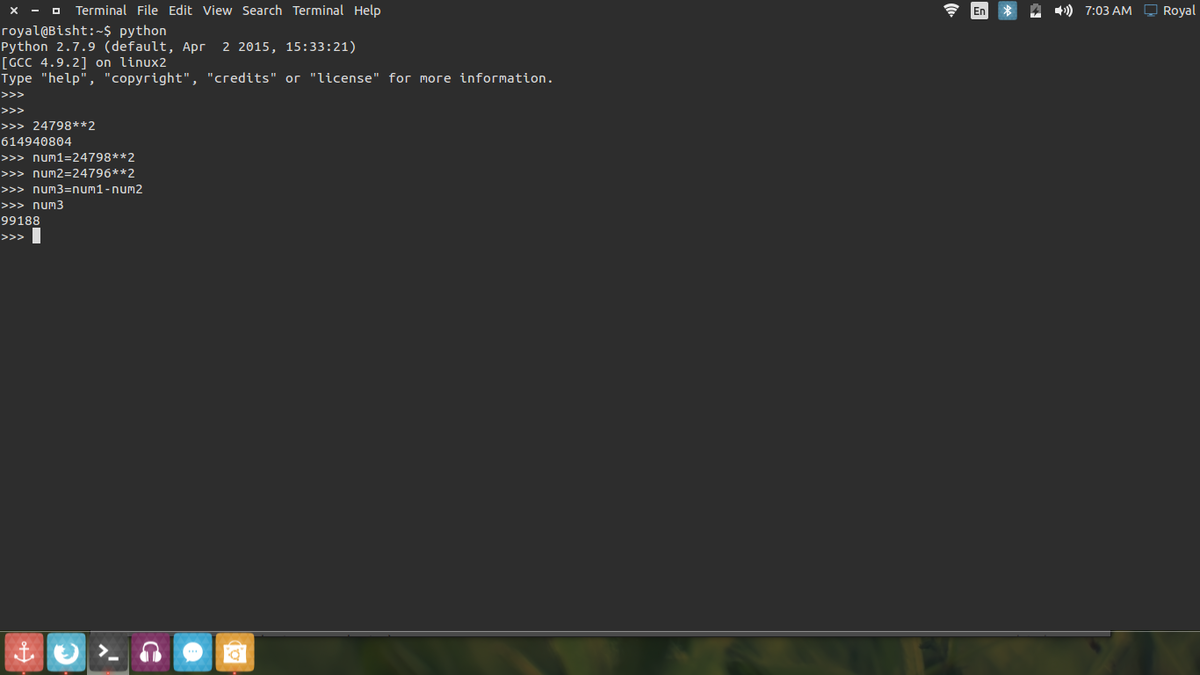
i HAVE A DIFFERENT APPROACH: let x=24798. Then the problem reads x^2-(x-2)^2. This transalates into 2x+ 4. Hence 2*24798+4 that transforms into 49600.

Rishiyur Balakrishnan - 6 years ago

x^2 - (x-2)^2 = x^2 - (x^2 - 4x + 4) = 4x - 4. and 4 (24,798) - 4 = 99,188

Barbara Maidelis - 6 years ago

it translates to 4x+4 not 2x+4

Callum Goodall - 6 years ago

Sravanth C.
May 19, 2015

We can also use this formula but it's a bit lengthier than Nihar Mahajan's,

Let, $a=24798$ and $b=24796$

Use, $a^{2}-b^{2}=(a+b)(a-b)$

Substituting the values we get,

$24798^{2}-24796^{2}=(24798+24796)×(24798-24796) \\ =(49594)×(2) \\ =\boxed{99188}$

Palash Som
May 19, 2015

this problem could be solved easily by using the formula that i created i.e.

$(a+2)^2$ - $(a)^2$ = $\boxed{4\times(a+1)}$

Be careful with notation. Before starting, you should have defined 'a' . In that case, a = 24796. But if had used this formula: [ a^2 - (a-2)^2) = 4 x (a-1) ], then the value of 'a' had been a=24796. This can look like something trivial but in mathematics you can never give a solution with a non-defined expression.

Likewise, congratulations for your result. That's right. Go on practising !!

Diego G.M - 6 years ago

Mine was this approach. :)

Arjun Madhavankutty - 6 years ago

Renan Birck Pinheiro
May 23, 2015

Be x = 24798.

Then one can write

$x^2 - (x-2)^2$

$= x^2 - (x^2 - 4x + 4)$

$= x^2 - x^2 + 4x - 4$

$= 4x - 4$

$= 99188.$

Pratik Raj
May 25, 2015

The given problem is to find the difference of two alternate squared number.

We can see that :

2^2 - 0^2 = 4

3^2 - 1^2 = 8

4^2 - 2^2 = 12 and so on.

We can see this as an Arithematic Progression with first term a = 4 and common difference d = 4.

24798^2 - 24796^2 is the 24797th term of this AP.

n = 24797

a n = a o + (n-1)d

Solution is : 4 + (24797-1)4 = 99188

Madiha Javid
May 24, 2015

n^2-(n-2)^2=4(n-2)+4Write a solution.

That +4 could factor in to make it 4(n-1)

Ben Donnangelo - 6 years ago

Khajahussain Shaik
May 23, 2015

a^2 - b^2 = (a+b)(a-b)= 49594×2= 99188

Mahesh Kp
Apr 4, 2016

a^2-b^2=(a+b)(a-b)

Suneesh Nair
Mar 19, 2016

You can also solve using (A square) minus (B Square) formula: (A-B) (A+B) 24798+24796=49594 (A+B) 24798-24796=2 (A-B) 49594 2=99188

Akash Patalwanshi
Nov 12, 2015

One can also use a = 24796 and b = 2 then

(a+ b) $^{2}$ - a $^2$

= 2ab + b $^{2}$

=( 2a)(2) + 4

= 4a + 4 = 99184 +4 = 99188

Muthukumaran Perumalsamy
Jul 17, 2015

Write a solution. (24796+2)^2 -(24796)^2 = 4 + 2x24796x2 = 4 (1+24797) = 99188

David Raju
Jul 16, 2015

it can be solved using the formula square distrubutive

Saharsh Anand
Jun 28, 2015

The same class 8th property... $a^2-b^2=(a+b)(a-b)$

So, $24798^2-24796^2=(24798+24796)(24798-24796)=49594\times 2=99188$

Prince Harshwardhan
Jun 18, 2015

we will use the property of (a+b)(a-b)

Willy Manzanas
Jun 5, 2015

since it's equal that (24796+2)^2-24796^2,then let x=24796 =(x+2)^2-x^2 =x^2+4x+4-x^2 =4x+4 =4(24796)+4 =99,188

Jagan Mohan Rao
Jun 2, 2015

Apply formula (a+1)^2 --(a-1)^2= 4a, where a=24797

Evan Dagg
Jun 1, 2015

It's a pattern, at first I too was stumped, but it clicked after a minute. I'm just glad to have learned something new today, I never knew such a pattern existed. The answer is the sum total multiplied by the difference. Like so:

3 squared = 3 * 3 = 9, and 2 squared = 4.

The results, 9 - 4 = 5

While 3 + 2 = 5

4 squared is 16, and 3 squared is 9.

16 - 9 = 7

4 + 3 = 7

4 - 3 = 1

1 * 7 = 7

The difference between the two is the same as the sum in those cases. Continuing:

6 squared is 36, 4 squared is 16.

36 - 16 = 20

6 + 4 = 10

6 - 4 = 2

2 * 10 = 20

So the answer of what squared subtract whatever else squared, is the same as the numbers added and multiplied by the difference between them. So if the numbers are 2 apart, then multiply by 2, if 3 apart, multiply by 3, etc.

So 24798^2 -24796^2 is the same as:

(24798 + 24796) * (24798 - 24796) =

(49594) * (2) =

99188

Pardon the informality, it's just a much easier explanation than those that require a stronger background in mathematics. The underlying logic is more important if someone is looking for the answer.

Ngo Duc Binh
May 31, 2015

24798^2 - 24796^2 = (24798 - 24796)(24798 + 24796) = 2 x 49594 = 99188

Mauricio Anaya
May 31, 2015

Not sure if the best or shortest but it is different from other responses .

Callum Goodall
May 31, 2015

The difference between 24796 and 24798 is two. If n becomes the value 24796 then you can therefore write the expression: (n+2)² - n², which equates to: n² + 4n + 4 - n², which can simplify to: 4n + 4. Replacing n with 24796 will give the number 99188

Rk Ravi Sundar
May 31, 2015

Using formula: a^2-b^2=(a+b)(a-b)

Jeffry Hartanto
May 29, 2015

Mine is pretty weird since I try to solve it using number pattern.

$4^{2} - 2^{2}$ = 12 = ( $4 \times 2$ ) + ( $2 \times 2$ )

$12^{2} - 10^{2}$ = 44 = ( $12 \times 2$ ) + ( $10 \times 2$ )

SO,

$24798^{2} - 24796^{2}$ = 99188= ( $24798 \times 2$ ) + ( $24796 \times 2$ )

I haven't try to figure it out, but I believe it's only true if it differs by 2..

Geoffrey De Velez
May 27, 2015

24798^2 - 24796^2

let x = 24796

= (x+2)^2 - x^2

= x^2 + 4x + 4 - x^2

= 4x + 4

substituting back x with 24796

= (4 * 24796) + 4

= 99184 + 4

= 99188

Dev Sharma
May 27, 2015

a^2-b^2 i.e (a-b)×(a+b) so 2×49595 = 99188

Partha Adhikari
May 26, 2015

Simple solution, for example take (3)^2-(1)^2 = 8 (4)^2-(2)^2= 12 (5)^2-(3)^2= 16 therefore we get, (n)^2 - (n-2)^2 = 4(n-1) So, (24798)^2 - (24796)^2 = 4(24797) = 99188

Stefanus Ricky
May 26, 2015

(a+1) x (a+1) - a x a = 2a+1 = a + (a+1)

just do it twice an you got (24796+24797 ) + (24797+24798)

Vedant Patil
May 26, 2015

its easy! (24798-24796) (24798+ 24798-2) =2(24798 2 -2) = 24798x4 - 4 =99188

Emrul Kais
May 26, 2015

let smaller number be n . So , given expression is, (n+2)^2 - n^2 = (n^2 + 4 n + 4 -n^2) = (4 n + 4) = 4*(n+1)

AwaIs Ali
May 26, 2015

Abhishek Nag
May 26, 2015

Even for multiplication of a large number, i.e. 24797 we might use a calculator, bt for addition we can avoid calculator. we can also use: a^2 - (a-1)^2 = a+a-1 so, 24798^2 - 24796^2 = 24798^2 - 24797^2 + 24797^2 - 24796^2 = 22798+ 2*24797+24796 = 99188

Hadiya Ali Khan
May 26, 2015

(24798)^2 - 24796 can be written as (24796+2)^2 - 24796 that equals =4+4(24796) =4+99184 =99188

Heugenel Delacruz
May 25, 2015

let h=24798
h^2 - (h-2)^2 =h^2 - (h^2 -4h +4) =4h-4

substitute the value of h =4(24798) -4 =99192 -4 =99188

Deepak Bisht
May 25, 2015

Lets feel something interesting :D

Atika Samiha
May 25, 2015

if we observe this 4^2-2^2=12,6^2-4^2=20,8^2-6^2=28,then we can establish an equation.let's assume that,b=a-2.so,a^2-b^2=(a+b)x2. here,24798^2-24796^2=(24798+24796)x2=99188

Mubashar Karamat
May 25, 2015

(a + b) * (a - b) 49594 * 2 = 99188

Saanika Gupta
May 24, 2015

Simply find a pattern, start out with smaller numbers such as 4^2- 3^2=7; 4+3=7 Try another 5^2-4^2=9; 5+4=9 See a pattern, the bases added together equal the answer. Now separate the bases by 2, 7^2-5^2= 24, which is the bases added together times 2, try with another, it works. So apply that pattern to this problem, 24798^2-24796^2= (24798+24796)*2 which equals 99188!