A calculus problem by Alex Li

It is known that there are some 3D closed shapes with infinite surface area, but only finite volume.

Is there a (continuously differentiable) curve $f: \mathbb{R} \rightarrow \mathbb{R}$ whose solid of revolution has a finite surface area but infinite volume?

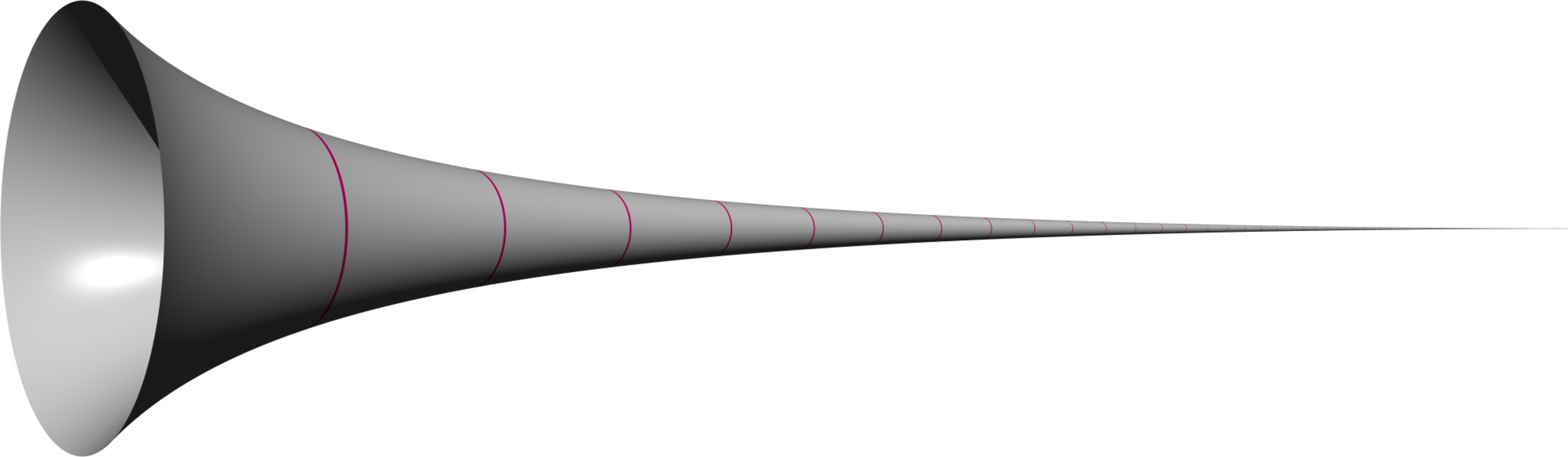
Gabriel's horn is formed by taking the solid of revolution of the curve $f: \mathbb{R^+} \rightarrow \mathbb{R}$ , $f(x) = \frac{1}{x+1}$ . It has finite volume and infinite surface area since:

$\begin{array} { l l } V & = \int_0^{\infty} \pi \left(\frac{1}{x+1}\right)^2 \, dx= \left[ \pi \frac{1}{x+1} \right]_0^{\infty} \\ & = \pi, \\ A & = \int_0^{\infty} 2\pi x \sqrt{ 1 + \left( \frac{-1}{(x+1)^2} \right)^2 } dx \\ & > 2\pi \int_0^\infty \frac{1}{x}\, dx = [ 2 \pi \ln (x+1)]_0^\infty = \infty.\end{array}$