This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
28 solutions
Ok this I understand thanks
i thought....(VI! x VII!):X!=V!(IxII):X=(VxII):X=1
Thanks a lot. What these problems are called?
Thank you, Leonardo. This is a very elegant and simple explanation.
Lovely explanation
its the easiest method ever bro
I cant understand
Log in to reply
You can think of it like this, 10! can be written as 7! X 8 X 9 X 10, as a result you can cancel the 7! from the top and bottom leaving yourself with 6! / 8 X 9 X 10
6! = 720, now cancel the 10 to get 72/8 X 9
8 X 9 is 72 so you are left with 72/72 which equals 1
So is mine
Log in to reply
Zeeshan i know u !! I have talked to u in case of requirement of fiitjee compendium u remember ??
If i solve it in this way - 6! 7!/ 10! = 6! ( 1 * 7 ) /10! = 7/ 10 9 8 7 = 1/ 720
I noticed that
6 ! = 7 2 0 = 8 × 9 × 1 0 = 7 ! 1 0 !
⇒ 1 0 ! 6 ! 7 ! = 1
nice work... thumbs up!
This is a classic case of Catalan Number: knowing the first few terms of the sequence, we get 6 × 7 = 4 2 = C 5 = 5 + 1 1 ⋅ ( 5 1 0 ) = 6 1 ⋅ 5 ! × 5 ! 1 0 = 6 ! × 5 ! 1 0 ! ⇒ 1 = 6 ! × 7 ! 1 0 ! . Reciprocate the expression yields the desired answer of 1 .
+1 for showing the scientific usefulness of 4 2 .
Thanks! I didn't know that!
Log in to reply
You can find out C 6 and it still works out! ⌣ ¨
Log in to reply
Yeah! it works .. amazing! Please suggest a name for the problem.
Log in to reply
@Nihar Mahajan – hehehe "No calculation above fifty"
Log in to reply
@Pi Han Goh – Sure ... troll master .. ⌣ ¨
Log in to reply
@Nihar Mahajan – Actually , I didn't understand the name of this question , can you explain it to me , Genius ?
Log in to reply
@A Former Brilliant Member – I'm no genius. All the other workings needs to show that 6 ! = 8 × 9 × 1 0 = 7 2 0 or 8 × 9 = 7 2 which are well above 5 0 , so setting an arbitrary rule like saying you can't do any arithmetic on a number that gives a resultant number greater than 5 0 makes it more challenging.
Log in to reply
@Pi Han Goh – Ooh! Thanks , I can't believe I never saw that .
Besides , do you plan on creating a message board ? We all can learn from genii like you :)
Log in to reply
@A Former Brilliant Member – I doubt that. We can chat on other platforms. Got an email or something?
@Pi Han Goh – Yeah , foolish on my part .
@A Former Brilliant Member – See Pi Han Goh's solution , it does not need calculations above 50 (actually 42) which is a possible solution , while people will calculate 720 like me..
Log in to reply
@Nihar Mahajan – What ths hell does the ! Mean? I never even seen that.
Can you please explain how you solved this question using combination?!!!
Log in to reply
Do you know the basics of combinations: ( m n ) ?
Log in to reply
Yes I do know about combination
Log in to reply
@Harshit Sharma – After knowing some basic properties of combinations, the next thing you do is read up Catalan's number. Then, voilà! You got the answer.
Noman...how can we post picture there ? This is the easiest n handy way to explain. I tried to post a pic but don't know the medium.
would you please guide me Why 6! = 720?
6!7!/10!
=6!/(10.9.8) [divide top and bottom by 7!]
=6.5.4.3.2.1/10.9.8 [write 6! out]
=3.2.5.2.2.3.2/5.2.3.3.2.2.2 [write as product of primes]
=1
6 x 5 x 4 x 3 x 2 x 1 x 7 x 6 x 5 x 4 x 3 x 2 x 1 / 10 x 9 x 8 x 7 x 6 x 5 x 4 x 3 x 2 x 1 then cancel it all out
6! x 7!/10!
=6! x 7! /10 x 9 x 8 x7!
=6!/10 x 9 x 8
=720/720=1
6! x 7!/10!=6! x 7!/10 x 9 x 8 x7!
We omit 7! It is reduced to 6x5x4x3x2/3x3x2x4x2x5=1
6! X 7! / 10 x 9 x 8 x 7! factorial seven cancelled each other then 720 / 720 = 1
6 ! × 7 ! = 2 2 × 3 2 × ( 2 2 ) 2 × 5 2 × ( 2 × 3 ) 2 × 7 = 2 8 × 3 4 × 5 2 × 7
1 0 ! = 2 × 3 × 2 2 × 5 × ( 2 × 3 ) × 7 × 2 3 × 3 2 × ( 2 × 5 ) = 2 8 × 3 4 × 5 2 × 7
6 ! × 7 ! = 1 0 ! ⇒ 1 0 ! 6 ! × 7 ! = 1
(6! 7!)/10! =(1 2 3 4 5 6 1 2 3 4 5 6 7)/1 2 3 4 5 6 7 8 9 10 =(1 2 3 4 5 6)/(8 9*10) =720/720 =1
It's italicized because you put the asterisks, and something between 2 asterisks is italicized . You need L A T E X .
simple... 1 0 ! 6 ! ∗ 7 ! = 8 ∗ 9 ∗ 1 0 1 ∗ 2 ∗ 3 ∗ 4 ∗ 5 ∗ 6 =1 therefore, ans.= 1
How do you know 6!/(8x9x10)=1? Then, you can move on to “therefore,”.
6! X 7! /10! = 6!/(8 * 9 * 10) = 1 as both are 720 or by canceling like terms in 5 * 2, 3 * 2, 2 * 2 and 3
Moderator note:
How do we know that 6! / 8 * 9 * 10 = 1?
You prime-factor it.
6!= 6 x 5 x 4 x 3 x 2 x 1
7!= 7 x 6 x 5 x 4 x 3 x 2 x 1
10!= 10 x 9 x 8 x 7 x 6 x 5 x 4 x 3 x 2 x 1 = 10 x 9 x 8 x 7!
6! x 7!/ 10! = 6! x 7!/10 x 9 x 8 x 7! = 6!/ 10 x 9 x 8 = 6 x 5 x 4 x 3 x 2 x 1/ 10 x 9 x 8 = 6 x 5 x 3 x (4 x 2 x 1)/10 x 9 x 8 =6 x 5 x 3 x 8/10 x 9 x 8 = 6 x 5 x 3/ 10 x 9 =90/90= 1
I included every step just in case anyone is confused! Hope it helps 😊
6! 7! / 10! = 6 5 4 3 2 1 7!/10 9 8 7! =2 3 5 4 3 2 1/2 5 3 3 4 2 =2 3 5 4 3 2 1/2 3 5 4 3 2 =1
See what I told @Rakibul Hasan Emon on this question
1x2x3x4x5x6x1x2x3x4x5x6x7/1x2x3x4x5x6x7x8x9x10 =2x3x4x5x6/8x9x10=1
7!×6!\10!
6!/10×9×8
720/720
1
1 0 ! 6 ! ∗ 7 ! = 1 0 ∗ 9 ∗ 8 ∗ 7 ! 6 ! ∗ 7 ! = 1 0 ∗ 9 ∗ 8 6 ∗ 5 ∗ 4 ∗ 3 ∗ 2 6 ! : 3 0 , 1 2 0 , 3 6 0 , 7 2 0 1 0 ∗ 9 ∗ 8 : 9 0 , 7 2 0 7 2 0 7 2 0 = 1
simple fac logic 6! 7!/10! 6!/10 9*8 6!=720 so answer 1
6! x 7! = 3628800 10! = 3628800 3628800/3628800=1
Really easy!
What if it really big number like 12! Or 24! You can't do that way
6! 7!/10!=6! 7!/10 9 8*7!=720/720=1
(6!X7!)/10! =(720X5040)/3628800 =3628800/3628800 =1
(6!×7!)/10! (6×5×4×3×2×1×7!)/(10×9×8×7!)=(6×5×4×3×2×1)/(10×9×8)=1
7!6!/10! 7!6!/10 9 8 7! 6!/10 9 8 120 6/720 720/720 1
In easier form, 6 X 5 X 4 X 3 X 2 X 1 X 7 X 6 X 5 X 4 X 3 X 2 X 1
10 X 9 X 8 X 7 X 6 X 5 X 4 X 3 X 2 X 1
= 6 X 5 X 4 X 3 X 2 X 1 _ _ _ _ _ __ 10 X 9 X 8 = 1
Just expand this and cancel all the factors and rada u get the answer
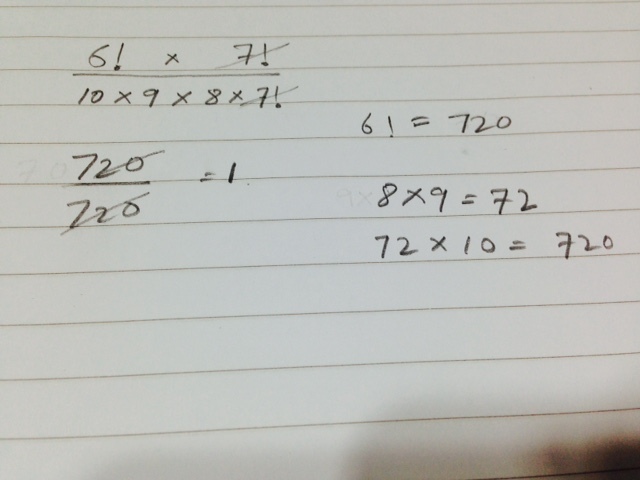
Since 1 0 ! = 1 0 × 9 × 8 × ( 7 ! ) ,
1 0 ! 6 ! × 7 ! = 1 0 × 9 × 8 × 7 ! 6 ! × 7 ! = 1 0 × 9 × 8 6 × 5 × 4 × 3 × 2 × 1 . Then, we can expand the denominator: 1 0 5 × 2 × 9 3 × 3 × 8 4 × 2 6 × 5 × 4 × 3 × 2 × 1 , and canceling common terms gives: 3 × 2 6 × 1 = 6 6 = 1 .