What a Magnitude!

We know that:

$\left| \vec { a } +\vec { b } +\vec { c } \right| \le \left| \vec { a } \right| +\left| \vec { b } \right| +\left| \vec { c } \right| \\ \vec { a } =(a,1);\vec { b } =(b,4);\vec { c } =(c,7)\\ \Rightarrow \vec { a } +\vec { b } +\vec { c } =(5,12)\Rightarrow \left| \vec { a } +\vec { b } +\vec { c } \right| =13\quad (\because a+b+c=5)\\ \Rightarrow \left| \vec { a } \right| +\left| \vec { b } \right| +\left| \vec { c } \right| \ge 13$

You know, when you have $5$ and $12$ , The answer is definitely $\boxed{13}$

This is such a beautiful question... I'll outline a physics/elementary geometry based approach.

The given quantities are obviously distances from A(a,1) , B(b,4) and C(c,7).

Let's consider a simpler case by fixing $c$ (for now).

That is, let's find the maximum value for $PA+PB$ subject to the constraint $a+b=k$ for some k.

Look at the figure below. (Note that the figure marked as A is actually the reflection of A about the y-axis, but that doesn't matter as far as distance is concerned)

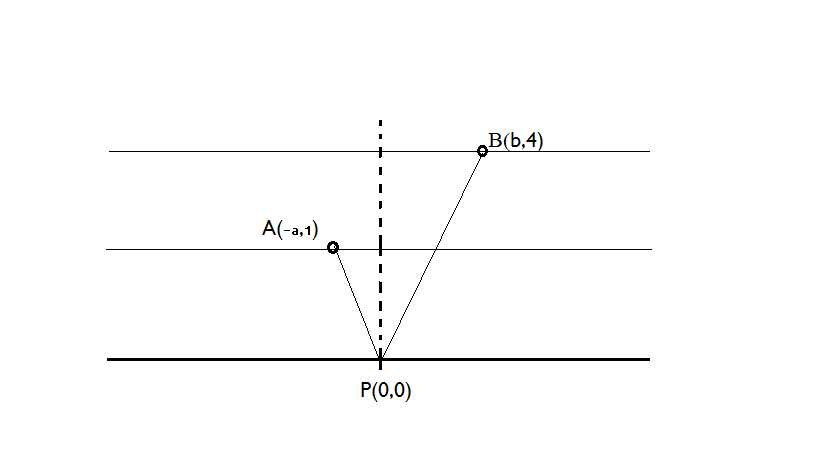
First note that point $A$ is constrained to move along $y=1$ and similarly $B$ has to move along $y=4$ . Also, their horizontal separation is fixed(=k).

Forget point P for now and instead consider the question, what is the minimum value of $DA+DB$ for any point $D$ on $y=0$ ? Well, that's pretty easy...

Reflect $B$ (or A) about $y=0$ and join this new point to A. The point where this line meets y=0 is the required point. This is equivalent to using Fermat's principle of least time. Now, this point is such that the angle of 'incidence' equals the angle of 'refraction'.

Okay, so now if we move the point $A$ (and thus B also has to move the same distance) such that point P coincides with $D$ , then this configuration of $A \quad and \quad B$ is what we need.

From geometry, we will get, $\frac{a}{b}=\frac{1}{4}$ .

But we did this after fixing the point $C$ . We could have fixed point A or B. Therefore, $if$ , the conditions we get by fixing the three points separately yield a common solution, then we are done.

(P.S : This is also what happens if we use Langrange multipliers. i.e We get three equations which we solve to get a concurrent solution).

Fortunately the solution does exist. Based on our previous reasoning it is easy to see that for the given values, $a:b:c=1:4:7$ and $a+b+c=5$

This yields, a= $\frac{5}{12}$ , b= $\frac{5}{3}$ , c= $\frac{35}{12}$ .

Note that P, A, B, C lie along a straight line in this case.

Without using Lagrange multipliers

let a= $\tan {\theta}$ b= $4\tan {\phi}$ c= $7\tan {\delta}$

Then we have

$S = \sec {\theta} + 4\sec{\phi} + 7\sec{\delta}$

where S is what we need to minimise given that $\tan {\theta} + 4\tan {\phi} + 7\tan {\delta} =5$

Now let us square S, to get

${ sec }^{ 2 }\theta \quad +\quad 16\quad { sec }^{ 2 }\phi \quad +\quad 49\quad { sec }^{ 2 }\delta \quad \\ \\ +8sec\theta sec\phi +56sec\phi sec\delta \quad +\quad 14sec\delta \quad sec\theta \\$

$\displaystyle Now$

$sec\theta sec\phi =\sqrt { 1+{ tan }^{ 2 }\theta } \sqrt { 1+{ tan }^{ 2 }\phi } \quad \ge \quad =1+tan\theta tan\phi$

similarly doing for other two and then using

$\\ 1+{ tan }^{ 2 }\theta ={ sec }^{ 2 }\theta$

i get

$\\ { sec }^{ 2 }\theta \quad +\quad 16\quad { sec }^{ 2 }\phi \quad +\quad 49\quad { sec }^{ 2 }\delta \quad \\ \\ +8sec\theta sec\phi +56sec\phi sec\delta \quad +\quad 14sec\delta \quad sec\theta \\ \ge \quad 1+16+49+8+56+14+{ tan }^{ 2 }\theta \quad +\quad 16\quad { tan }^{ 2 }\phi \quad +\quad 49\quad { tan }^{ 2 }\delta \quad \\ \\ +8tan\theta tan\phi +56tan\phi tan\delta \quad +\quad 14tan\delta \quad tan\theta \quad =\quad 144+(tan\theta +4tan\phi +7tan\delta )^{ 2 }\quad =\quad 169$

so S= $\sqrt {169}$ = 13

DIRECT APPROACH USING MINKOWSKI'S INEQUALITY

$\sqrt{a^2+1^2} + \sqrt{b^2+4^2} + \sqrt{c^2+7^2} \geq \sqrt{(a+b)^2+(1+4)^2}+ \sqrt{c^2+7^2} \geq \sqrt{(a+b+c)^2+(1+4+7)^2} = \sqrt{5^2+12^2} = \boxed {13}$

By lagrange's multipliers,

1. a+b+c=5
2. $\lambda$ = $\frac{a}{\sqrt{a^{2}+1}}$
3. $\lambda$ = $\frac{b}{\sqrt{b^{2}+1}}$
4. $\lambda$ = $\frac{c}{\sqrt{c^{2}+49}}$

By using 2,3 and 4, b=4a and c=7a. Substituting b and c in 1, a= $\frac{5}{12}$ , b= $\frac{5}{3}$ , c= $\frac{35}{12}$

By using these values one can easily calculate given expression to be $\boxed{13}$

if a,b,c are real not taking a+b+c=5,then min value when a=b=c=0,then its value is 1+4+7=12,but when c=5,then sqrt of (c^2+49)<9,then min value is<14,so it is 13.

what is lagrange multiplier???

i tried by cauchy schartz but i am getting min as 15..Y is that..but i ended up guessing 14 and 13 to get right ANS

Well, the solutions given are quite "over the head"(that's because they are really quite amazing). But an alternative always goes fine(I guess).

By AM-GM,

$\sqrt{{a}^{2} + 1} \geq \sqrt{2a}$

$\sqrt{{b}^{2} + 16} \geq \sqrt{8b}$

$\sqrt{{c}^{2} + 49} \geq \sqrt{14c}$

Adding them all up,

$\sqrt{{a}^{2} + 1} + \sqrt{{b}^{2} + 16} + \sqrt{{c}^{2} + 49} \geq \sqrt{2a} + \sqrt{8b} + \sqrt{14c}$

Just arranging it a little bit,

$\geq \sqrt{14c} + \sqrt{8b} + \sqrt{2a}$

Using Chebyshev,

$\geq \frac{\sqrt{14} + \sqrt{8} + \sqrt{2}(\sqrt{a} + \sqrt{b} + \sqrt{c})}{3}$

Here I assume that $a,b,c$ are positive reals because that will not change the equation. Hence, $a,b,c <5$ , hence, their sum of square roots will be just less than their sum. Therefore,

$\geq \frac{\sqrt{14} + \sqrt{8} + \sqrt{2}(5)}{3} \approx 13.307163456488711$

Hence, our final answer becomes $13$ .

I plugged in c = 5. I got 1 + 4 + √73 < 14. So it has to be an integer greater than 12 and less than 14, I wonder what it could be?