A chemistry problem by Siddharth Parkar
Find the total number of cyclic structures and stereomers isomers possible for a compound of formula C X 5 H X 1 0 .
The answer is 7.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
2 solutions
Hey the answer is 7 but the solution above is wrong. 1,2-dimethyl-cyclopropane has 2 chiral carbon atoms, but since it has same substituents bonded to it there's a meso form, therefore giving rise to 3 stereoisomers. So totally 7 with only cyclic considered.
Log in to reply
Make a report to confirm this. Why don't you post a solution.
Log in to reply
Its not about writing another solution or reporting. I was saying only a part of your solution is wrong because of what I said above. It should be 4 + 3 = 7
Log in to reply
@Vignesh S – My answer is incorrect. I just want the correct answer from the rest or the challenge master.
@Vignesh S – I am getting 5 cyclic structures. Where am i wrong?
cis-2-pentene and trans-2-pentene are stereomers, but what about the cyclic structures? Don't they have any stereomers? Are they all achiral? @Chew-Seong Cheong
Log in to reply
Make a report and post a solution.
Going through the 5 cyclic structures, they can't have any isomer.
Log in to reply
Why not Sir?
Log in to reply
@Anik Mandal – For example cyclopentane, it has only one form. Methylcyclobutane, however you place the branch (---H), you still get the same by rotating or flipping. Same for the other cyclic structures.
@Anik Mandal – I am also learning Chemistry. I checked what I learn and may be this a better reply. From this link , we note that:
The essential requirement for this stereoisomerism is that each carbon of the double bond must have two different substituent groups (one may be hydrogen).
This means that for stereoisomerism to happens, we must first have a double bond and then the each carbon of the double bond must have two different substituent groups. Two different substituent groups is impossible for double bond in a cyclic structure.
Log in to reply
@Chew-Seong Cheong – Enantiomers are stereomers, too. Your solution is wrong. When you count them like you did, you should get 6: cyclopentane(1), methylcyclobutane(2), 1,1-dimethylcyclopropane(3), ethylcyclopropane(4), cis 1,2dimethylcyclopropane(5) and trans 1,2dimethylcyclopropane(6). You can't count 1,2 dime-cyclopropane three times like this! However, the trans isomer is chiral and exists as 2 enantiomers (+/-), so you got (1), (2), (3), (4), (5), +(6), -(6), meaning 7 isomers. :P
Log in to reply
@Jane Roe – I don't get what you mean. There are five isomers with cyclic structure and they are the bottom five in the attached image. There are two enantiomers/stereomers which are cis and trans 2-pentene. So the answer is 5 + 2 = 7 .
Log in to reply
@Chew-Seong Cheong – Whaaat. So you are saying the problem asks you to find cyclic structures AND the structures with stereomers? That's totally wrong. They are asking ONLY for cyclic structures, including their stereomers if they have any. 2-pentene is not cyclic, so you don't count it.
Log in to reply
@Jane Roe – I am no expert in this and I am not disputing it. I just answered the question to the best of my knowledge on the subject. That was why I have requested you to lodge a report here so that everyone will know the truth.
@Jane Roe – You can lodge a report on this.
Log in to reply
@Vignesh S – So what are you saying exactly? My answer was 7 and i counted the meso form. Why do people always reply without having read my whole comment? In my original comment i was actually saying what you yourself did, that the solution should be 4+3, not 5+2. I just feel like Chew-Seong-Cheong didn't get the question, since he also counted noncyclic isomers because they had cis/trans forms. But I get how he sees things so I'm trying to clarify, you know.
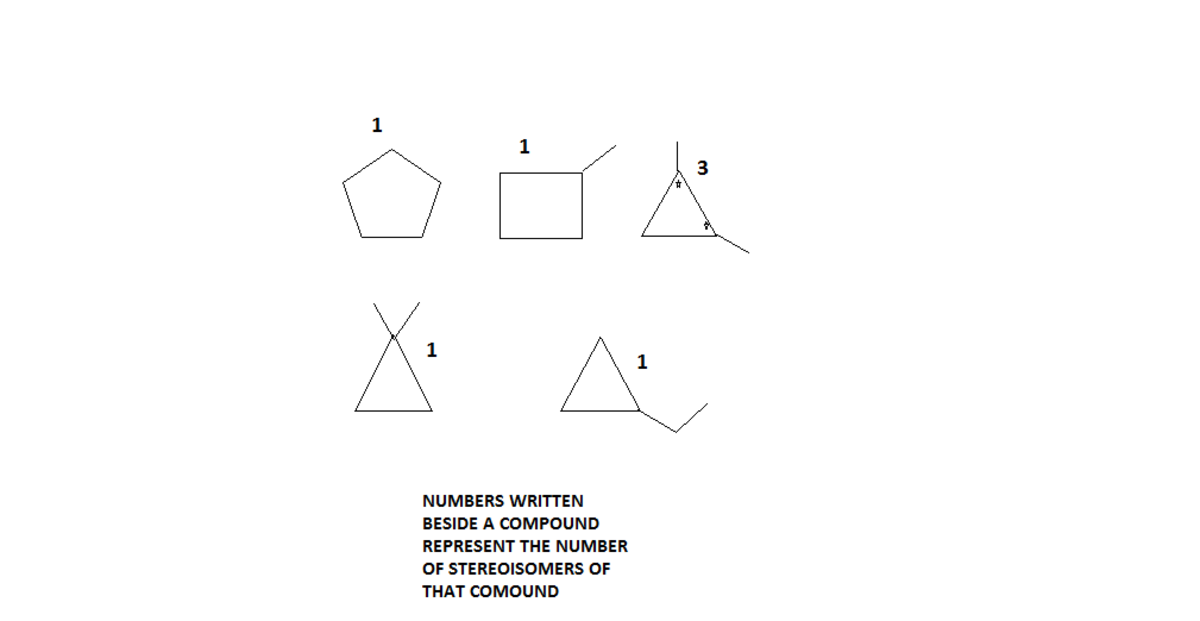
C X 5 H X 1 0 has a total of 1 1 possible isomers of which 5 have cyclic structures and cis-2-pentene and trans-2-pentene are stereomers. Therefore the answer is 5 + 2 = 7 . See image below: