A resistor swarm

First, let's mark the points on the same potential. I have done so on the picture below:

Next, we draw a simpler scheme:

On the picture above, we have several groups of parallelly connected resistors, and because all of the resistances are the same, we can present the following equivalent scheme:

On the picture above, we have 4 resistors connected in a series, so the equivalent resistor will be equal to their sum:

Switching the numerical values, we see that $Re = 15Ω$ .

We can find the equivalent resistance using the junction and the loop rule without redrawing the circuit. First let's look at the currents:

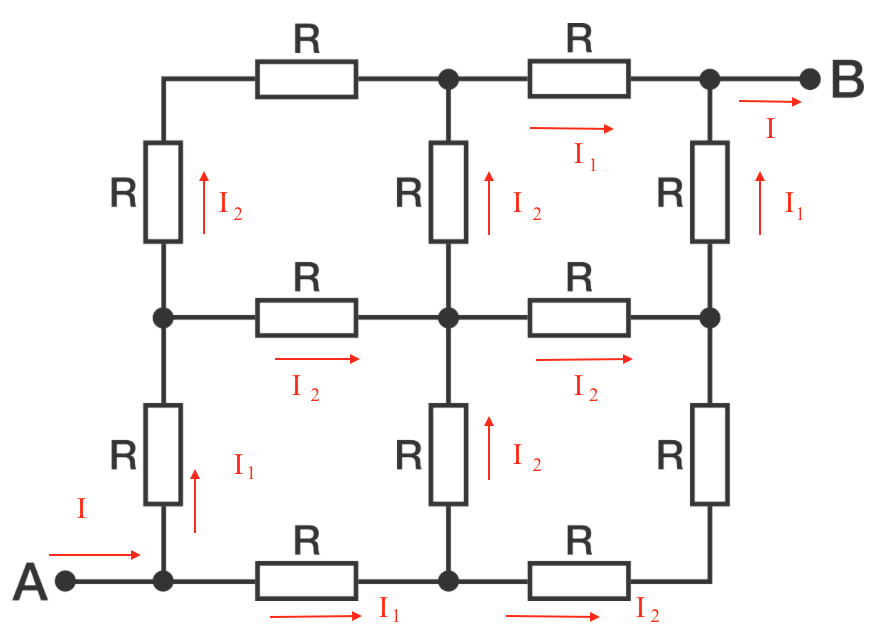
From junction rule we have: $I=2I_1$ and $I_1=2I_2$

Now we can apply a potential difference $V_{AB}$ and find a loop. We can follow this path:

So from the loop rule we have:

$V_{AB}-2I_1 R-2I_2 R=0$

Using the junction rule from above that becomes:

$V_{AB}=\frac{3}{2} I R$

The equivalent resistance between A and B is

$R_{eq}=\frac{V_{AB}}{I}=\frac{3}{2} R=15 \Omega$

First, we label the points (each line represents a resistor of $R \Omega$ ) :

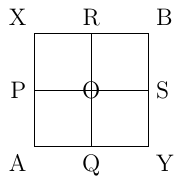
Then we redraw the grid so that it's easier to see:

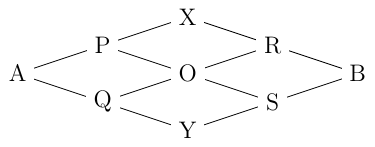
And the table below shows how many resistors there are on each column, and the resistance of each column:

So since all the columns are in series, we have:

$\frac{R}{2} + \frac{R}{4} + \frac{R}{4} + \frac{R}{2} = \frac{3R}{2} = \frac{30}{2} = 15 \Omega$

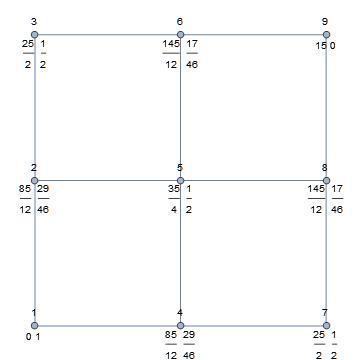
The number over the node is the assigned node number. The number below and to the left of the node is the effective resistance from node 1. The number below and to the right of the node is the voltage assuming that the input voltage at node 1 is 1 and that node 9 is at 0 voltage (grounded). These were extracted from a mesh analysis of the lattice.

As has been noted elsewhere, nodes at the same voltage (if AC, then, including phase) may be shorten together without affecting the answer of these problems.