An elegant geometric proof also exists

Using Cauchy Schwarz Inequality, $(1+4+16)(x^2+y^2+z^2)\geq(x+2y+4z)^2$ with equality only when $\frac{x}{1}=\frac{y}{2}=\frac{z}{4}$ Thus x,y,z are in G.P

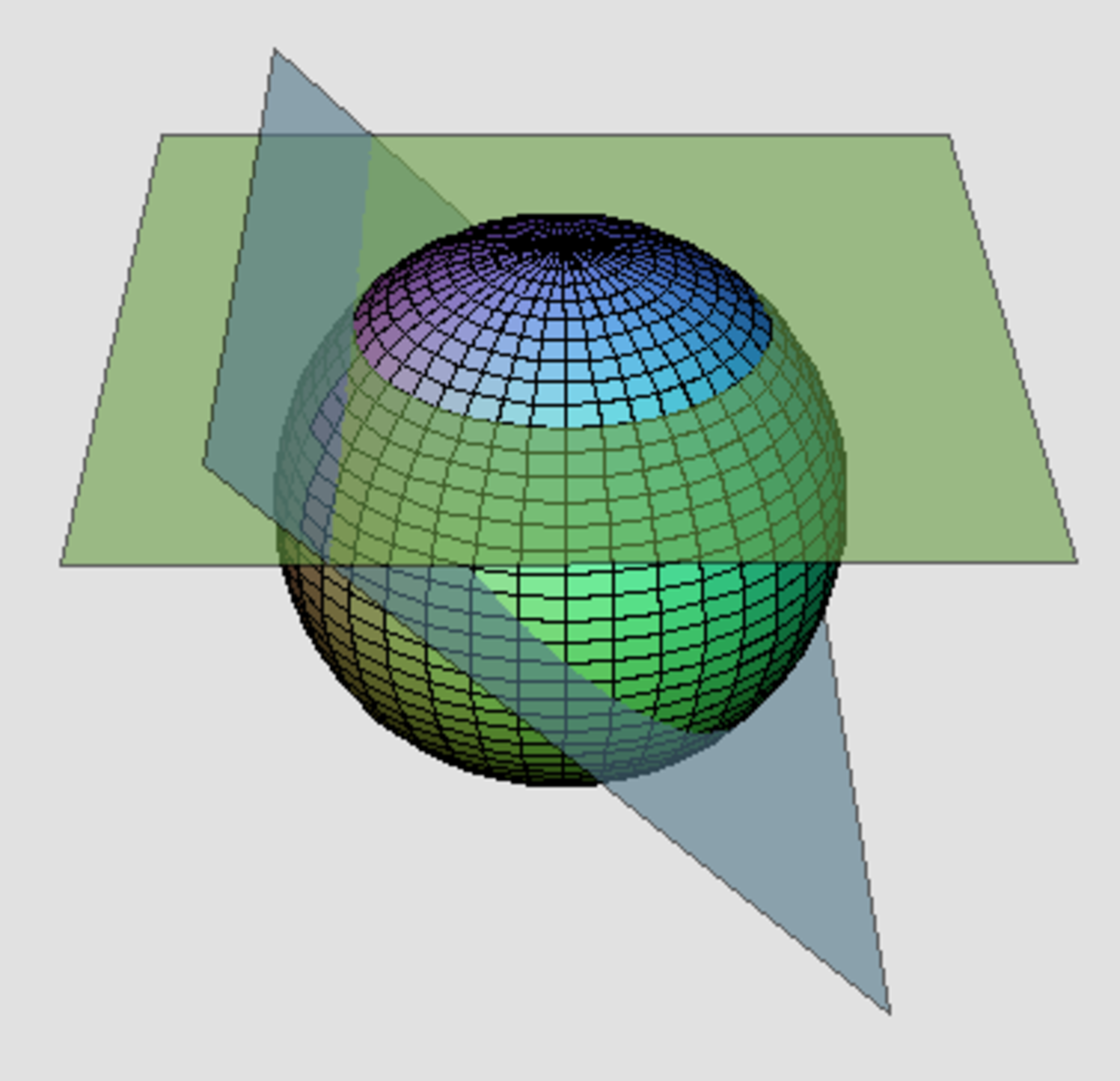
Also for the geometrical proof, consider the plane $x+2y+4z=k$ Its distance from origin is $\frac{k}{\sqrt{21}}$ .

For the foot of perpendicular from origin, If P is the foot of perpendicular from the origin on the plane , its distance from origin is given by $\sqrt{x^2+y^2+z^2}=\frac{x+2y+4z}{\sqrt{21}}$ where $k=x+2y+4z$ as P lies on the plane. square it to get the given equality.

Thus the problem reduces to finding the foot of perpendicular as we can see that the equality is achieved when $(x,y,z)$ are coordinates of FOP.

Also, another way to find the FOP is by moving in the direction of the normal from the origin. The direction ratios of the normal are (1,2,4). So if I move a distance $\frac{k}{\sqrt{21}}$ in the direction of the normal, we have the coordinate as $\frac{1}{21}k,\frac{2}{21}k,\frac{4}{21}k$ (by converting the ratios into cosines.). As we can see that this point is the FOP as it is perpendicular to the plane from the origin and satisfies the equation of the plane.

Thus $x=\frac{1}{21}k,y=\frac{2}{21}k,z=\frac{4}{21}k$ and we can see that $\frac{x}{1}=\frac{y}{2}=\frac{z}{4}$ Hence x,y,z are in G.P