Angles in a Circle

$\angle ABC$ and $\angle CDE$ adds up to $45^{\circ}$ . $\begin{array}{cc} \begin{aligned} x + 3x = & \space 45 \\ 4x = & \space 45 \end{aligned} & \implies x = \boxed{11.25} \end{array}$

Inscribed Angle Theorem (the reason why $\angle CDE$ doesn't change measurement in the animation.)

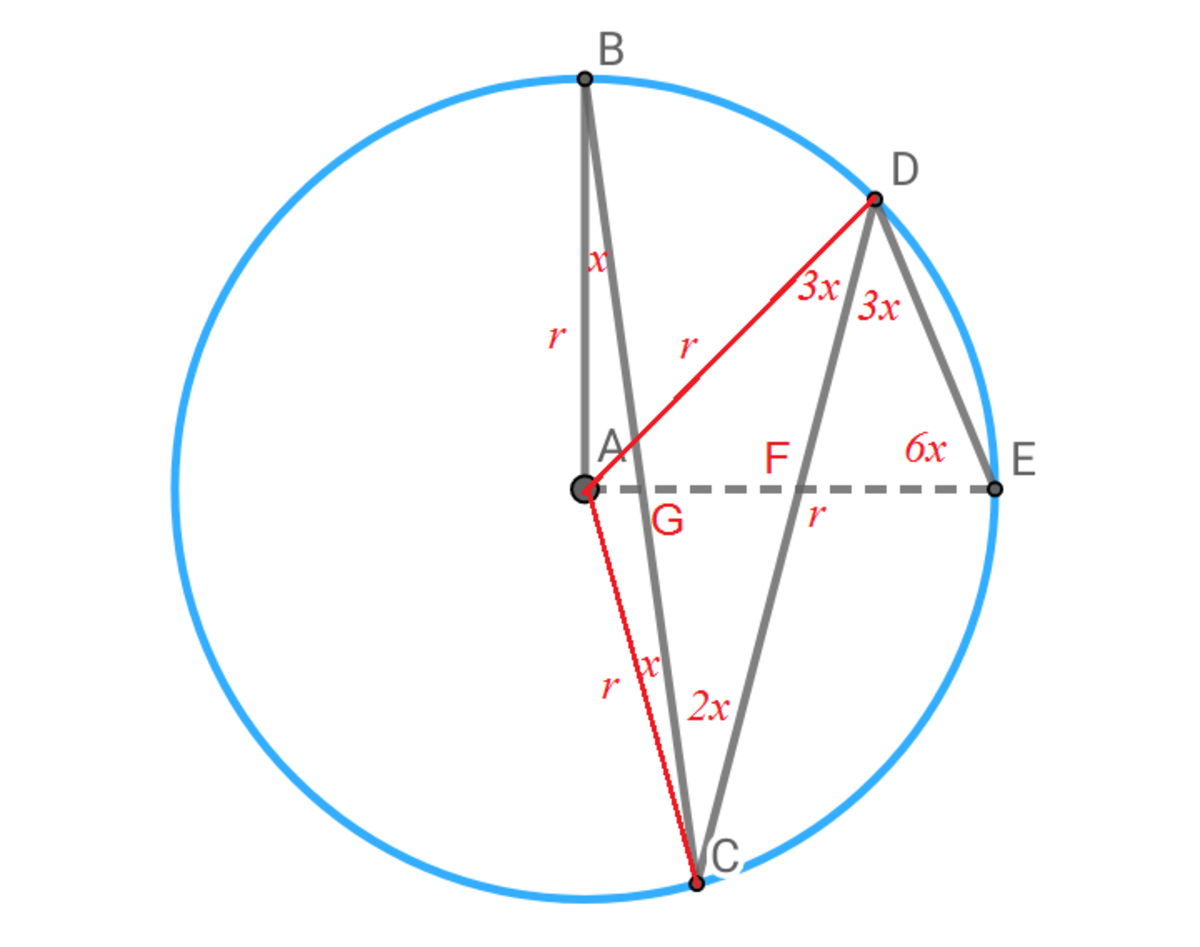
Let the radius of the circle be $r$ . Then we note that $\triangle ABC$ is isosceles and $\angle ACB = \angle ABC = x$ and $\angle ACD = 3x$ . Similarly, $\triangle ACD$ is isosceles and $\angle ADC = \angle ACD = 3x$ . Again $\triangle ADE$ is isosceles and $\angle AED = \angle ADE = 6x$ .

Let $AE$ intersect $CD$ and $BC$ at $F$ and $G$ respectively. Then $\angle CGF = \angle AGB = 180^\circ - 90^\circ - x = 90^\circ - x$ . Similarly, $\angle DFE = \angle CFG = 180^\circ - (90^\circ-x) - 2x = 90^\circ - x$ . Then we have for $\triangle DEF$ :

$\begin{aligned} \angle FDE + \angle DEF + \angle DFE & = 180^\circ \\ 3x + 6x + 90^\circ - x & = 180^\circ \\ 8x & = 90^\circ \\ \implies x & = \boxed{11.25}^\circ \end{aligned}$

Join $\overline {BE}$ . Then $\angle {ABE}=45\degree$ (since $\triangle {ABE}$ is right-angled isosceles, with the right angle at $A$ ). So, $\angle {CBE}=45\degree-x$ .

Standing on the same arc $CE$ , the angles $\angle {CBE}$ and $\angle {CDE}$ are equal. So $45\degree-x=3x\implies 4x=45\degree$

$\implies x=\dfrac {45}{4}=\boxed {11.25\degree}$ .