Another Locus Problem

Suppose for definiteness that $B > C$ . Suppose that $P$ is a point such that $\angle PBA = \angle PCA = \theta$ . If we set up a coordinate system with $C$ at the origin, $A$ at the point $(b,0)$ and $B$ at the point $(b-c\cos A,c\sin A)$ , then the coordinates of $P$ can be found by solving the simultaneous equations $y \; = \; x\tan\theta \hspace{2cm} y - c\sin A \; = \; -(x - b + c\cos A)\tan(\theta+A)$ and hence $P$ has coordinates $x \; = \; \frac{b\sin(\theta+A) - c\sin\theta}{\sin(2\theta+A)}\cos\theta \hspace{2cm} y \; = \; \frac{b\sin(\theta+A) - c\sin\theta}{\sin(2\theta+A)}\sin\theta$ and hence the locus of $P$ can be given by the polar equation $r \; = \; \frac{b\sin(\theta+A) - c\sin\theta}{\sin(2\theta+A)} \; = \; \frac{b\sin A + (b\cos A - c)\tan\theta}{\sin A(\cot\frac12A - \tan\theta)(\tan\frac12A + \tan\theta)}\sec\theta \hspace{2cm} 0 < \theta < C$ Note that if $2C < B+C = \pi - A$ , so that $C < \tfrac12\pi - \tfrac12A$ , and hence $\tan C < \cot\tfrac12A$ , so this function for $r$ is well-defined. We can now calculate the area of the region of the triangle that contains $C$ to be $\Delta_+ \; = \; \frac12\int_0^C r^2\,d\theta \; = \; \int_0^{\tan C} \frac{(b\sin A + (b \cos A - c)t)^2}{2\sin^2A(\cot\frac12A - t)^2(\tan\frac12A + t)^2}\,dt$ which can be evaluated by partial fractions, and the result $\Delta_+ \; = \; \tfrac12\Delta + \tfrac18(b^2-c^2)\sin A\ln\left(\tfrac{b+c}{b-c}\right)$ can be obtained by slogging through various triangle identities.

---

All this is not very inspiring. Let us see what is really going on. Note that the perpendicular bisector $\mathcal{L}$ of $BC$ is the locus of points $Q$ such that $\angle QCB = \angle QBC$ , and hence the desired locus of points $P$ such that $\angle PBA = \angle PCA$ is the isogonal conjugate $\mathcal{H}$ of $\mathcal{L}$ , and hence is a circumconic of the triangle $ABC$ . Since the line $\mathcal{L}$ intersects the circumcircle of $ABC$ in two places, $\mathcal{H}$ is a hyperbola. Since $\mathcal{L}$ passes through the circumcentre $O$ of the triangle $ABC$ , it follows that $\mathcal{H}$ passes through the isogonal conjugate of $O$ , namely the orthocentre $H$ of the triangle $ABC$ . This means, by Feuerbach's Conic Theorem, that $\mathcal{H}$ is a rectangular hyperbola, whose centre lies on the nine-point circle of $ABC$ . The line $\mathcal{L}$ has equation $(b^2 - c^2)ax + a^2(by - cz) \; = \; 0$ in trilinear coordinates, and hence the hyperbola $\mathcal{H}$ has equation $(b^2 - c^2)ayz + a^2bxz - a^2cxy \; = \; 0$

Using the standard formula for the centre of a circumconic, we deduce that the centre of $\mathcal{H}$ is the midpoint $M$ of $BC$ . I shall not identify the axes of the hyperbola precisely, since it is not necessary. Let us suppose that the axis system has been found, so that we have an $XY$ -coordinate system with $M$ at the origin, and that the equation of $\mathcal{H}$ is $XY = K^2$ for some $K > 0$ , and suppose that $B$ and $A$ have coordinates $(U,K^2U^{-1})$ and $(V,K^2V^{-1})$ respectively. Then the area of the small segment of the triangle is $\Delta_- \; = \; \tfrac12(V-U)(K^2U^{-1} + K^2V^{-1}) - \int_U^V K^2 X^{-1}\,dX \; = \; \tfrac12\left(\tfrac{V}{U} - \tfrac{U}{V}\right)K^2 - K^2\ln\left(\tfrac{V}{U}\right)$ Now $\left(\begin{array}{c} V \\ K^2V^{-1} \\ 0 \end{array}\right) \times \left(\begin{array}{c} U \\ K^2U^{-1} \\ 0 \end{array}\right) \; = \; \left(\begin{array}{c} 0 \\ 0 \\ K^2(\frac{V}{U} - \frac{U}{V}) \end{array}\right)$

and hence $\tfrac12K^2\left(\tfrac{V}{U} - \tfrac{U}{V}\right) \; = \; |MBA| \; = \; \tfrac12|ABC| \; = \; \tfrac12\Delta$ where $\Delta$ is the area of the triangle $ABC$ , so that $\Delta_- \; = \; \tfrac12\Delta - K^2\ln\left(\tfrac{V}{U}\right)$ Let $D$ be the angle $\angle BMA$ , and let $d = MA$ be the length of the median from $A$ . Then we have $d^2 = \tfrac12(b^2+c^2) - \tfrac14a^2$ , and hence $\cos D \; = \; \frac{d^2 + \frac14a^2 - c^2}{ad} \; = \; \frac{b^2 - c^2}{2ad}$ so that $\frac{K^4 + U^2V^2}{UV} \; = \; \overrightarrow{MA} \cdot \overrightarrow{MB} \; = \; \tfrac12ad\cos D \; = \; \tfrac14(b^2 - c^2)$ while $\frac{(V - U)^2(K^4 + U^2V^2)}{U^2V^2} \; = \; AB^2 \; = \; c^2$ and hence $\left(\sqrt{\tfrac{V}{U}} - \sqrt{\tfrac{U}{V}}\right)^2 \; = \; \frac{(V-U)^2}{UV} \; = \; \frac{AB^2}{\overrightarrow{MA} \cdot \overrightarrow{MB}} \; = \; \frac{4c^2}{b^2-c^2}$ which implies that $\frac{V}{U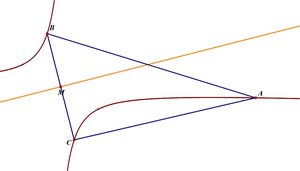} \; = \; \frac{b+c}{b-c}$ Finally we see that $\frac{V}{U} - \frac{U}{V} \; = \; \frac{4bc}{b^2-c^2}$ and hence $K^2 \; = \; \frac{b^2-c^2}{4bc}\Delta \; =\; \tfrac18(b^2 - c^2)\sin A$ so that the formula for $\Delta_-$ becomes $\Delta_- \; = \; \tfrac12\Delta - \tfrac18(b^2 - c^2)\sin A \ln\left(\tfrac{b+c}{b-c}\right)$ What happens when $B < C$ ? In this case the hyperbola is oriented differently, and the portion of the hyperbola that is internal to the triangle is a curve from $A$ to $C$ , instead of from $A$ to $B$ (see the final diagram). If $B=C$ the hyperbola $\mathcal{H}$ collapses to the perpendicular bisector $\mathcal{L}$ of $BC$ , dividing the triangle into two triangular regions of equal area $\tfrac12\Delta$ .

---

To answer the problem, we have $a=5\,,\,b=4\,,\,c=3\,,\,A = 90^\circ$ , so that $\Delta_- = 3 - \tfrac78\ln7$ , making the answer $3 + 7 + 8 = \boxed{18}$ .

Let $A(0,0)$ , $B(3,0)$ , $C(0,4)$ , $P(x,y)$ , and $\angle PBA = \angle PCA = \theta$ . Then

$\begin{aligned} \frac y{3-x} & = \tan \theta = \frac x{4-y} \\ 4y - y^2 & = 3x - x^2 \\ x^2 - y^2 - 3x + 4 y & = 0 \\ \left(x - \frac 32 \right)^2 - (y-2)^2 & = \frac 94 - 4 = - \frac 74 \\ \implies y & = 2 - \sqrt{\left(x - \frac 32 \right)^2+\frac 74} \end{aligned}$

Therefore, the small area is

$\begin{aligned} A & = \int_0^3 y \ dx = \int_0^3 \left(2 - \sqrt{\left(x - \frac 32 \right)^2+\frac 74}\right) dx & \small \blue{\text{Let }\sinh t = \frac {2x-3}{\sqrt 7} \implies \cosh t\ dt = \frac 2{\sqrt 7} dx} \\ & = 2 x \ \bigg|_0^3 - \int_{\sinh^{-1} - \frac 3{\sqrt 7}}^{\sinh^{-1} \frac 3{\sqrt 7}} \frac 74 \cosh^2 t \ dt \\ & = 6 - \frac 7 2 \int_0^{\sinh^{-1} \frac 3{\sqrt 7}} \cosh^2 t \ dt \\ & = 6 - \frac 7 4 \int_0^{\sinh^{-1} \frac 3{\sqrt 7}} (\cosh 2t + 1) \ dt \\ & = 6 - \frac 74 \left[\frac {\sinh 2 t}2 + t \right]_0^{\sinh^{-1} \frac 3{\sqrt 7}} \\ & = 6 - 3 - \frac 74 \sinh^{-1} \frac 3{\sqrt 7} \\ & = 3 - \frac 78 \ln 7 \end{aligned}$

Therefore, $l + m + n = 3 + 7 + 8 = \boxed{18}$ .

Take $A$ as the origin of coordinates, $\overline {AC}$ along the $x$ -axis, $\overline {AB}$ along the $y$ -axis. Then the locus of $P$ is

$x^2-y^2-4x+3y=0$

$\implies y=\dfrac {3+\sqrt {4x^2-16x+9}}{2}$

The smaller area is

$\displaystyle \int_0^4 \dfrac {3+\sqrt {4x^2-16x+9}}{2}dx$

$=3-\dfrac {7\ln 7}{8}$

So $l=3,m=7,n=8$ and $l+m+n=\boxed {18}$ .