Applying Pythagoras
If the square top of a table has a diagonal of 6 feet, find the area of the table top (in square feet).
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
2 solutions
.how did you get 2 x 2 = 3 6 . please,give clear explanation.
Log in to reply
I have edited my answer.
Log in to reply
thanks.just give me one answer-how can i learn hard latex techniques to post calculus,tough looking problems?
Log in to reply
@Mohammad Khaza – In brilliant, they have solutions writing techniques. When writing a solution, you will see it. Just follow the rules and you would be able to write with latex. I hope that was helpful. Also, if you tag any of the staff, probably they have more advice on that issue.
@Mohammad Khaza – Do you want 'Limits'?
Log in to reply
@Munem Shahriar – sure. if you know ,please...
Log in to reply
@Mohammad Khaza – Try the code,
\large \lim_{x\to\infty}
It can be different when used
Put the code inside of \ [ \ ]
Appears as x → ∞ lim
Put the code inside of \ ( \ )
Appears as lim x → ∞
Tell me if this helps. @Mohammad Khaza
Log in to reply
@Munem Shahriar – oh! THAT IS WORKING. GREAT!soon i will post and solve calculus problems
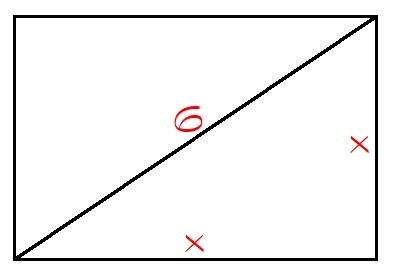 6
2
=
x
2
+
x
2
6
2
=
x
2
+
x
2
3 6 = 2 x 2
x 2 = 1 8
fantastically solved!!
Since the diagonal is 6 . By applying Pythagoreas theorem, we have x 2 + x 2 = 6 2 = 3 6 ⟹ 2 x 2 = 3 6 , where x is the side of the square (table top) ⟹ x 2 = 1 8 . Therefore, the area of the square top is 1 8 .