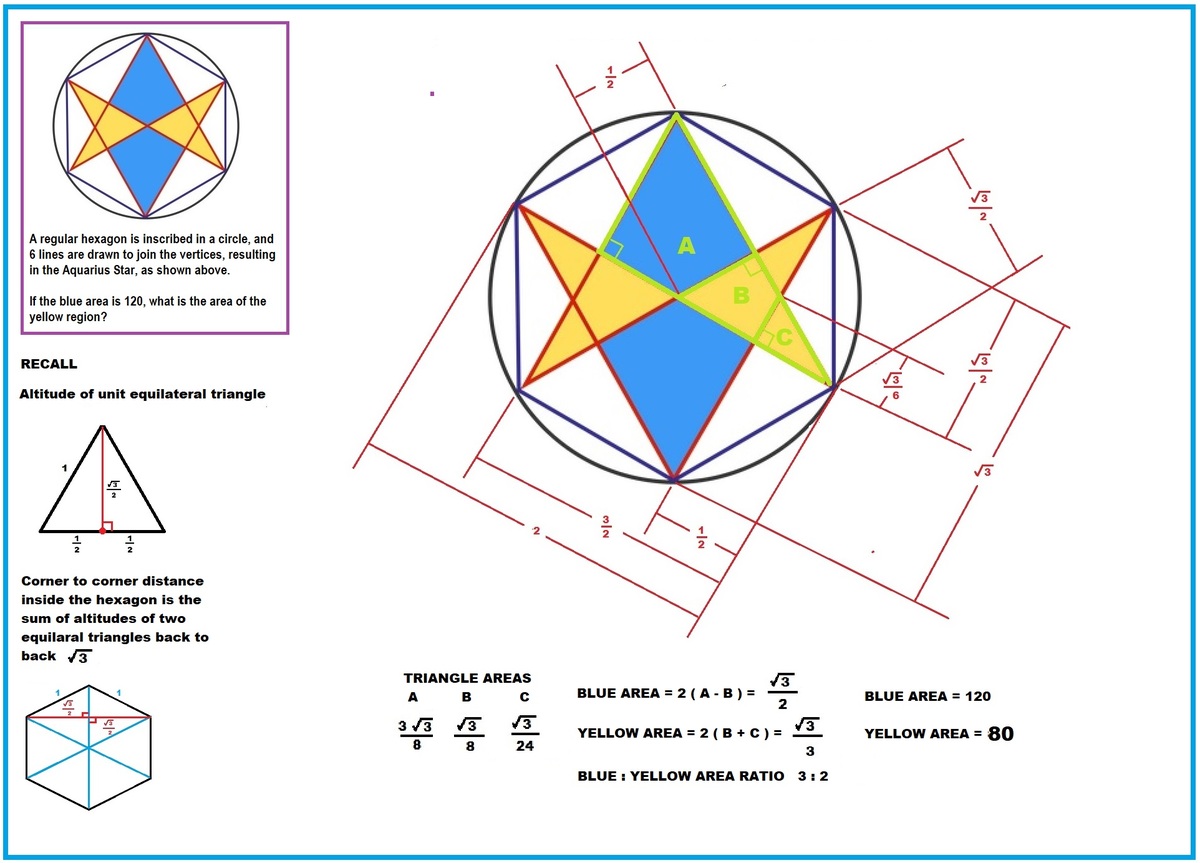
Aquarius Star

Count the triangles. There are 12 blue triangles, and 8 yellow triangles. Therefore, if blue area = 120, the yellow area = 80.

According to the image above, considering triangle $ACE$ , the center $O$ is the centroid of the triangle, making the triangles $AOE$ , $EOC$ , $COA$ congruent.

And since the inscribed hexagon has each side length equal to the radius, they are $3$ rhombhi combining with common vertex $O$ . Thus, the red lines crossing $AE$ & $AC$ are the bisectors for the triangles' bases.

Thereby, each blue kite has the same area as triangle $AOE$ , equal to $\dfrac{1}{3}$ of triangle $ACE$ .

With similarity, each blue kite has an equal area to triangle $FOE$ or $BOC$ .

And similarly, the red and green lines also intersect each other at the centroids of those $2$ triangles.

Thus, the yellow area is $\dfrac{2}{3}$ of those triangles, which equals to the blue area.

Finally, the yellow area = $\dfrac{2}{3}\times 120 = 80$ .

1. $EI = IO$ since $FD$ is perpendicular to $BE$ , thus $FI$ is the altitude of equilateral triangle $OFE$ .
2. $[ GHO] = [HIO]$ by symmetry and $= [EIO]$ since $EI = IO$ and $= [FGH]$ by symmetry
3. Thus, one yellow quadrilateral is equal in area to 4 small triangles $[GHO]$ .
4. $AG = GE$ since $CF$ is perpendicular to $AE$ thus $CF$ is the altitude of equilateral triangle $ACE$ .
5. $[AGO] = [EGO ]$ since $AG = GE$ , which is equal in area to 3 small triangles.
6. Hence, the blue quadrilateral is equal in area to 6 small triangles.
7. Thus, yellow : blue $= 4 : 6$ . So the yellow are is $120 \times \frac{4}{6} = 80$ .

The hard way, as usual!