Area problem

In each case, the area shaded is half of the area of the square. Since the red regions added are the same each time, the purple and pink areas must both have the same area of $\boxed{120}$ .

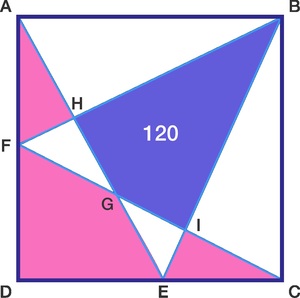
Let the area of the square $[ABCD] = A_{square}$ , the blue area be $A_{\color{#69047E}purple}$ and the sum of the pink areas be $A_{\color{#D61F06}pink}$ . Note that:

$\begin{cases} A_{\color{#69047E}purple} + [FGH] + [BCI] & = [BCF] & = \frac 12 A_{square} \\ A_{\color{#D61F06}pink} + [FGH] + [BCI] & = [ADE] + [BCE] & = \frac 12 A_{square} \end{cases} \implies A_{\color{#D61F06}pink} = A_{\color{#69047E}purple} = \boxed{120}$

This is a slight "cheating" answer, but since it is implied that a unique answer exists, the positions of points F on AD and E on DC shouldn't matter.

Slide point F to A and slide point E to C. Quadrilateral BHGI is now a right triangle, and half of the square. The pink areas form the other half of the square. So, under the assumption that there is a unique answer, the areas are the same.

Starting from $\Delta AFH$ and going in a clockwise rotation, designate the areas of the distinct regions as $a,b,c,d,e,f,g,h$ . (In this designation, then, the areas of the red regions will be $a, e, g$ , the area of the blue region will be $c$ and the areas of the white regions $b, d, f, h$ .)

Now as $|AF| + |FD|$ is equal to the side length of the square, we know that $|\Delta ABF| + |\Delta FCD|$ will equal half the area of the square. But so too will $|\Delta ABE|$ . Therefore

$|\Delta ABF| + |\Delta FCD| = |\Delta ABE| \Longrightarrow a + b + e + f + g = b + c + f \Longrightarrow a + e + g = c$ .

But this just means that the sum of the areas of the 3 red regions is equal to that of the blue region, and so the desired sum is $\boxed{120}$ .

Area of the non-pink region equals |AEB|+|CFB| - 120 which equals |ABCD| - 120 (since |AEB| + |CFB| = |ABCD|).

Area of the pink region = |ABCD| - area of non-pink region = |ABCD| - (|ABCD| - 120) = 120

The answer is 120.
Let the areas of each part are as shown on the picture: 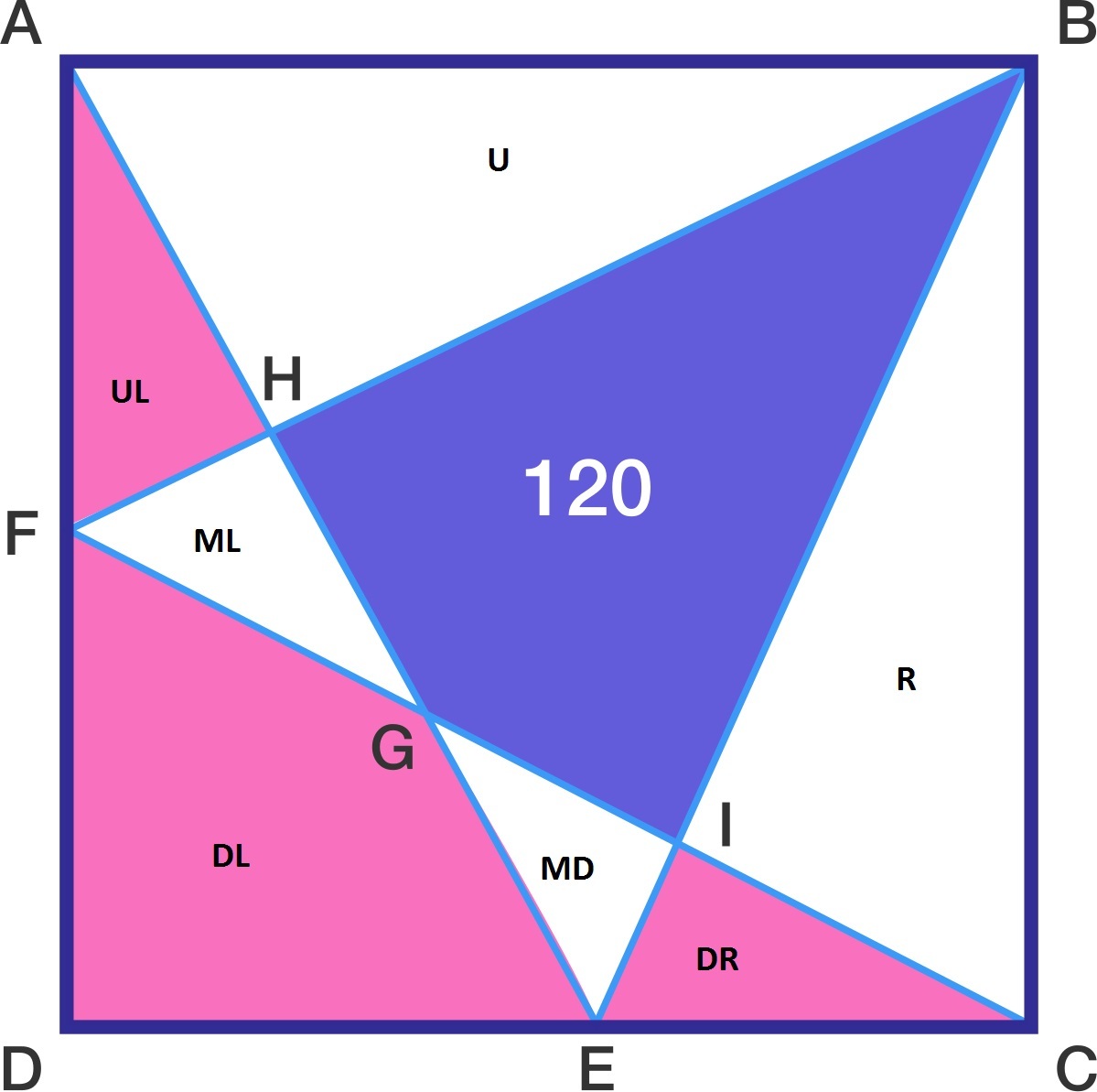We can write and solve two equations for PA(PinkArea) = UL + DL + DR
(1) [ABF]+[FCD]=[BCF] for any position of F on AD
(2) [DAE]+[EBC]=[ABE] for any position of E on DC

           ML+120+R = UL+U+DL+MD+DR = PA+U+MD   
           U+120+MD = UL+ML+DL+R+DR = PA+R+ML

        ML+120+R = PA+U+MD
     +  U+120+MD = PA+R+ML 
  ----------------------------------      
   ML+MD+U+R+240 = ML+MD+U+R+2PA -> 2PA=240 -> PA=120

I simply cheated with this one. Because I am lazy I simply made points F and G coincident with D and C the problem is then trivial. An engineers approach! ,

$[AHB] = [FGED] + [FHG]$

Half Square:

$= [AHB] + [FHG] + 120$

$= [AHB] + [FHG] + [AHF] + [ICE] + [FGED]$

Then:

$[AHB] + [FHG] + 120 = [AHB] + [FHG] + [AHF] + [ICE] + [FGED]$

$120 = [AHF] + [FGED] + [ICE]$