Asap

Don't use calculus, it will get messy.

Hint: Fermat's principle. If light passes through 2 points, it chooses the path that does so in the shortest time.

Additional hints: $\frac{v_{\text{swim}}}{v_{\text{run}}} = \frac{3}{5} = \frac{\sin \theta}{\sin 90^{\circ}} \\ \ \\ 8.75 + 24.75 + 22.5 = 56$

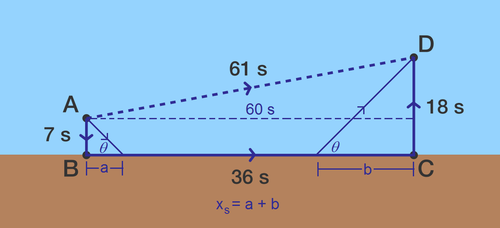
Since Agatha's swimming rate is constant. The distance is directly proportional to time. Therefore, the time taken for a distance of $BC$ is given by $t_s = \sqrt{61^2- (18-7)^2} = 60 \text{ s}$ . If the Agatha's swimming speed and running speed are $v_s$ and $v_r$ respectively, then $v_s = \frac {36}{60} v_r = \frac 35 v_r$ .

By Fermat's principle, the shortest path of travel is that taken by light. In this case the beach $BC$ acts like a mirror the swimming path is reflected at the beach with the same angle of incidence and angel of reflection. The total distance swam is then $d_s = \sqrt{x_s^2+y_s^2}$ , where $x_s$ and $y_s$ are the distances parallel and perpendicular to $BC$ respectively. Let $v_r =1$ . Then the time taken by Agatha to run $x_s$ distance is $x_s$ seconds. And her swimming time is $\frac 53 x_s$ seconds. We note that she takes $7+18 = 25 \text{ s}$ to swimming $y_s$ . The time taken running on the beach is $36-x_s$ . Then the total time taken by her to travel from $A$ to $D$ is given by:

$\begin{aligned} t & = \underbrace{36-x_s}_{\text{Running}} + \underbrace{\sqrt{\left(\frac 53x_s\right)^2+25^2}}_{\text{Swimming}} = 36-x_s + \frac 53 \sqrt{x_s^2+225} \end{aligned}$

To find $t_{\min}$ , $\dfrac {dt}{dx_s} = - 1 + \dfrac {5x_s}{3\sqrt{x_s^2+225}}$ . We note that $\dfrac {dt}{dx_s}=0$ , when $x_s =11.25$ and $\dfrac {d^2t}{dx_s^2} > 0$ . Therefore, $t_{\min} = 36-11.25 + \dfrac 53 \sqrt{11.25^2+225}=\boxed{56}$ .

Assume that her speed in sea is $v=1ms^{-1}$ , then all we get is $AB=7m, CD=18m, AD=61m,$ and also by the Pythagorean theorem, we have $BC=\sqrt{AD^2-(CD-AB)^2}=\sqrt{61^2-11^2}=60(m)$ , so her speed on the land is $v'=\dfrac{60}{36}=\dfrac53ms^{-1}$ .

Thus, assume she is swimming straightly from $A$ to a point $X$ lies between $BC$ , and let $BX=x$ . Then she running from $X$ to $Y$ which is a point between $BC$ but nearer to $C$ , then also let $CY=y$ . After she reached point $Y$ , she then changes her direction to point $D$ and swimming straight until she gets to her son.

This all-time process cost a time $T$ , and it can be expressed as $T=\dfrac{\sqrt{x^2+49}m}{1ms^{-1}}+\dfrac{(60-x-y)m}{\dfrac53ms^{-1}}+\dfrac{\sqrt{y^2+324}m}{1ms^{-1}}$ which then simplify to $T=\sqrt{x^2+49}+\sqrt{y^2+324}+36-\dfrac35x-\dfrac35y$ Because the variable $x$ and $y$ can change independently. So we can let $S_1=\sqrt{x^2+49}-\dfrac35x$ , and $S_2=\sqrt{y^2+324}-\dfrac35y$ then $T=S_1+S_2+36$ .

This is a small research on this kind of minimum,

So the minimum of $S_1$ will be $7\sqrt{1-\dfrac9{25}}=\dfrac{28}5$ and the minimum of $S_2$ will be $18\sqrt{1-\dfrac9{25}}=\dfrac{72}5$ .

Finally, we have $\begin{aligned}T_{min}&=36+S_{1min}+S_{2min}\\&=36+\dfrac{28}5+\dfrac{72}5\\&=\boxed{56(s)}\end{aligned}$

We'll start by finding the length of the shoreline with respect to the rates. Assume Agatha swims at a rate of 1 foot per second. Then by the Pythagorean theorem, the shoreline is $60$ feet long, and Agatha runs at a rate $\frac{3}{5}$ of her swimming speed.

I claim that given a distance of $a$ from the shoreline, a running time of $r$ with respect to swimming time, and a sufficiently long shoreline, the distance required to swim with respect to the shoreline is always $\frac{ra}{\sqrt{1-r^2}}$ Proof Let the distance swam with respect to the shoreline be $x$ . Then by Pythagorean Theorem, the distance swam is $\sqrt{a^2+x^2}$ . Let the shoreline then be of length $b$ . Then the distance ran is $b-x$ . Weighting these distances with their rates gives $t=\sqrt{a^2+x^2}+r(b-x)$ $\frac{dt}{dx}=\frac{x}{\sqrt{a^2+x^2}}-r=0$ $x^2=r^2(a^2+x^2)$ $x^2(1-r^2)=r^2a^2$ $x=\frac{ra}{\sqrt{1-r^2}}$ Which completes the proof.

Now, plugging in our values then gives the horizontal distances at the beginning and end, which turns out to be $x_a=\frac{21}{4}, x_b = \frac{27}{2}$ And so the optimal time is $t=\sqrt{49+\left(\frac{21}{4}\right)^2}+\frac{3}{5}\cdot \left(60-\frac{21}{4}-\frac{27}{2}\right) + \sqrt{324+\left(\frac{27}{2}\right)^2}=\frac{35}{4}+\frac{99}{4}+\frac{90}{4}=\frac{224}{4}=\boxed{56}$

If the angles she makes with the line perpendicular to the shore when she's swimming are P and Q, and her swimming speed is x (so her running speed is 5x/3), then the time taken = (7x secP + 18x secQ)/x + (36 - 7x tanP - 18x tanQ)/(5x/3) = 7secP - 4.2tanP + 18secQ - 10.8tanQ + 36. Differentiate w.r.t. P gives 7secP.tanP - 4.2 sec^2 P = 0 when sin P = 3/5. Similarly sin Q = 3/5, so total time is (7 + 18).5/4 - (4.2 + 10.8).3/4 + 36 = 56. Not very elegant, but you could hardly call it messy.

One could easily find that $\displaystyle \frac{v_{water}}{v_{beach}} = \frac{3}{5}$ . Let $x$ be amount of time in seconds spent on the beach. We can manipulate the diagram by hiding the path of the $x$ seconds on the beach as displayed below.

The shortest path on the water is the diagonals of the horizontal distance $\left(36-x\right)v_{beach}$ (we and the vertical distance $25v_{water}$ in the second diagram, which means the distance traveled in water is $\sqrt{\left(\left(36-x\right)v_{beach}\right)^{2} + \left(25v_{water}\right)^{2}}$ .

The function of time is:

$\displaystyle t(x) = t_{water} + t_{beach}$

$\displaystyle = \frac{s_{water}}{v_{water}} + x$

$\displaystyle = \frac{\sqrt{\left(\left(36-x\right)v_{beach}\right)^{2} + \left(25v_{water}\right)^{2}}}{v_{water}} + x$

$\displaystyle = \sqrt{\left(\left(36-x\right)\frac{v_{beach}}{v_{water}}\right)^{2} + \left(25\right)^{2}} + x$

$\displaystyle = \sqrt{\left(\frac{5}{3} \left(36-x\right)\right)^{2} + \left(25\right)^{2}} + x$

$\displaystyle = \sqrt{\left(60-\frac{5}{3}x\right)^{2} + 625} + x$

Solving $t'(x) = 0$

$\displaystyle \frac{-\frac{5}{3}\left(60-\frac{5}{3}x\right)}{\sqrt{\left(60-\frac{5}{3}x\right)^{2} + 625}} + 1 = 0$

gives $\displaystyle x= \frac{99}{4}$ which is a local minima of $t(x)$

Therefore, the minimum gives $\displaystyle t\left(\frac{99}{4}\right) = \boxed{56 \text{ seconds}}$

After you realise that the swimming speed is 3/5 that of the running speed, it's quite easy to prove that there exists and then find the value of an ideal angle of travel. Calculate how much time it takes to swim to and from the shore using this angle, and then add in the time it takes to run between the two shoreline points.