Ball, Don't Fall!
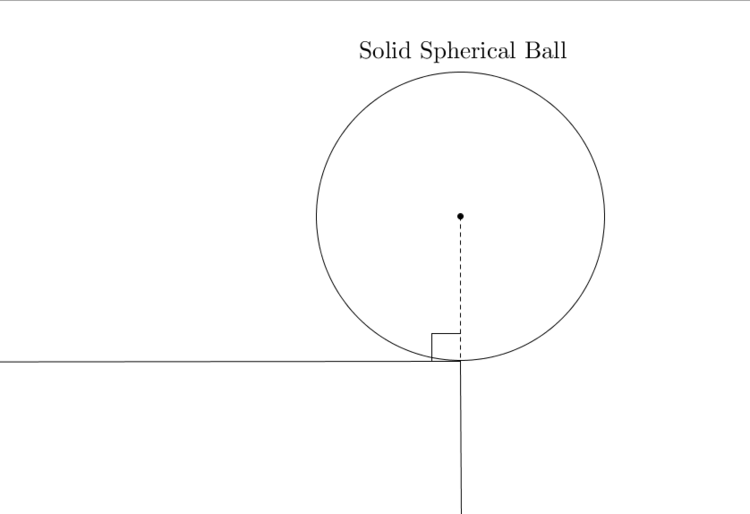 A solid spherical ball is placed carefully on the edge of a table in the position shown in the figure. The coefficient of static friction between the ball and the edge of the table is
0
.
5
. It is then given a very slight push. It begins to fall off the table.
A solid spherical ball is placed carefully on the edge of a table in the position shown in the figure. The coefficient of static friction between the ball and the edge of the table is
0
.
5
. It is then given a very slight push. It begins to fall off the table.
Find the angle (in degrees) turned by the ball before it slips.
Details and Assumptions
- Find the angle with the vertical.
The answer is 41.83.
This section requires Javascript.
You are seeing this because something didn't load right. We suggest you, (a) try
refreshing the page, (b) enabling javascript if it is disabled on your browser and,
finally, (c)
loading the
non-javascript version of this page
. We're sorry about the hassle.
3 solutions
Exactly what I did ! But @Ronak Agarwal @Mvs Saketh @Pratik Shastri can you please explain its Motion in the duration of θ = 4 1 . 8 3 to θ = 5 6 . 9 6 degrees ?? Thanks!
Log in to reply
@DEEPANSHU GUPTA The situation is more complex, since the ball has begun to slip, now friction actually does work, and thus the balls rate of gaining energy decreases,, also it is no longer pure rolling about the cliff ,, thus we have to consider the situation as a superposition of translation and rotation about com now, and yes it wont lose contact at the same angle now, since now even friction does work, it seems mathematically complex , but i will tery to analyse it and report back
Log in to reply
Yes , And I wonder that how we can proceed further if we want to some further analysis ! I mean that As @Ronak Agarwal Posted his question that Find The range of this cylinder if it is fall from the clif and performing The Projectile ! Since We can't apply energy conservation etc..!! I tried this at my own but situation becomes more and more complex ! So What you r thinking about it ? ? @Mvs Saketh
Log in to reply
@Deepanshu Gupta – When rolling ceases (pure) bro, then the centre is no longer rotating about the cliff and hence we must take coriolis force into account as well (if we wish to continue keeping origin at the cliff tip) , so indeed its complicated and indeed energy is not conserved ,. Whilst the good thing is friction becomes constant but the bad things out weigh it , so i suppose u will get a really complicated differential equation
Comprehensive Solution. Up voted!
@ronak aggarwal- it seems you have done the last part without calculator (because you expressed it as a fraction first) is it true? and if so how could you solve it without calculator?
Log in to reply
Simplifying the equation for θ we would we getting :
1 7 c o s ( θ ) − 4 s i n ( θ ) = 1 0
It can also we written as :
3 0 5 c o s ( θ + ϕ ) = 1 0
where t a n ( ϕ ) = 1 7 4
Taking inverse :
θ = c o s − 1 ( 3 0 5 1 0 ) − t a n − 1 ( 1 7 4 )
Ofcourse after this I used a calculator for finding θ
Log in to reply
oh that is much better than squaring and adding extraneous roots! +1
Why you use moment of inertia about the tip not about the center of mass @Ronak Agarwal @Deepanshu Gupta @Mvs Saketh @Pratik Shastri .
 The situation
The situation
figure is in rotating frame
Slipping begins when the acceleration of the point of contact of the ball with the edge is no longer at rest,,
That is the superposition of rotational and translational accelerations about the com for the contact point is no longer 0
or mathematically a ≥ α R
now in the limiting case,, friction is just able to support pure rolling about edge or it is maximum,
Also we know that only friction provides the torque about com,,
and that balls centre of mass only moves perpendicular to the direction of normal reaction,, and the only forces along it are the component of gravity along it (sin component) and friction acting opposite to it giving the ball acceleration along it.
thus we have
g s i n θ − m f = a f R = I α f = μ N = μ ( m g c o s θ − m w 2 r )
and finally through energy conservation we have
2 m g R ( 1 − c o s θ ) = I a b o u t e d g e w 2
Solving all these we get two values for the angle as
θ = 4 1 . 8 3 ( a p p r o x ) a n d θ = 6 8 . 3 4 4
the latter is rejected obviously ( can be shown from the fact that had the ball not slipped at 41.83 degrees it would have lost contact at somewhere near 53 degrees, and so theres no way it can slip at an angle greater than that)
thus we get answer
Nice diagram :D
Nice!+1 :)
Mvs Saketh can u plz can tell why mw^2r Acting in the dir. Of normal?
The following diagram show's all the forces being applied on the sphere when it is making an angle θ with the horizontal.
I will be taking direction along the normal as x-axis and direction anti parellel to friction as y-axis.
Till the moment the ball starts slipping we can assume the whole sphere to be rotating about the tip.
First thing we would be calculating is the angular velocity of the ball, it can be easy calculated by using work energy theorom.
Work done by gravity = Change in Kinetic energy.
m g R ( 1 − c o s ( θ ) ) = 2 1 I t i p ω 2
I t i p = I c o m + m R 2 = 5 7 m R 2
We get the expression of ω as :
ω = 7 R 1 0 g ( 1 − c o s ( θ ) )
Now we will find angular acceleration of the ball :
m g s i n ( θ ) R = 5 7 m R 2 α
⇒ α = 7 R 5 g s i n ( θ )
⇒ a y = α R = 7 5 g s i n ( θ )
There is no acceleration of ball in x-axis. Hence writing equation of equilibrium in x-direction we get :
m g c o s ( θ ) = N + m ω 2 R
Putting the expression of ω we get :
N = 7 m g ( 1 7 c o s ( θ ) − 1 0 )
Also using force equation in y direction we get :
m g s i n ( θ ) − f = m a y
Putting the expression of a y we get :
f = 7 2 m g s i n ( θ )
At the time of slipping we have :
η N = f
Where η is the co-efficient of friciton :
Putting the expression of N , f and η = 0 . 5 we get :
1 4 m g ( 1 7 c o s ( θ ) − 1 0 ) = 7 2 m g s i n ( θ )
Solving θ comes out to be :
θ = t a n − 1 ( 1 0 2 0 5 ) − t a n − 1 ( 1 7 4 )